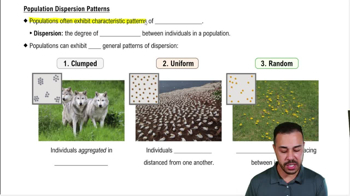
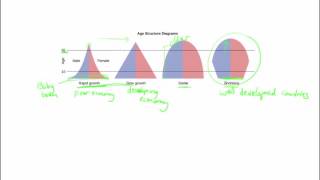
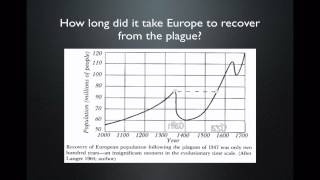
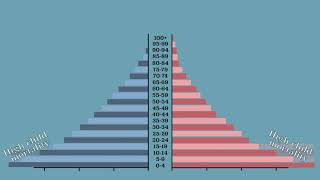
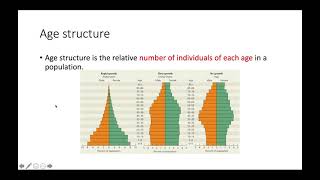
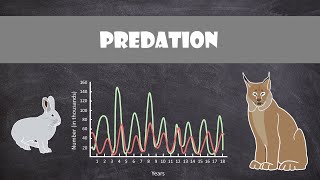
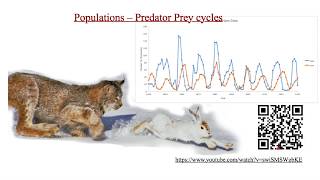
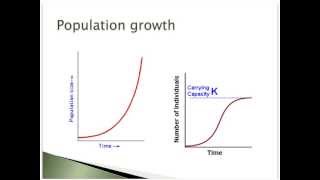
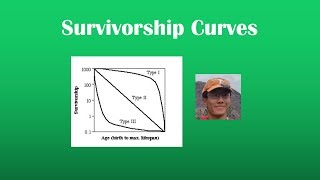
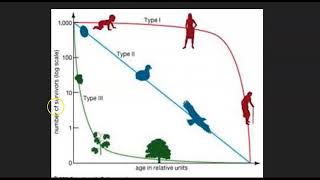
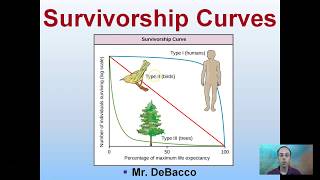
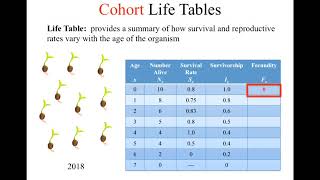
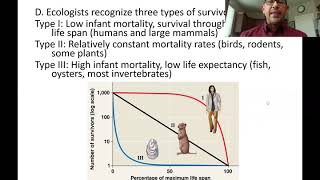
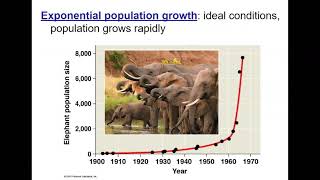
Per capita just means per individual in a population. So the per capita birth rate is the number of offspring produced by the average member of the population per unit time, because it's a rate, remember? So the mortality rate is going to be the average number of deaths per unit time in a population. We'll look at these two factors to see how population sizes fluctuate. Now, if the birth rate is equal to the death rate, your population size won't change. However, if your birth rate is higher than your death rate, your population is going to increase in size. Whereas if your death rate is higher than your birth rate, your population is going to decrease in size. Now the difference between the birth rate and the death rate, or the mortality rate, I should say, is the per capita rate of increase, or r. Now when r=0, like we saw at that midpoint, you have 0 population growth. And again, that's because the birth and mortality rates are equal. When you have an r that's greater than 1, meaning your birth rate exceeds your mortality rate, you get exponential population growth. And this is a J-shaped curve, you can see it right down here. This is what exponential growth looks like. And basically, this is a density-independent type of growth, meaning that it does not depend on the number of individuals in the population.

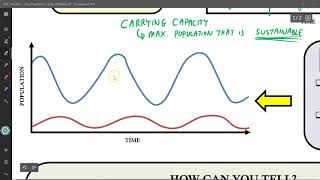
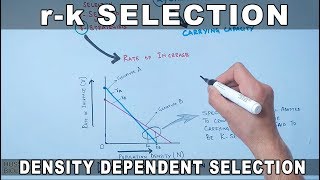
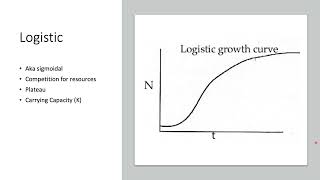
And if the birth rate is as high as possible and the death rate is as low as possible, you can have an intrinsic rate of increase or r_max, the maximum rate of increase. So exponential growth does occur in nature sometimes, but it can't occur indefinitely, basically. Resources are finite, space is finite, you know, everything is finite in nature, and that's why even though you might have a period of exponential growth for a population, eventually that's going to burn itself out. And what we will often see is logistic population growth. So basically, there's an upper limit to the population size that an area can sustain. And this limit is due to the number of resources available, how much space is available, and because individuals are going to compete for those resources and that space, and there's going to be, in fact, other species competing for those resources in that space. Additionally, and this doesn't always apply, but the amount of waste produced by the individuals can lead to limits on population size. For example, if you take a look at yeast, which produce alcohol, they produce ethanol, which is waste to them as a byproduct of their metabolism, and eventually that waste can kill them. So, it's not always relevant, but it can be relevant. So carrying capacity is just this maximum population size. It's the limit on the population that the area can sustain, and we represent it with the letter k.

So here you can see that in this particular chart the carrying capacity is reached due to a limit on the food supply. However, it could be other factors as well, in actual real-life scenarios. Now here we can see the characteristic S-shaped curve of the logistic growth curve. And a couple things that I want you to take note of is that the population size will increase and r will go up, and then r will go down. Actually, in the second part of the curve r is approaching 0. And one thing that I want you to take note of is that r is going to be at its greatest when N=1/2k. So if this is our carrying capacity, the halfway marker there is going to represent the greatest r value. Alright, let's turn the page.