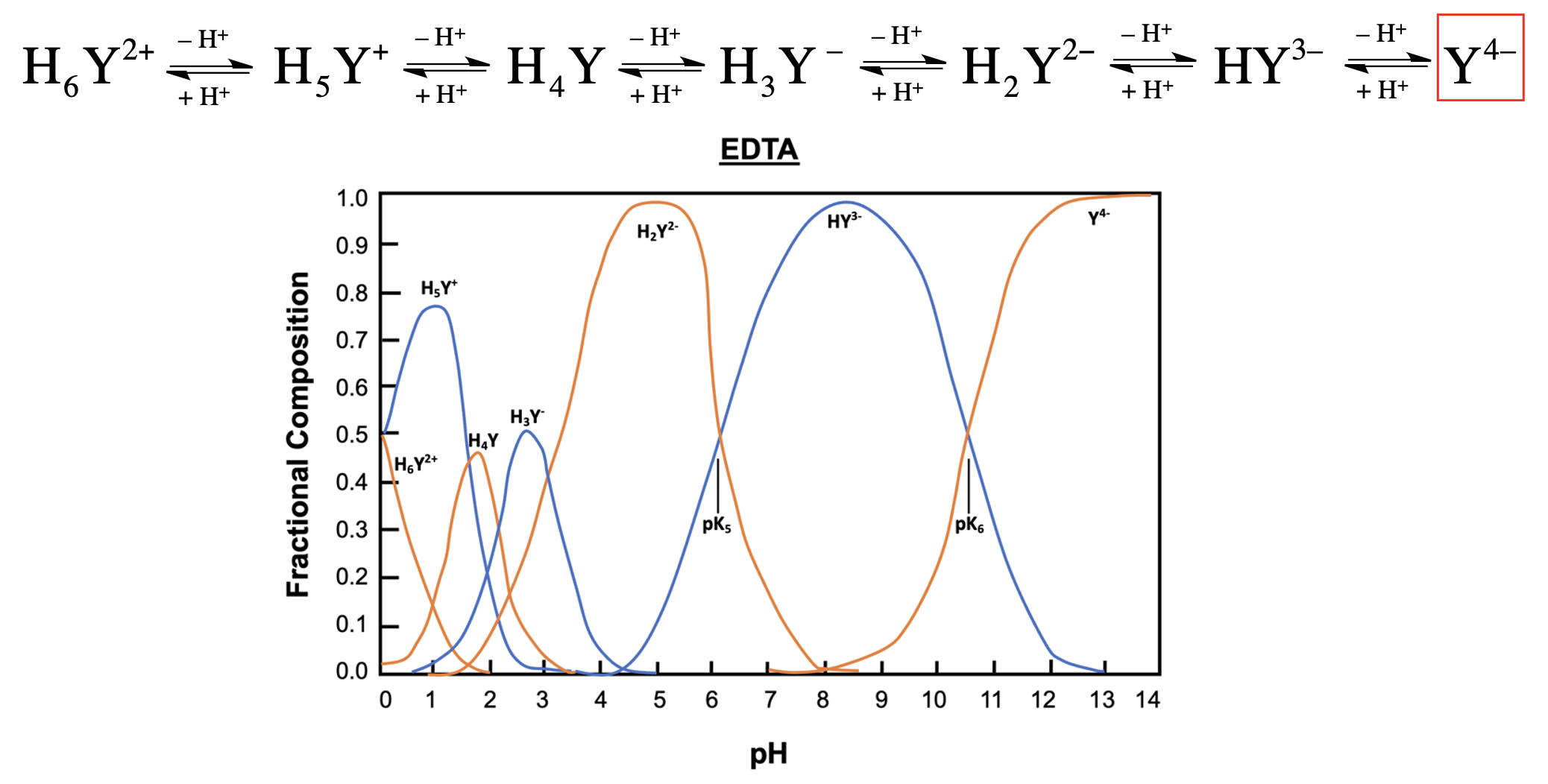
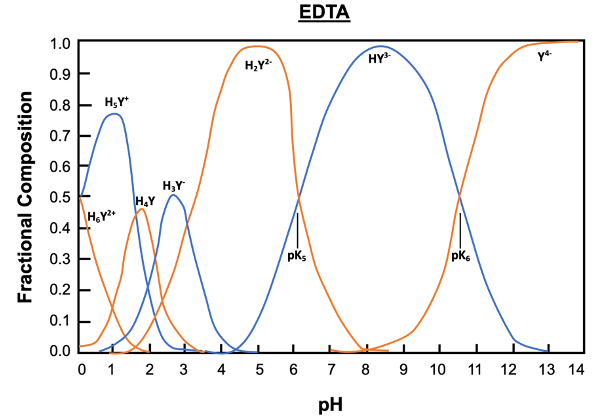
Hey, everyone. So, in this video, we're going to take a look at EDTA. Here, we're going to say in its fully protonated form, EDTA exists as a hexaprotic acid. Here it has the form of H6 because hexa means 6, protic refers to protons y 2 +2 +2+2+2+2+2+2+2+2+2+2+2+2.0 -four, so EDTA -four. Now its form can change based on the number of H+ it possesses. So that can increase or decrease depending on the pH of the solution. We're going to say here that remember for a biological system, carboxylic acids are considered strong organic or biological acids. They tend to have pKa values that are lower. So, here we have a carboxylate group, another one, another one, and another one. 4 carboxylate groups, which correspond to these 4 pKa's. Then, we're going to say that we have these 2 amine groups that can be protonated and therefore have their own pKa values. Those will result in these 2 higher pKa's. Remember we've talked about this in the past, that if your pH is greater than your pKa, then that can result in the deprotonation of a group. And if your pH is less than your pKa, then that can just be protonation. The environment is not basic enough to remove an H+ ion. So here the charge can change for EDTA based on the pH of the solution itself that it's submerged in. Now we're gonna say in order to form metal complexes, these acidic hydrogens must first be removed. We're going to say that EDTA can exist in up to 7 different forms depending on the pH of the solution. So we're taking a look here, we're going to say that this is considered the acidic form or fully protonated form. This exists when the pH of the solution is low enough, so it exists in an acidic environment. What we have here is basically the removal of protons or H+ over time as the pH of the solution increases. So we have 6 protons, 5, 4, 3, 2, 1, and then none. We're going to say that out of all these forms, this one here is the one that is neutral. This neutral form is the one that's easiest to keep intact within a solution. So if we want to transport EDTA over long periods of time submerged in a solution, we typically do it in its neutral form. We keep going and we get to this one here. This form here is our basic form. In this form the pH is high enough that we've removed all the acidic protons from EDTA, so it exists in this EDTA form that we saw earlier. We're going to say that this basic form is the ideal form when dealing with metal complexes. Now if we take a look here at this graph, this graph is basically showing us at different pHs which of the 7 forms predominates. So we're gonna say here that let's say for example here a pH of 6, it aligns with this pKa of 5. This pKa of 5 means that we have kind of an equilibrium with one another, this form, and this form of EDTA. At that pH of 6, we would say that we have an even split between them. So 50% H2Y-2 50% HY-3 At this point here, pKa 6, we're going to say that this is when our pH is around 10.37. We're going to say at that pH we have an equal amount of y-3 and the basic form. Beyond that pH, so higher than 10.37, we'd have the basic form be the dominant or predominant form. Another piece of information that's good to know from this graph is that we can see that below a pH of 3, we can see that 4 of the 7 forms exist. So these are the forms that exist. So basically, they exist when the environment is a pre-acidic pH of 3 and lower. Now, we can say to calculate the fraction of EDTA in its basic form we can utilize the following equation. So here we have basically the fraction, and sub of the basic form equals the concentration of the basic form divided by the concentration of EDTA. So here that would equal the concentration of my basic form. When we say here the concentration of EDTA, we're talking about the concentration of all 7 forms. Now here this can be expanded further into this lower equation. This lower equation, when we have the fraction of our basic form, it's used when the pH of a solution is given and we're asked to find the fraction of the basic form. Because as you can see here, we have concentrations of H+ involved throughout the equation. And remember that if you know pH, you know H+ because H+ equals 10 to the negative pH. And we can see also that we have Ka values being involved in this equation. We also know what our Ka values are because remember that Ka equals 10 to the negative pKa. So when it comes to EDTA, it has a lot of different forms and those forms are determinant on the pH of the solution in which our EDTA structure is submerged. Remember the basic form is the best form when it comes to dealing with metal complexes themselves.
- 1. Chemical Measurements1h 50m
- 2. Tools of the Trade1h 17m
- 3. Experimental Error1h 52m
- 4 & 5. Statistics, Quality Assurance and Calibration Methods1h 57m
- 6. Chemical Equilibrium3h 41m
- 7. Activity and the Systematic Treatment of Equilibrium1h 0m
- 8. Monoprotic Acid-Base Equilibria1h 53m
- 9. Polyprotic Acid-Base Equilibria2h 17m
- 10. Acid-Base Titrations2h 37m
- 11. EDTA Titrations1h 34m
- 12. Advanced Topics in Equilibrium1h 16m
- 13. Fundamentals of Electrochemistry2h 19m
- 14. Electrodes and Potentiometry41m
- 15. Redox Titrations1h 14m
- 16. Electroanalytical Techniques57m
- 17. Fundamentals of Spectrophotometry50m
EDTA - Online Tutor, Practice Problems & Exam Prep
 Created using AI
Created using AIEDTA, a hexaprotic acid, can exist in multiple forms depending on the pH of the solution, influencing its ability to form metal complexes. The basic form of EDTA is most effective for complexation, with formation constants (Kf) indicating the stability of these complexes. The relationship between pH and the fraction of the basic form is crucial for determining the concentration of free metal ions. The conditional formation constant (Kf') incorporates the fraction of EDTA in its basic form, essential for understanding metal-ligand interactions.
EDTA
EDTA
Video transcript
EDTA represents a hexaprotic compound that possesses 7 different forms based on the pH of the solution.
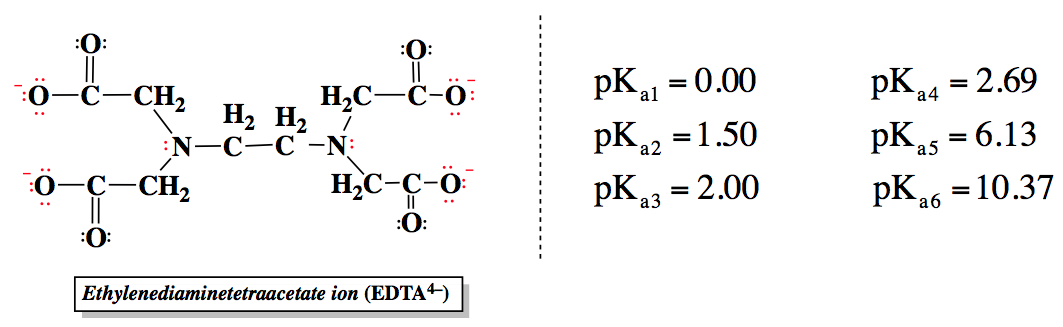
The basic form of EDTA, Y4-, is the predominate form at higher pH values, while the acidic form, H6Y2+, predominates at lower pH values.
The fraction of basic form present in solution can be determined by either given concentrations or by the pH of the solution.
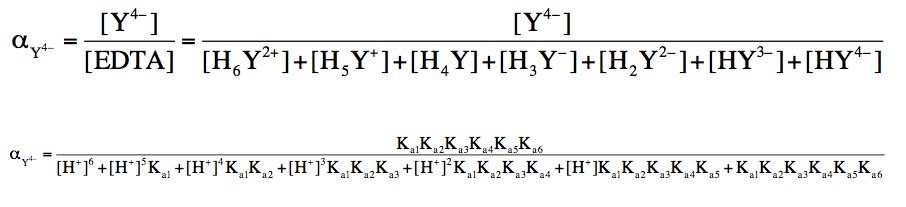
EDTA Calculations
EDTA Calculations 1
Video transcript
So here it states, the formal concentration of EDTA is 1.50 millimolar. Now we're asked, what is the concentration of the basic form at a pH of 5.0? Alright. So, we need to determine what the concentration of our basic form will be at this given pH. And here we have the overall concentration of EDTA. Out of this total concentration, a portion of it belongs to the basic form. We're going to say that the concentration of my basic form equals the initial concentration of EDTA as a whole times the fraction of EDTA that exists in the basic form, which we designate as alpha.
Here, we're going to change our concentration to molarity. You could keep it in millimolar but I'll just change it to molarity. So, remember here that 1 millimolar is equal to 10-3 mol/L. So here we have 1.50×10-3 molar. And here we're dealing with a pH of 5.
What we have here are all our pH values set to a temperature of 25 degrees Celsius and an ionic strength of 0.10 mol/L. Remember, what do we notice? As the pH increases from 0 to 14, look at the fraction that exists in the basic form. We can see that it's increasing. And this makes sense because on the previous page, we saw that chart with the waves and the lines, and we saw that as the pH increased, we got more and more of our basic form taking up the predominant form of the EDTA solution. And that's all this chart here is telling us. As the pH is increasing, a greater percentage or greater fraction of my total EDTA solution exists in the basic form. And what this is telling me is that once I get to pHs of 13 and 14, it's so basic that 100% of my total EDTA solution is in the basic form. All we do now is we just have to look up 5 for pH and here is my alpha for the basic form at that particular pH. We're just going to plug that value in.
So when we multiply that, we get, 4.35×10-10 mol/L. What that answer is telling me, it's telling me that the total amount of EDTA with all 7 forms together totals 1.50×10-3 mol/L. Out of that amount, this is how much of it exists in the basic form at that pH of 5. Just remember, this goes hand in hand with the graph that we saw on the previous page with the waves. As the pH is increasing, the basic form becomes more prevalent. Don't worry. Just come back and see how I approach example 2.

EDTA Calculations 1
Video transcript
For example 2, we need to determine the fraction of our EDTA that exists in the basic form when the pH is 8.50. Now from our chart given here, 8.50 would lie somewhere between 89 of course. We should expect our answer to be somewhere between 4.2 times 10-3 and 0.041. Remember, if they're asking us to determine what our fraction of the basic form of EDTA is and all they give us is the pH, then the formula that we utilize is alpha, our fraction of the basic form equals ka1·ka2·ka3·ka4·ka5·ka6 H+6 + 5·ka1 + H+4·ka1·ka2 + H+3·ka1·ka2·ka3 + H+2·ka1·ka2·ka3·ka4 + H+·ka1·ka2·ka3·ka4·ka5 + ka1·ka2·ka3·ka4·ka5·ka6 . Remember, EDTA in its fully protonated form is a hexaprotic acid. Therefore, it has 6 Ka values or 6 pKa values.
Now, they give us pH. So with pH, we know what the H+ is because remember, H+ equals 10-pH. So it'll be 10-8.50. And then, here we have our pKa values that were: pKa3 was 2, pKa4 was 2.69, pKa5 was 6.13, and then pKa6 was 10.37.
If you know your pKa value, then you know what your Ka value is. So here, we'd say that ka1 equals 10-pKa1. So 10-1.50, 10-2.00, 10-2.69, 10-6.13, and finally, this would be 10-10.37. So all we do here is we plug in these values that we have here into each one of the spaces that we have here. It's a long arduous process but this is the formula we'd have to utilize in order to figure out our fraction of the basic form of EDTA.
When you plug in all those values, so when we plug in all the Ka values and we multiply them with each other, what we would get as a result is 2.04174 times 10-23. Then, we could plug in all the values we have for each one of these here. So when we do that for each one of them, we'd get a bunch of different values. So I'll write them here: 1.0 times 10-51 + 3.16228 times 10-43 + 3.16228 times 10-36 + 1.0 times 10-29 + 6.54 times 10-24 + 1.51356 times 10-21 + 2.04174 times 10-23.
So when you add up all of that on the bottom, you still have what you have on the top. Divided by all of those units added together on the bottom gives us a grand total of 1.54043 times 10-21. So here, when we plug that into our calculators to get our final answer, we get an answer of 0.0133, which is a reasonable answer. Technically, we should only have 2 significant figures for the most part because the majority of those numbers have 2 significant figures. So we say 0.013, which is a reasonable answer because it is a number that falls in between these two values of 4.2 times 10-3 and 0.041. Remember, as our pH is increasing, the fraction of the basic form of EDTA is increasing in amount. And remember, we use this version of the formula when we're only given the pH of the solution.
We use this form to figure out the fraction of the basic form. If they had given us the form of each of the 7 major forms of EDTA, then we'd use the original equation to figure out the fraction of basic form of EDTA. Like both formulas are pretty complex and long, so make sure you carefully plug in the right numbers step by step in order to find the final answer.
Now that you've seen this, answer this practice question that's left on the bottom. This one is much more straightforward. I've given us a graph which you'll have to utilize to find your answer. Once you attempt that, come back and see if your answer matches up with mine.
Determine the pH where αY4– equals 0.20.

EDTA Complexes
EDTA Complexes
Video transcript
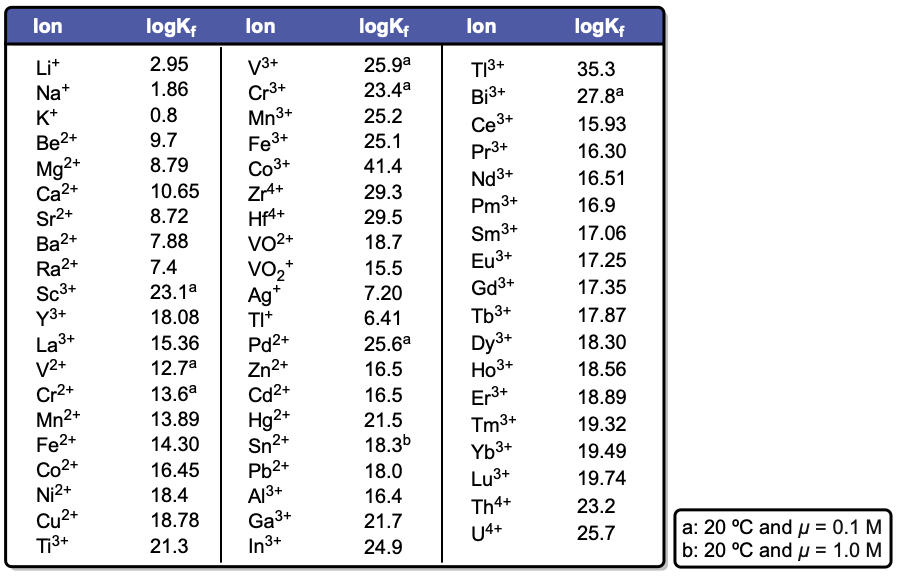
So, with EDTA complexes comes the discussion of our formation or stability constants. Formation or stability constants represent the equilibrium constant for the reaction between a ligand and a metal. Here our formation or stability constant is shown by this variable. Now, the ligand in question that we're dealing with is EDTA. Now the general formula is our metal ion. Here we don't know what its charge is, so we say n+. It could represent a metal with a +1 charge, or a +2 charge, or a +4 charge, or +3 charge. + y-4. y-4 represents EDTA; this is the basic form of EDTA and is the predominant form that is usually used when dealing with a metal ion, but other forms of EDTA could also form ligand connections with the metal. It's just that this one, in particular, is the major form that is usually used. Now, when they combine together, we get our EDTA complex. So here it's m y n4. For example, if you're dealing with calcium, which is +2 in its charge, and we're dealing with EDTA. So calcium is +2, EDTA will be -4 here, so the overall net charge would be -2. So we'd say, CaEDTA(2-). If we're dealing with, let's say, iron 3 with EDTA, so this is +3, this is still -4. So overall, this would be -1. That's how the overall charge works, and here we're dealing with an equilibrium that's being established because we have the reversible arrows and just like with any equilibrium constant, we'll say that our formation or stability constant Kf equals products over reactants. So it would equal the EDTA complex divided by the concentration of my free metal ion times the concentration of EDTA. This formula itself is useful in determining the concentration of free metal ion within the solution. What happens is that not every ounce or every amount of the free metal will connect with EDTA. There will be some free-floating metal ions that are around that haven't combined with every single EDTA molecule. This expression that we have here can be used to determine the amount of free metal ion that's existing within the solution. Now we're going to say the formation constant for metal EDTA complexes is given below, so these are all the values that we have. Realize that some of them have superscripts of a and b. So when it's a, that means the temperature is 20 degrees Celsius, and our ionic strength is 0.1 M, and for the one that's labeled with a subscript of b, that is 20 degrees Celsius still, but now our ionic strength is 1 M. What we should realize here is that the common trend is the higher our charge becomes, then our positive charge becomes, then the greater the affinity between the ligand and the metal, and the higher your Kf value will be. Okay. So again, the more positive the charge, the stronger the connection between the ligand and the metal. The higher your Kf, the more products would be favored, so the more your EDTA complex would be formed. Now, we're going to say, only a portion of EDTA exists in its basic form, and the lower the pH, the more the other forms predominate. Remember, we have to go to pHs of 13 and 14 for 100% of my solution to be in the basic form. If my pH is less than that, then portions of the solution will exist in the other six forms of EDTA. Now here we're going to say under a fixed pH, the fraction of the basic form of EDTA becomes a constant and can be used to determine the conditional formation constant. So our conditional formation constant is here, so it's Kf', so we have this value here to say prime. It represents the formation of our EDTA complex at any pH value. So here our conditional formation constant equals the fraction of your EDTA in basic form times your formation or stability constant and that still equals products over reactants. So it's still the EDTA complex divided by the concentration of free metal ion times the concentration of EDTA. We'll have to utilize these different expressions, equations, as well as values to answer the following questions when it comes to EDTA complexes. So click on to the next video and see how we approach the question where we're asked to find the concentration of free barium ion within our EDTA complex that's formed when it reacts with EDTA itself.
Each metal ion has its own unique formation constant.
The conditional formation constant can be calculated by the determining the fraction of the basic form and the formation constant of the metal ion.
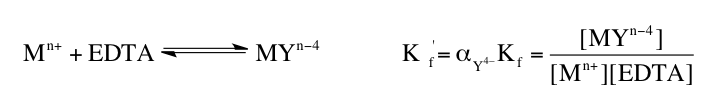
EDTA Complexes
Video transcript
So, here we have to basically discover the amount of free barium ion that exists when we've already formed our EDTA complex. We're going to utilize this equation and substitute in barium for that unknown m. So, we're going to have Ba2+ plus EDTA. Remember, EDTA, we're dealing with its basic form, so its charge is minus 4. So, minus 4 plus 2 from the barium, so the net charge at the end will be minus 2. So, that's how we came up with BaY2-. Now, we're going to have our initial, our change, and our amount at equilibrium. Here they give me an initial concentration for the barium EDTA complex as being 0.10 molar. We don't have any initial amounts for the EDTA and the barium, 2+ ion. Since we have an initial amount for our complex, it's going to be decreasing over time, so this is minus x. These 2 would be plus x. Bring down everything, so this is plus x plus x point 10 minus x.
With any ice chart setups, which we have to figure out equilibrium amounts, we need to know what our formation constant will be in this case, and not just our formation constant, but our conditional formation constant. We're going to have to utilize this portion here. They're telling us that the pH is 10. On our previous video, we said that when the pH is 10, if we look on the chart from a few pages ago, we know that the value of our basic form of EDTA would be 0.30. We need to figure out what our KF is. For barium ion, the log KF of barium is 7.88. We take the inverse of the log function. So, KF of barium ion equals 107.88. When these two are multiplied together, that gives us 2.28 × 107.
Now that we have our conditional formation constant, which is based on the pH given. We're going to say that this constant, like all other constants, equals products over reactants. So, my barium complex divided by my barium ion times EDTA. We're going to plug in the values that we know. So, our conditional formation constant calculated as 2.28 × 107 equals, at equilibrium, 0.10 minus x divided by x2. We're going to have to solve for x, so we multiply both sides by x2. So, 2.28 × 107 × x2 = 0.10 minus x.
Our equation now is 2.28 × 107 x2 + x - 0.10. Next, we're going to use the quadratic formula to isolate our x variable. So, it'll be -1 ± √(1 - 4 × 2.28 × 107 × -0.10) divided by 2a, which is 2 × 2.28 × 107. When we solve, we get two possible values for x: x could be 6.62 × 10-5 molar, or x could be negative 6.62 × 10-5 molar. Remember, at equilibrium, your final concentrations cannot ever be negative, so this cannot be the answer for x. This would be my answer for x, and at equilibrium, x represents the concentration of both EDTA and barium ion. Here we don't care about the EDTA. We're focused on the barium ion, the free-floating barium ion concentration, so this would be our answer.
When it comes to determining the amount of free-floating metal ion, this is the approach we must take. We have to set up a basic ice chart, look at the stoichiometric relationship between EDTA and the free-floating ion in the formation of our EDTA complex. Remember, we have a polydentate EDTA that forms a 1 to 1 ratio with the free-floating metal ion. You also have to determine the conditional formation constant and use that at the particular pH given to determine your final concentration at the end. So, keep in mind the approaches that we used here to find the answer for free-floating metal ions.
EDTA Complexes Calculations
EDTA Complexes Calculations 1
Video transcript
So for this question, it asks us to determine the conditional formation constant for this EDTA complex at a pH of 8.0. Now, remember our conditional formation constant is \( k'_{f} \). It is equal to the fraction of the basic form of EDTA which we'll designate as \( \alpha \), times my formation or stability constant. Now here, if we take a look, remember, EDTA itself is -4 in terms of its charge when dealing with the basic form. The overall charge is -1. For that to occur, cobalt here would have to have a charge of +3. We're dealing with cobalt 3 ion interacting with EDTA to produce this EDTA complex. Now, here they're telling us that our pH is 8.0. Remember, in the chart that we saw a few pages ago, when the pH is 8.0, then the fraction of the basic form of EDTA gives us a value of \( 4.2 \times 10^{-3} \). Here when we're dealing with whole integers in terms of pH, so 0 to 14, we can simply look at that chart and pick the value that we see for the fraction of the basic form of EDTA.
Now remember, if our pH is a value that is not a whole integer, so let's say we had 6.15 instead of 8.0, we'd have to utilize the equation that we've used before to figure out the fraction of the basic form. So remember, that was the one where we had \( k_{a1} \) all the way to \( k_{a6} \) on top divided by \( H^{+6} \) plus all the other portions of the denominator. So that one will be a longer process to figure out the fraction of the basic form of our EDTA species. But again, we're dealing with whole numbers, so we can simply look at the chart and pick the value that we see. Alright. Now we have that. Then when we're dealing with cobalt 3, well, the log of \( K_F \) for cobalt 3 ion equals 41.4. But we don't want the log of \( K_{F} \), we just want \( K_{F} \). So we're going to take the inverse log function. That's \( K_{F} \) of cobalt 3 ion equals \( 10^{41.4} \). So we'll put \( 10^{41.4} \) here and then multiply those two values together. It gives me an answer of \( 1.05 \times 10^{39} \) as the conditional formation constant at that exact pH.
Now remember, your \( k \) value, it's greater than 1. That means that products are highly favored which would mean that when cobalt 3 ion comes into contact with EDTA, there is a high propensity or a high desire to form this complex here. That's what our \( k_{F} \) value was telling us here. Remember, the more positive the charge of the metal ion, the greater the affinity the EDTA molecule or ligand has for the positive ion. The more they're going to combine together to help make this complex here. Now that we've seen this simple example, move on to example 2. Remember and utilize some of the techniques that we used in the previous page to help figure out the amount of free tin 2 ion within this example given. So good luck, guys.
EDTA Complexes Calculations 1
Video transcript
Here we need to find the concentration of free, tin 2 ions in 0.20 molar of this EDTA complex at a pH of 9. Now what we have here is we have a potassium ion involved, but then this right here has to be SnEDTA2-. And it's this complex, EDTA complex, that we're focused on. Here, for it to have a negative two charge overall, that means that tin had to have been plus 2. EDTA was minus 4, and that’s how we got that charge of minus 2 at the end. We won't pay attention to the potassium which is acting as a spectator ion. So we'll have our initial amount, the change, and then what we have at equilibrium. We have 0.20 molar of our EDTA complex. Initially, we have 0 of these 2 reactants. We’re losing some of our EDTA complex in order to create some of these free-floating metal ions and EDTA. We bring down everything.
Now, we need to determine what our conditional formation constant will be. So k′f=α, the portion or fraction of our basic form of EDTA times our formation or stability constant. We're working here with 9.00 as our pH. If we look at our table, we find that the fraction of the basic form of EDTA at a pH of 9 comes out to 0.041. Then if we look at our chart which looks at the metal EDTA complexes and the formation constants connected to them, kf of Sn2+ equals 10 to the power of 18.3. So, 10 to the power of 18.3. Multiplying those two together gives me 8.18 × 1016 as my conditional formation constant. Now that we have that, we can set up our equilibrium expression and solve for our missing x variable.
So here, 8.18×1016
Find the concentration of free Na + in 0.15 M Li 3[Na(EDTA)] at pH = 10.00.
Here’s what students ask on this topic:
What is EDTA and how does it function as a hexaprotic acid?
EDTA (Ethylenediaminetetraacetic acid) is a hexaprotic acid, meaning it can donate six protons (H+). In its fully protonated form, it is represented as H6Y. The structure of EDTA includes four carboxylate groups and two amine groups, each with their own pKa values. The form of EDTA changes with the pH of the solution, affecting its protonation state. At low pH, EDTA is fully protonated, while at high pH, it is fully deprotonated, existing as Y4-. This deprotonated form is most effective for forming metal complexes, as it can bind to metal ions through its multiple donor sites.
 Created using AI
Created using AIHow does pH affect the different forms of EDTA?
The pH of a solution significantly affects the form of EDTA. At low pH (acidic conditions), EDTA is fully protonated (H6Y). As the pH increases, protons are gradually removed, leading to forms like H5Y-, H4Y2-, and so on, until it reaches the fully deprotonated form Y4- at high pH (basic conditions). The neutral form (H2Y2-) is often used for transport in solutions. The basic form (Y4-) is ideal for forming metal complexes, as it can effectively bind to metal ions.
 Created using AI
Created using AIWhat are formation or stability constants (Kf) in the context of EDTA complexes?
Formation or stability constants (Kf) represent the equilibrium constant for the reaction between a ligand (like EDTA) and a metal ion. The general formula for this reaction is Mn+ + Y4- ⇌ MY(n-4)-. The Kf value is calculated as the concentration of the EDTA-metal complex divided by the product of the concentrations of the free metal ion and EDTA. Higher Kf values indicate stronger and more stable complexes. The Kf values vary with the charge of the metal ion and the pH of the solution.
 Created using AI
Created using AIHow do you calculate the fraction of EDTA in its basic form?
The fraction of EDTA in its basic form (Y4-) can be calculated using the equation:
Here, [Y4-] is the concentration of the basic form, and [EDTA] is the total concentration of all forms of EDTA. This can be expanded to include pH and pKa values, as:
where n is the number of protons removed, and Ka values are the dissociation constants.
 Created using AI
Created using AIWhat is the conditional formation constant (Kf') for EDTA complexes?
The conditional formation constant (Kf') accounts for the fraction of EDTA in its basic form (Y4-) at a given pH. It is calculated as:
Here, αY is the fraction of EDTA in its basic form, and Kf is the formation constant. This allows for the determination of the stability of EDTA-metal complexes at different pH levels, considering that not all EDTA will be in the Y4- form at lower pH values.
 Created using AI
Created using AI