Titrations are an important topic that we're going to discuss when it comes to stoichiometric reactions, but we'll talk about it consistently when we're dealing with terms that you should recall. Here we're going to say that when we're dealing with gravimetric analysis, this is just basically helping us to determine the amount of product that we can isolate within a chemical reaction. And we're going to say the calculations that we use to determine that isolated amount is termed stoichiometry. Now, with stoichiometry, we have to employ the use of the stoichiometric chart. 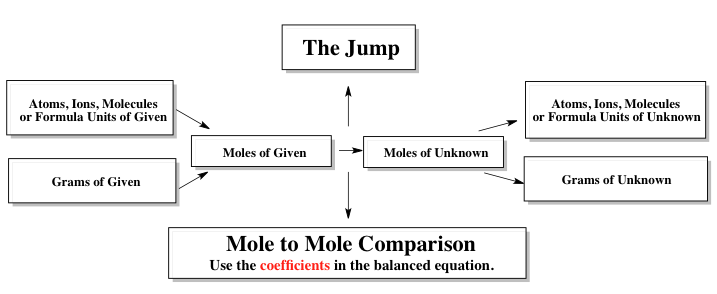Remember, with the stoichiometric chart, we'll be given a balanced chemical reaction and from that balanced chemical reaction, we'll be given amounts for either some elements and compounds and asked to find the unknown quantity of some other element or compound within that balanced equation. Now with the stoichiometric chart, we have our given information which typically is given to us in some units of mass or substance. They may give us given amounts in grams, possibly moles. Remember, when we're going from grams to moles, we use the molecular weight of the compound itself. They may give us the initial amount in atoms, ions, molecules, or formula units. In this case to go to moles, we'd use Avogadro's number which is 6.02223. Remember when we use the term atoms, atoms are for basically neutral single elements. So, if we're talking about an oxygen atom, we're just talking about O. We use the term for ions when we have a single element with a charge. Then the term molecules are used for covalent compounds which are just nonmetals connected together. And formula units are used for ionic compounds where we have a positive ion connected to a negative ion. Now, using the stoichiometric chart, we'll be given information. Usually one of these values and it's our job to go to the unknown amount of our other compound or element within the balanced equation. Now, when we're looking for the unknown, they may ask us to find the unknown in moles, atoms, ions, molecules, formula units, or grams itself. Remember, as we're going from an area where we know stuff because it's given to us to an area where we know nothing at all, we have to make basically a leap from one side to the other side. That's why we call this the jump as we go from the side where we are given information to the side where we have our unknown. Remember, when we make this jump, we have to do a multiple comparison. That's why a balanced equation is needed with stoichiometry because when we do this multiple comparison, we have to use the coefficients within the balanced equation. Knowing this basic setup for the stoichiometric chart will be essential for any type of titration, stoichiometric calculations that we're going to follow in the example question that's left on the bottom by how I answer the following example question that's left on the bottom by using the stoichiometric chart and what we remember dealing with stoichiometric.
Volumetric Titrations - Online Tutor, Practice Problems & Exam Prep
Classical Stoichiometric Methods involve the use of both volumetric methods (titrations) and gravimetric methods.
Titrations
Volumetric Titration
Video transcript
Volumetric Titration
Video transcript
So let's take a look at the example given below. Here it says that iron(III) can be oxidized by an acidic potassium dichromate solution according to the net ionic equation below. We're asked how many microliters of a 0.250 molar iron(II) chloride solution are needed to completely react with 9.12 grams of a compound containing 41.5 percent potassium dichromate. Alright. Remember, with any type of board problem, even stoichiometric ones, we always start out with what exactly they are asking me to find. We're being asked to find microliters of my iron(II) chloride solution. Next, we write down all the given information that we have. From the information that we can see, we have 0.250 molar iron(II) chloride. What is that really saying? Remember, molarity is moles over liters. So that's really saying that we have 0.250 moles of iron(II) chloride over 1 liter. We have 9.12 grams of a compound. And it's saying that of that 9.12 grams of that compound, 41.5 weight percent of it is potassiu
Titrations Calculations
Titrations Calculations 1
Video transcript
We continue our discussion of titrations with the following example question. Here it says magnesium reacts with hydrochloric acid according to the reaction below. We are asked how many grams of 5.310% by weight of aqueous magnesium are required to provide a 25% excess to react with 75 ml of 0.0550 molar hydrochloric acid. So, in this question, what they're asking us to find is the grams of my aqueous magnesium solution. So, we're looking for grams of solution. From the information provided, we need to isolate that variable. So, if we take a look here, we're going to say that they're giving us 5.310 percent. That means that we have 5.310 grams of magnesium for every 100 grams of solution. We're told here that we have 75 ml of 0.0550 molar hydrochloric acid. Remember the word 'of' means multiply. So we need to realize here that if I can change my ml's into liters and then multiply it by the molarity, I'll get the moles of hydrochloric acid. So, that's what we're going to do. We're going to change ml's into liters. Remember, 1 liter is 1000 milliliters. That molarity there really means 0.0550moles of hydrochloric acid over 1 liter of solution. And now that we have the moles of HCl, realize here that that's going to give me 0.004125 moles of hydrochloric acid. This number here represents our 100% amount of hydrochloric acid. But in the question itself, it's saying that we need to do a 25% excess. That means that we're going to take these moles and we're going to multiply them by 25% excess. That's 100% + 25%, which is 125%. Again, we can't use percentages within our calculations, so we'll have to divide this by 100. So that's 1.25. When I multiply these moles by 1.25, that gives me a new set of moles as 0.005156 moles of HCl. So this represents my 25 percent excess. All right. So now, all we n
Titrations Calculations 1
Video transcript
So continuing with our discussion of volumetric titrations, we have example 2. Here it states that the density of a 2.20 molar solution of methanol is 0.976 grams per milliliter. It asks us what the molality of the solution is. The molar mass of methanol is given as 31.034 grams per mole. Remember that molality is denoted as lowercase m. Molality equals our moles of solute divided by kilograms of solvent. This will require a bit of work on our part to isolate moles of solute and the kilograms of solvent. From the information given, we see that we have a 2.20 molar solution. Molarity itself equals moles of solute over liters of solution. So this 2.20 molar is equivalent to 2.20 moles of solute, which would be methanol, divided by 1 liter of solution. Also, we're given the density of our solution, so the density (d) is 0.976 grams of solution per 1 milliliter of solution. The molar mass is additional information that we could calculate on our own, so you don't even need to consider that given information at this point. Without doing any math, we can see that we can isolate the moles of solute because for molarity, we have the moles of our solute, which is methanol. So we already have that part figured out.
Now, the hard part comes from identifying your kilograms of solvent. To do that, realize that we have the volume of our solution, which is 1 liter, and we have the density. When we multiply those together, that gives us 976 grams of solution. Remember, a solution is comprised of two parts: solute and solvent. If I can isolate the solute, what I'll have left are grams of solvent. Remember, our solute is methanol. We have the moles of methanol from the very beginning. I'm going to convert those into grams and subtract them from the grams of solution, and what will be left are our grams of solvent. So, take the 2.20 moles of methanol, use the molar mass given to us, so it's 1 mole of methanol for every 31.034 grams of methanol. Doing that gives us a mass of 68.2748 grams of methanol. Subtracting it from the 976 will give us 907.7252 grams of solvent. Now that we have those grams of solvent, just change them into kilograms. We have 907.725 grams of solvent, and we're going to say here for every 1 kilogram, there are 1,000 grams. That's 0.907725 kilograms of my solvent. Take that and plug it up here. When we divide those two numbers, we're going to get 2.42 molal as our answer. So that will represent the molality of our solution. Remember, we can isolate the molality of a solution if we have the density of the solution and the molarity of the solution. Using these approaches, you'll be able to answer any questions similar to this one.
Titrations Calculations 2
Video transcript
In this video, we take a look at a back titration. Now, in a back titration, we have 2 compounds reacting with one another and at the end, we're going to have an excess remaining of one of the reagents. With that excess, we then react it with a second compound. This can help us determine the concentration of the initial analyte or it could help us to figure out the volume of the second compound being used. The variety of questions from a back titration can be endless depending on how you write it. In this first example, we'll take a look at a typical type of back titration question. Here it says, 0.4317 grams sample of calcium carbonate is added to a flask that also contained 120.50 ml of 1.530 molar hydrobromic acid. Now, here we have our balanced equation for hydrobromic acid. It says additional water is then added to create a 250 ml solution A. Next, a 20 ml aliquot of solution A is taken and titrated with 0.0980 molar sodium hydroxide. We're asked at the end how many milliliters of sodium hydroxide were used. Alright, so in this question, we need to figure out what our ml's of sodium hydroxide are. What we need to realize here is that we have potassium carbonate reacting with hydrobromic acid. And we're going to say here, if we were the first step we're going to do is just set up a regular stoichiometric example. We're going to say here that we have 0.4317 grams of calcium carbonate. We're going to say here for every 1 mole of calcium carbonate, how many grams of calcium carbonate do we have in that? Well, we say that calcium, we have 1 calcium, one carbon, and 3 oxygens. Based on the periodic table, their masses are 40.078 grams, 12.0107 grams, and 15.99994 grams. Multiply across, add up the totals, gives us 100.087 grams of calcium carbonate. At this point, we're now going to see what the stoichiometric relationship is between calcium carbonate and hydrobromic acid. Here we're going to say according to my balanced equation, for every one mole of calcium carbonate, there are 2 moles of hydrobromic acid. All we're doing at this point is just figuring out stoichiometrically how many moles of hydrobromic acid would be needed to completely react with this amount of calcium carbonate. That's all we're doing. So for that many grams of calcium carbonate we need exactly 0.008627 moles of hydrobromic acid. What we have to do now is we have to figure out from this volume and this molarity how many moles of HBr do we actually have present. We're going to change our ml's into liters. Remember your molarity is moles over liters. So that comes out to 0.019125 moles of hydrobromic acid hydrobromic acid present. So you can clearly see that the amount of HBr needed is smaller than the amount of HBr that we have present. That really asks us now how much of the HBr was left unused? We have this initial amount of hydrobromic acid minus what we need. So this is how much hydrobromic acid we have left from the first equation. So this is how many moles of HBr we have left and we're told that water is added to this moles of HBr left until we have a volume of 250 ml's. So that's saying that we have 0.01048 moles of HBr, and it's connected to 0.250 liters. All I did here is I converted ml's into liters. Next, they say that we take a 20 ml aliquot of that amount. Okay. So all I'm doing is I'm taking 20 ml's of that HBr. What does that mean? So 20 ml's when I change it to liters is 0.020 liters. That portion is just telling me to multiply those 2 together to figure out how many moles of HBr I took out from just that 20 ml aliquot portion. Remember, we're trying to figure out how many ml's of NaOH we have. We have the molarity of NaOH. So what we're going to do here is we're going to say, according to this second equation, for every 1 mole of NaOH, I have 1 mole of HBr. So for every one mole of HBr, we have one mole of NaOH. Then, all I have to do at this point is I'm going to use the molarity now that's right here, which is 0.0980 moles of NaOH for every 1 liter. And for every 1 liter, we have 1000 milliliters. So at the end, that gives me 8.5698 ml's. And here we're going to do 3 sig figs because this number here has 3 sig figs compared to the others which have 4. So this is 8.57 ml of NaOH would have been needed in order to completely neutralize any excess HBr left. So this is a little bit different from what we're accustomed to seeing with titration questions. Remember in a back titration question, we utilize 2 different equations. We'll have some excess of an analyte left so we'll have to use a second compound in order to neutralize the remainder of that analyte. Doing that can help us to determine the molarity of the second compound, its volume, even its molar mass. In this example, we use that excess HBr and compared it to the NaOH in order to figure out the volume of the NaOH that was present.
Titrations Calculations 2
Video transcript
Here is an example that says, "The amount of iron can be determined by using dichromate to oxidize Iron 2 to Iron 3. The equation for this process is shown below." Here, it says, "Determine the percentage by mass or percent by mass of Iron in 0.3500 grams of ore sample, if the complete titration of Iron 2 requires 20.7 milliliters of a 0.0185 molar dichromate solution." Alright. So here, they're asking us to find the percent by mass of iron. So, we're going to say here, percent by mass or mass percent would equal the grams of iron, and it's in terms of Iron 2 ion, divided by the grams of the ore sample, times 100. We already know a portion of this because we know how much ore sample we have, which is 0.3500 grams. All we need to do now is use solution stoichiometry to determine the grams of Iron 2 ion.
So, to do that, we look at this information given to us for dichromate. Remember the word "of" means multiply. And remember, moles equal liters times molarity. So what I'm going to do here is divide these mL by 1000. That'll give me 0.0207 liters. I can then multiply by 0.0185 molar dichromate. That's going to give me 3.8295×10-4 moles of dichromate ion. Since I know the moles of dichromate ion, I can use stoichiometry to find the moles of Iron 2 ion. So here, we're going to set up a mole to mole comparison. Moles of dichromate go here on the bottom. Moles of Iron 2 ion go up here. Since we're doing a mole to mole comparison, we need to look at the coefficients of the balanced equation, and it's for every 1 mole of dichromate, there are 6 moles of Iron 2 ion. Moles cancel out, and now we're going to have grams of Iron 2 ion. So we have 1 mole of Iron 2 ion, and according to the periodic table, iron weighs 55.85 grams, including this ion. So, at the end, we're going to get our grams here, which comes out to 0.1283 grams of Iron 2 ion. Take that and plug it over here. When we work this out, we get 36.7% as the percent by mass of iron within this ore sample. So this will be our final answer.
Titrations Calculations
Video transcript
For this example question, it asks what the molar mass of a 0.750 gram sample of a diprotic binary acid is if it requires 50 ml of 0.440 molar calcium hydroxide to completely neutralize it. First of all, we're dealing with an acid and a base mixing, so we have a titration. We need our balanced equation. Here they say "diprotic," which means it has 2 acidic hydrogens so H2. "Binary" just means it doesn't have oxygen present, so here our placeholder, since we don't know what it is, would be A. So, H2A is a generic formula for a diprotic binary acid. Then, we are going to add calcium hydroxide, which would give me: when this breaks up into ions, it breaks up into H-, A2-, Ca2+, OH-. We know opposites attract, so this negative and this positive will combine together giving me water, and then this 2- and 2+ will be attracted to each other and give me calcium A. Balancing this, we'd have to just put a 2 here. So, now our equation is balanced. All the other coefficients are just 1.
Now, they're asking for the molar mass of this diprotic binary acid. Remember, molar mass itself is grams per mole. Technically, you already know half of the answer because you already know how many grams of this acid you possess: 0.750 grams. To figure out the moles, we'll have to utilize stoichiometry. Now, remember the word "of" means multiply when it's between two numbers. And remember that molarity equals moles over liters. If you multiply both molarity and liters, you would see that they equal moles. So moles equals liters times molarity. So we just divide this by 1,000 to get liters and multiply by the molarity to get moles of calcium hydroxide. So here we're going to work this out, so we have 50 ml's, and we're going to say here that 1 liter equals 1,000 milliliters, or 1 milliliter equals 10-3 liters, either way is fine. Then we're going to say molarity is moles over liters. So it's this many moles of calcium hydroxide over 1 liter. Now because we know the moles of calcium hydroxide, we can do a mole to mole comparison to find the moles of our diprotic acid. So according to my balanced equation, it's a one to one relationship. For every 1 mole of calcium hydroxide, we have 1 mole of our diprotic acid. So here, these cancel out with these. And when I plug this in, it gives me my moles of my diprotic acid. It comes out to be 0.022 moles of my diprotic acid. So just write it over here for additional reference. So now, take the grams we had from the beginning, take the moles we just calculated, together they give me my molar mass, which is 34.09 grams per mole. So, this particular diprotic binary acid has that molar mass, 34.09 grams per mole. This is our final answer.
A 1.000 g sample of Na2CO3 (MW: 105.99 g/mol) is dissolved in enough water to make 200.0 mL of solution. A 25.00 mL aliquot required 32.18 mL of HCl to completely neutralize it. What is the molar concentration of HCl?
Na2CO3 (aq) + 2 HCl (aq) → 2 KCl (aq) + H2O(l) + CO2 (g)
