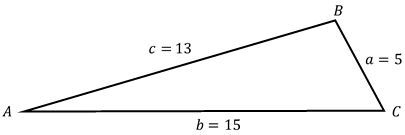
If I give you this triangle on the left and ask you to solve for side c, you'd be able to do it given everything we've learned about the law of sines. Because we have a side and its opposing angle, all we need is another piece of information, and we could set up a law of sines between 2 ratios in which we know 3 out of 4 variables. We've seen how to do this before. But sometimes, in some triangles, you won't be given a side and its opposite angle. Here we have a and b, but not the angles. Here, we have angle c, but not its corresponding side. Whenever this happens, you can't use the law of sines, and I'll show you why in just a second. It might seem like we're going to get stuck here, but what I'm going to show you is that we'll need a different set of equations to solve these types of problems called the law of cosines. What I'm going to show you is that it's actually a very straightforward set of equations, and most of the time when you use it, it's pretty much just plug and chug. So let's just jump right into this example, and I'll show you how it works. Alright?
So we've seen how to solve these types of problems on the left where we set up a law of sines. Basically, just going to ignore side a because I don't know anything about it, and I've already got the answer sort of written out for you. All it is just c equals 4.9. So we could figure this out pretty quickly.
Why doesn't it work for the triangle on the right side? Well, basically, let's just write out all of the law of sines, the whole entire thing. So I'm going to write that sineaa = sinebb = sinecc. What do I know about this problem? I know the two sides, a and b, but I don't know the angles. Here, I have the angle c, but not the corresponding side. So in each one of the terms, I only know one out of the two variables. And what's worse is that because I only have one angle, I can't solve for the other two angles because I have 2 unknowns there. So it seems like I'm kind of stuck here. I can't actually solve for side c. And that's because in these types of situations, you just can't use the law of sines. You have too many unknowns.
So what I'm going to do here is I'm just going to go ahead and show you the law of cosines. The law of cosines really just is sort of a pattern, and there are sort of 3 variations because the letters could be different, and it depends on what you're trying to solve for, but the pattern is all the same. So for a and b, the equation is already written. For c, I'm just going to go ahead and give it to you. c2 = a2 + b2 - 2 times the two letters that you just wrote, so a and b, times the cosine of the corresponding angle that's over here on the left side. So that's always going to be the pattern for a and b. You can just double-check it over here.
So, really, what this is, law of cosines, is it's just a set of equations that relates the squares of the three triangle sides to a known angle. That's the most important thing here. You're going to relate these things to an angle which you know. And in this case, we do because we have the angle big c. We know what that angle is.
So if I'm trying to solve for little c over here, I'm just going to use this variation of the law of cosines. Alright. So we're going to see that c2 = a2 + b2 - 2ab cosc. Pretty straightforward. The rest is basically just plug and chug because you have all of these numbers. So a is 3, so this isr 9 + 4, so this is 13 - 12 times the cosine of 60 degrees. This basically ends up being 13 - 6, which equals 7. So when you take the square root, what you're going to see here is that c is equal to the square root of 7. Alright. So you obviously could have turned this into a decimal at any point. I just sort of kept it a little bit clean like a square root, but that's basically it.
So we just know that C is equal to the square root of 7. That's how you use the law of cosines. So I just want to point out a couple of things here. You can also use the law of cosines to solve for any missing angles as long as you have the other three sides, so you can totally do that. And the last thing I want to mention here is you may have noticed at some point when I was writing this that this kind of looks like the Pythagorean theorem. We've got a squared + b squared = c squared, but then you have this other term over here. That's actually because the law of cosines, or, sorry, the Pythagorean theorem, is really just a special case of the law of cosines. But that's just a fun fact. You don't really need to know that. Anyway, thanks for watching, and I'll see you in the next one.