Here are the essential concepts you must grasp in order to answer the question correctly.
Cosine Function
The cosine function, denoted as cos(x), is a fundamental trigonometric function that relates the angle x in a right triangle to the ratio of the length of the adjacent side to the hypotenuse. It is periodic with a period of 2π, meaning that cos(x) repeats its values every 2π radians. Understanding the behavior of the cosine function is essential for solving equations involving it.
Recommended video:
Graph of Sine and Cosine Function
Inverse Trigonometric Functions
Inverse trigonometric functions, such as arccos(x), are used to find angles when the value of a trigonometric function is known. For example, if cos(x) = -1/2, we can use arccos(-1/2) to find the principal angle. However, since trigonometric functions are periodic, multiple angles can satisfy the equation, necessitating a comprehensive approach to find all solutions.
Recommended video:
Introduction to Inverse Trig Functions
General Solutions of Trigonometric Equations
When solving trigonometric equations, it is important to find all possible solutions within a specified interval or in general terms. For cosine equations, the general solution can be expressed as x = arccos(value) + 2nπ or x = -arccos(value) + 2nπ, where n is any integer. This accounts for the periodic nature of the cosine function and ensures that all angles that satisfy the equation are included.
Recommended video:
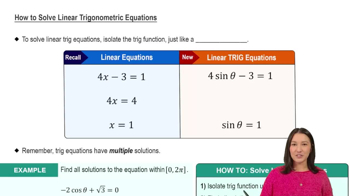
How to Solve Linear Trigonometric Equations
 Verified Solution
Verified Solution


 4:25m
4:25m
