Table of contents
- 0. Review of College Algebra4h 43m
- 1. Measuring Angles39m
- 2. Trigonometric Functions on Right Triangles2h 5m
- 3. Unit Circle1h 19m
- 4. Graphing Trigonometric Functions1h 19m
- 5. Inverse Trigonometric Functions and Basic Trigonometric Equations1h 41m
- 6. Trigonometric Identities and More Equations2h 34m
- 7. Non-Right Triangles1h 38m
- 8. Vectors2h 25m
- 9. Polar Equations2h 5m
- 10. Parametric Equations1h 6m
- 11. Graphing Complex Numbers1h 7m
6. Trigonometric Identities and More Equations
Introduction to Trigonometric Identities
Problem 5.70c
Textbook Question
Verify that each equation is an identity.
(sin 3t + sin 2t)/(sin 3t - sin 2t ) = tan (5t/2)/(tan (t/2))
 Verified step by step guidance
Verified step by step guidance1
<Step 1: Use the sum-to-product identities for sine. The sum-to-product identities are: $\sin A + \sin B = 2 \sin\left(\frac{A+B}{2}\right) \cos\left(\frac{A-B}{2}\right)$ and $\sin A - \sin B = 2 \cos\left(\frac{A+B}{2}\right) \sin\left(\frac{A-B}{2}\right)$.>
<Step 2: Apply the sum-to-product identities to the numerator and denominator of the left-hand side. For the numerator $\sin 3t + \sin 2t$, use $A = 3t$ and $B = 2t$. For the denominator $\sin 3t - \sin 2t$, use the same values for $A$ and $B$.>
<Step 3: Simplify the expressions obtained from the sum-to-product identities. The numerator becomes $2 \sin\left(\frac{5t}{2}\right) \cos\left(\frac{t}{2}\right)$ and the denominator becomes $2 \cos\left(\frac{5t}{2}\right) \sin\left(\frac{t}{2}\right)$.>
<Step 4: Divide the simplified numerator by the simplified denominator. This results in $\frac{2 \sin\left(\frac{5t}{2}\right) \cos\left(\frac{t}{2}\right)}{2 \cos\left(\frac{5t}{2}\right) \sin\left(\frac{t}{2}\right)}$.>
<Step 5: Simplify the expression by canceling out the common factor of 2 and using the identity $\frac{\sin A}{\cos A} = \tan A$. This gives $\tan\left(\frac{5t}{2}\right) / \tan\left(\frac{t}{2}\right)$, which matches the right-hand side of the original equation, verifying the identity.>
Recommended similar problem, with video answer:
 Verified Solution
Verified SolutionThis video solution was recommended by our tutors as helpful for the problem above
Video duration:
0m:0sPlay a video:
Was this helpful?
Key Concepts
Here are the essential concepts you must grasp in order to answer the question correctly.
Trigonometric Identities
Trigonometric identities are equations that hold true for all values of the variable where both sides are defined. Common identities include the Pythagorean identities, angle sum and difference identities, and double angle formulas. Understanding these identities is crucial for verifying equations and simplifying expressions in trigonometry.
Recommended video:

Fundamental Trigonometric Identities
Sine and Tangent Functions
The sine function, sin(t), represents the ratio of the opposite side to the hypotenuse in a right triangle, while the tangent function, tan(t), is the ratio of the sine to the cosine of the angle, or sin(t)/cos(t). These functions are periodic and have specific properties that can be used to manipulate and verify trigonometric equations.
Recommended video:

Sine, Cosine, & Tangent of 30°, 45°, & 60°
Algebraic Manipulation of Trigonometric Expressions
Algebraic manipulation involves rearranging and simplifying trigonometric expressions using identities and properties. This includes factoring, combining fractions, and substituting equivalent expressions. Mastery of these techniques is essential for verifying identities and solving trigonometric equations effectively.
Recommended video:

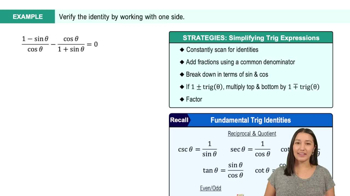
Simplifying Trig Expressions

 6:19m
6:19mWatch next
Master Even and Odd Identities with a bite sized video explanation from Callie Rethman
Start learningRelated Videos
Related Practice