Table of contents
- 0. Review of College Algebra4h 43m
- 1. Measuring Angles39m
- 2. Trigonometric Functions on Right Triangles2h 5m
- 3. Unit Circle1h 19m
- 4. Graphing Trigonometric Functions1h 19m
- 5. Inverse Trigonometric Functions and Basic Trigonometric Equations1h 41m
- 6. Trigonometric Identities and More Equations2h 34m
- 7. Non-Right Triangles1h 38m
- 8. Vectors2h 25m
- 9. Polar Equations2h 5m
- 10. Parametric Equations1h 6m
- 11. Graphing Complex Numbers1h 7m
2. Trigonometric Functions on Right Triangles
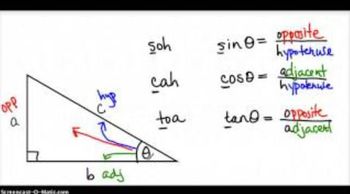
Trigonometric Functions on Right Triangles
Problem 22a
Textbook Question
Solve each problem. See Examples 1 and 2. Distance between Two Ships Two ships leave a port at the same time. The first ship sails on a bearing of 52° at 17 knots and the second on a bearing of 322° at 22 knots. How far apart are they after 2.5 hr?
 Verified step by step guidance
Verified step by step guidance1
Convert the speed of each ship from knots to nautical miles per hour. Since 1 knot is equal to 1 nautical mile per hour, the first ship travels at 17 nautical miles per hour and the second at 22 nautical miles per hour.
Calculate the distance each ship travels in 2.5 hours. For the first ship, multiply 17 nautical miles per hour by 2.5 hours. For the second ship, multiply 22 nautical miles per hour by 2.5 hours.
Determine the angle between the paths of the two ships. Since bearings are measured clockwise from north, the angle between the two bearings (52° and 322°) is the difference between them, which is 322° - 52°.
Use the Law of Cosines to find the distance between the two ships. The formula is: \( c^2 = a^2 + b^2 - 2ab \cdot \cos(C) \), where \( a \) and \( b \) are the distances traveled by the ships, and \( C \) is the angle between their paths.
Solve the equation from the Law of Cosines to find the distance \( c \) between the two ships after 2.5 hours.
Recommended similar problem, with video answer:
 Verified Solution
Verified SolutionThis video solution was recommended by our tutors as helpful for the problem above
Video duration:
6mPlay a video:
Was this helpful?
Key Concepts
Here are the essential concepts you must grasp in order to answer the question correctly.
Bearing
Bearing is a navigation term that describes the direction of one point from another, measured in degrees from the north. In this problem, the bearings of the ships (52° and 322°) indicate their respective directions relative to true north. Understanding bearings is crucial for visualizing the paths of the ships and calculating the angle between them.
Velocity and Distance
Velocity is the speed of an object in a specific direction, expressed in units such as knots (nautical miles per hour). To find the distance traveled by each ship, multiply their speed by the time traveled. In this case, each ship's distance after 2.5 hours can be calculated, which is essential for determining how far apart they are.
Recommended video:

Example 1
Law of Cosines
The Law of Cosines is a formula used in trigonometry to find the lengths of sides in a triangle when two sides and the included angle are known. In this scenario, it helps to calculate the distance between the two ships by treating their paths as sides of a triangle, where the angle between them can be derived from their bearings.
Recommended video:

Intro to Law of Cosines

 6:4m
6:4mWatch next
Master Introduction to Trigonometric Functions with a bite sized video explanation from Nick Kaneko
Start learningRelated Videos
Related Practice