Table of contents
- 0. Review of College Algebra4h 43m
- 1. Measuring Angles39m
- 2. Trigonometric Functions on Right Triangles2h 5m
- 3. Unit Circle1h 19m
- 4. Graphing Trigonometric Functions1h 19m
- 5. Inverse Trigonometric Functions and Basic Trigonometric Equations1h 41m
- 6. Trigonometric Identities and More Equations2h 34m
- 7. Non-Right Triangles1h 38m
- 8. Vectors2h 25m
- 9. Polar Equations2h 5m
- 10. Parametric Equations1h 6m
- 11. Graphing Complex Numbers1h 7m
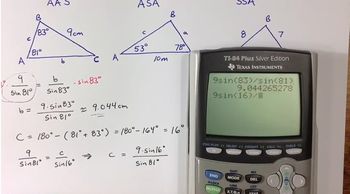
7. Non-Right Triangles
Law of Sines
Problem 42
Textbook Question
In Exercises 41–42, find a to the nearest tenth.
 Verified step by step guidance
Verified step by step guidance1
Identify the trigonometric function or relationship involved in the problem. This could be sine, cosine, tangent, or any other trigonometric function.
Set up the equation using the given information and the appropriate trigonometric function. For example, if you are given an angle and need to find the opposite side, you might use the sine function: \( \sin(\theta) = \frac{\text{opposite}}{\text{hypotenuse}} \).
Rearrange the equation to solve for the unknown variable, in this case, 'a'. This might involve multiplying both sides by the hypotenuse if using the sine function, for example.
Substitute the known values into the equation. This includes the angle measure and any side lengths provided in the problem.
Use a calculator to compute the value of 'a' to the nearest tenth, ensuring your calculator is set to the correct mode (degrees or radians) based on the angle measure given.
Recommended similar problem, with video answer:
 Verified Solution
Verified SolutionThis video solution was recommended by our tutors as helpful for the problem above
Video duration:
7mPlay a video:
Was this helpful?
Key Concepts
Here are the essential concepts you must grasp in order to answer the question correctly.
Trigonometric Ratios
Trigonometric ratios are relationships between the angles and sides of a right triangle. The primary ratios are sine, cosine, and tangent, which relate the angles to the lengths of the opposite, adjacent, and hypotenuse sides. Understanding these ratios is essential for solving problems involving right triangles and for finding unknown side lengths or angles.
Recommended video:

Introduction to Trigonometric Functions
Inverse Trigonometric Functions
Inverse trigonometric functions, such as arcsine, arccosine, and arctangent, are used to determine the angle when the lengths of the sides of a triangle are known. These functions allow us to reverse the process of finding trigonometric ratios, enabling us to solve for angles in various applications. Mastery of these functions is crucial for accurately determining angles in trigonometric problems.
Recommended video:

Introduction to Inverse Trig Functions
Rounding Techniques
Rounding techniques are methods used to simplify numbers to a specified degree of accuracy, often to make calculations easier or results clearer. In this context, rounding to the nearest tenth means adjusting a number to one decimal place, which is important for presenting final answers in a clear and concise manner. Understanding how to round correctly is essential for ensuring precision in mathematical results.
Recommended video:

How to Use a Calculator for Trig Functions

 4:27m
4:27mWatch next
Master Intro to Law of Sines with a bite sized video explanation from Patrick Ford
Start learningRelated Videos
Related Practice