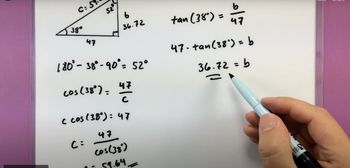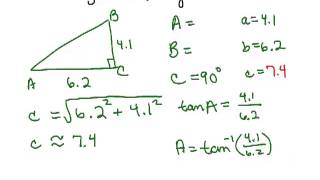Table of contents
- 0. Review of College Algebra4h 43m
- 1. Measuring Angles39m
- 2. Trigonometric Functions on Right Triangles2h 5m
- 3. Unit Circle1h 19m
- 4. Graphing Trigonometric Functions1h 19m
- 5. Inverse Trigonometric Functions and Basic Trigonometric Equations1h 41m
- 6. Trigonometric Identities and More Equations2h 34m
- 7. Non-Right Triangles1h 38m
- 8. Vectors2h 25m
- 9. Polar Equations2h 5m
- 10. Parametric Equations1h 6m
- 11. Graphing Complex Numbers1h 7m
2. Trigonometric Functions on Right Triangles
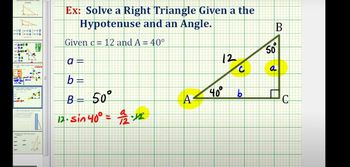
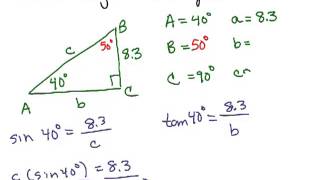
Solving Right Triangles
Problem 14
Textbook Question
Solve each right triangle. When two sides are given, give angles in degrees and minutes. See Examples 1 and 2.
 Verified step by step guidance
Verified step by step guidance1
<Step 1: Identify the given sides of the right triangle. Let's denote them as 'a' and 'b', where 'a' is the length of one leg and 'b' is the length of the other leg.>
<Step 2: Use the Pythagorean theorem to find the hypotenuse 'c'. The formula is: c = \sqrt{a^2 + b^2}.>
<Step 3: Calculate one of the non-right angles using the tangent function. For example, if you want to find angle \( \theta \), use \( \tan(\theta) = \frac{a}{b} \). Then, find \( \theta \) by taking the arctangent: \( \theta = \tan^{-1}(\frac{a}{b}) \).>
<Step 4: Convert the angle \( \theta \) from decimal degrees to degrees and minutes. To do this, take the decimal part of the angle and multiply it by 60 to get the minutes.>
<Step 5: Find the other non-right angle by subtracting \( \theta \) from 90 degrees, since the sum of angles in a triangle is 180 degrees and one angle is 90 degrees.>
Recommended similar problem, with video answer:
 Verified Solution
Verified SolutionThis video solution was recommended by our tutors as helpful for the problem above
Video duration:
4mPlay a video:
Was this helpful?
Key Concepts
Here are the essential concepts you must grasp in order to answer the question correctly.
Right Triangle Properties
A right triangle is defined by one angle measuring 90 degrees. The relationships between the sides and angles are governed by the Pythagorean theorem, which states that the square of the hypotenuse is equal to the sum of the squares of the other two sides. Understanding these properties is essential for solving right triangles, as they provide the foundational relationships needed to find unknown angles and lengths.
Recommended video:

30-60-90 Triangles
Trigonometric Ratios
Trigonometric ratios, including sine, cosine, and tangent, relate the angles of a triangle to the lengths of its sides. For a right triangle, sine is the ratio of the opposite side to the hypotenuse, cosine is the ratio of the adjacent side to the hypotenuse, and tangent is the ratio of the opposite side to the adjacent side. These ratios are crucial for calculating unknown angles when two sides are known.
Recommended video:

Introduction to Trigonometric Functions
Angle Measurement in Degrees and Minutes
Angles can be measured in degrees, where one full rotation is 360 degrees, and in minutes, where one degree is divided into 60 minutes. This method of measurement is particularly useful in trigonometry for providing precise angle values. When solving triangles, it is important to convert between these units as needed, especially when presenting answers in degrees and minutes.
Recommended video:

Reference Angles on the Unit Circle

 4:18m
4:18mWatch next
Master Finding Missing Side Lengths with a bite sized video explanation from Nick Kaneko
Start learningRelated Videos
Related Practice