Here are the essential concepts you must grasp in order to answer the question correctly.
Distributive Property
The distributive property states that a(b + c) = ab + ac. It allows us to multiply a single term by each term within a parenthesis. This property is essential for simplifying expressions and solving equations, as it helps to eliminate parentheses and combine like terms effectively.
Recommended video:
Imaginary Roots with the Square Root Property
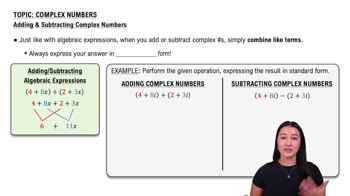
Combining Like Terms
Combining like terms involves adding or subtracting terms that have the same variable raised to the same power. This process simplifies expressions by consolidating similar components, making it easier to work with and understand the overall expression. For example, in the expression x + x, both terms are like terms and can be combined.
Recommended video:
Adding and Subtracting Complex Numbers
Simplification of Expressions
Simplification of expressions refers to the process of reducing an expression to its simplest form. This often involves using the distributive property, combining like terms, and eliminating unnecessary parentheses. The goal is to make the expression easier to interpret and work with, which is crucial in algebra and trigonometry.
Recommended video:
Simplifying Trig Expressions
 Verified Solution
Verified Solution


 7:48m
7:48m
