Here are the essential concepts you must grasp in order to answer the question correctly.
Trigonometric Functions
Trigonometric functions, such as sine, cosine, and tangent, relate angles to ratios of sides in right triangles. The sine function, specifically, represents the ratio of the length of the opposite side to the hypotenuse. Understanding these functions is crucial for solving equations involving angles, as they provide the foundational relationships needed to manipulate and solve trigonometric equations.
Recommended video:
Introduction to Trigonometric Functions
Algebraic Manipulation
Algebraic manipulation involves rearranging and simplifying equations to isolate variables. In the context of the given equation, this means moving terms around to express one side in terms of the other. Mastery of algebraic techniques, such as factoring and combining like terms, is essential for effectively solving trigonometric equations and finding all possible solutions.
Recommended video:
Algebraic Operations on Vectors
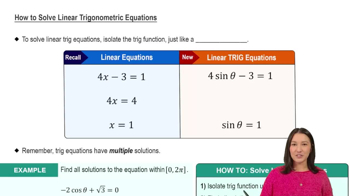
General Solutions of Trigonometric Equations
Trigonometric equations often have multiple solutions due to the periodic nature of trigonometric functions. For example, the sine function has a period of 2π, meaning that if θ is a solution, then θ + 2kπ (where k is any integer) is also a solution. Understanding how to derive and express these general solutions is key to fully solving trigonometric equations and identifying all possible angles that satisfy the equation.
Recommended video:
How to Solve Linear Trigonometric Equations
 Verified step by step guidance
Verified step by step guidance Verified Solution
Verified Solution


 4:25m
4:25m