In Exercises 23–34, find the exact value of each of the remaining trigonometric functions of θ. sin θ = 5/13, θ in quadrant II

Verified step by step guidance

1. <insert step 1: Recall that in quadrant II, sine is positive and cosine is negative.>
2. <insert step 2: Use the Pythagorean identity: \( \sin^2 \theta + \cos^2 \theta = 1 \).>
3. <insert step 3: Substitute \( \sin \theta = \frac{5}{13} \) into the identity: \( \left(\frac{5}{13}\right)^2 + \cos^2 \theta = 1 \).>
4. <insert step 4: Solve for \( \cos^2 \theta \) and then find \( \cos \theta \), remembering it is negative in quadrant II.>
5. <insert step 5: Use the values of \( \sin \theta \) and \( \cos \theta \) to find the other trigonometric functions: \( \tan \theta = \frac{\sin \theta}{\cos \theta} \), \( \csc \theta = \frac{1}{\sin \theta} \), \( \sec \theta = \frac{1}{\cos \theta} \), and \( \cot \theta = \frac{1}{\tan \theta} \).>

Key Concepts

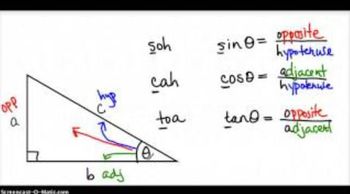
Trigonometric Functions

Trigonometric functions relate the angles of a triangle to the lengths of its sides. The primary functions include sine (sin), cosine (cos), and tangent (tan), along with their reciprocals: cosecant (csc), secant (sec), and cotangent (cot). Understanding these functions is essential for solving problems involving angles and side lengths in right triangles.

Pythagorean Identity

The Pythagorean identity states that for any angle θ, the relationship sin²(θ) + cos²(θ) = 1 holds true. This identity is crucial for finding the values of other trigonometric functions when one function is known. In this case, knowing sin(θ) allows us to calculate cos(θ) and subsequently the other trigonometric functions.

Quadrants and Signs of Trigonometric Functions

The unit circle is divided into four quadrants, each affecting the signs of the trigonometric functions. In quadrant II, sine is positive while cosine and tangent are negative. Recognizing the quadrant in which the angle lies helps determine the signs of the trigonometric functions, which is vital for accurately calculating their values.