Here are the essential concepts you must grasp in order to answer the question correctly.
Factoring Polynomials
Factoring polynomials involves expressing a polynomial as a product of its simpler components, or factors. This process is essential for simplifying expressions and solving equations. Common methods include factoring out the greatest common factor, using special products like the difference of squares, and applying the quadratic formula for polynomials of degree two.
Recommended video:
Difference of Squares
The difference of squares is a specific factoring technique applicable to expressions of the form a² - b², which can be factored into (a + b)(a - b). This concept is crucial for the given polynomial, as 25s⁴ - 9t² can be recognized as a difference of squares, where a = 5s² and b = 3t. Understanding this allows for efficient factorization.
Recommended video:
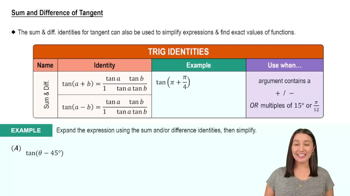
Sum and Difference of Tangent
Degree of a Polynomial
The degree of a polynomial is the highest power of the variable in the expression. In the polynomial 25s⁴ - 9t², the degree is determined by the term with the highest exponent, which is 4 from 25s⁴. Recognizing the degree helps in understanding the polynomial's behavior and the methods suitable for its factorization.
Recommended video:
Converting between Degrees & Radians
 Verified step by step guidance
Verified step by step guidance Verified Solution
Verified Solution


 7:48m
7:48m