Table of contents
- 0. Review of College Algebra4h 43m
- 1. Measuring Angles39m
- 2. Trigonometric Functions on Right Triangles2h 5m
- 3. Unit Circle1h 19m
- 4. Graphing Trigonometric Functions1h 19m
- 5. Inverse Trigonometric Functions and Basic Trigonometric Equations1h 41m
- 6. Trigonometric Identities and More Equations2h 34m
- 7. Non-Right Triangles1h 38m
- 8. Vectors2h 25m
- 9. Polar Equations2h 5m
- 10. Parametric Equations1h 6m
- 11. Graphing Complex Numbers1h 7m
1. Measuring Angles
Angles in Standard Position
Problem 25a
Textbook Question
Find the measure of each marked angle. See Example 2.
 Verified step by step guidance
Verified step by step guidance1
Identify the given angles and any relationships between them, such as complementary, supplementary, or vertical angles.
Use the properties of the angles to set up equations. For example, if two angles are complementary, their sum is 90 degrees; if they are supplementary, their sum is 180 degrees.
Solve the equations to find the measures of the unknown angles. This may involve algebraic manipulation such as adding, subtracting, or dividing both sides of the equation.
Check your solutions by substituting the values back into the original relationships to ensure they satisfy the conditions given in the problem.
If applicable, use trigonometric identities or ratios to find any additional angle measures or to verify your solutions.
Recommended similar problem, with video answer:
 Verified Solution
Verified SolutionThis video solution was recommended by our tutors as helpful for the problem above
Video duration:
2mPlay a video:
Was this helpful?
Key Concepts
Here are the essential concepts you must grasp in order to answer the question correctly.
Angle Relationships
Understanding angle relationships is crucial in trigonometry, as it involves recognizing how angles interact within geometric figures. This includes concepts such as complementary angles (summing to 90 degrees), supplementary angles (summing to 180 degrees), and vertical angles (which are equal). These relationships help in setting up equations to find unknown angle measures.
Recommended video:
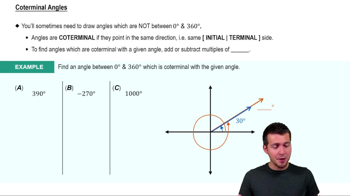
Coterminal Angles
Trigonometric Ratios
Trigonometric ratios relate the angles of a triangle to the lengths of its sides. The primary ratios—sine, cosine, and tangent—are defined as the ratios of the lengths of the sides of a right triangle. For example, sine is the ratio of the opposite side to the hypotenuse. These ratios are essential for solving problems involving angles and can be used to find unknown angles when side lengths are known.
Recommended video:

Introduction to Trigonometric Functions
Geometric Constructions
Geometric constructions involve creating figures using a compass and straightedge, which can help visualize and solve problems related to angles. Understanding how to construct angles and triangles accurately is vital for determining angle measures. This skill is often applied in conjunction with angle relationships and trigonometric ratios to find the measures of marked angles in various geometric contexts.
Recommended video:

Adding Vectors Geometrically

 5:50m
5:50mWatch next
Master Drawing Angles in Standard Position with a bite sized video explanation from Patrick Ford
Start learningRelated Videos
Related Practice