Table of contents
- 0. Review of College Algebra4h 43m
- 1. Measuring Angles39m
- 2. Trigonometric Functions on Right Triangles2h 5m
- 3. Unit Circle1h 19m
- 4. Graphing Trigonometric Functions1h 19m
- 5. Inverse Trigonometric Functions and Basic Trigonometric Equations1h 41m
- 6. Trigonometric Identities and More Equations2h 34m
- 7. Non-Right Triangles1h 38m
- 8. Vectors2h 25m
- 9. Polar Equations2h 5m
- 10. Parametric Equations1h 6m
- 11. Graphing Complex Numbers1h 7m
6. Trigonometric Identities and More Equations
Introduction to Trigonometric Identities
Problem 5.80b
Textbook Question
Verify that each equation is an identity.
(1 + sin x + cos x)² = 2(1 + sin x) (1 + cos x)
 Verified step by step guidance
Verified step by step guidance1
Start by expanding the left side of the equation: \((1 + \sin x + \cos x)^2\). Use the formula \((a + b + c)^2 = a^2 + b^2 + c^2 + 2ab + 2ac + 2bc\).
Calculate each term: \(a^2 = 1^2 = 1\), \(b^2 = (\sin x)^2 = \sin^2 x\), \(c^2 = (\cos x)^2 = \cos^2 x\), \(2ab = 2 \cdot 1 \cdot \sin x = 2\sin x\), \(2ac = 2 \cdot 1 \cdot \cos x = 2\cos x\), \(2bc = 2 \cdot \sin x \cdot \cos x = 2\sin x \cos x\).
Combine these terms to get: \(1 + \sin^2 x + \cos^2 x + 2\sin x + 2\cos x + 2\sin x \cos x\).
Use the Pythagorean identity \(\sin^2 x + \cos^2 x = 1\) to simplify: \(1 + 1 + 2\sin x + 2\cos x + 2\sin x \cos x = 2 + 2\sin x + 2\cos x + 2\sin x \cos x\).
Now expand the right side: \(2(1 + \sin x)(1 + \cos x) = 2((1 + \sin x) + (1 + \sin x)\cos x) = 2(1 + \sin x + \cos x + \sin x \cos x)\). Simplify to see if both sides are equal.
Recommended similar problem, with video answer:
 Verified Solution
Verified SolutionThis video solution was recommended by our tutors as helpful for the problem above
Video duration:
0m:0sPlay a video:
Was this helpful?
Key Concepts
Here are the essential concepts you must grasp in order to answer the question correctly.
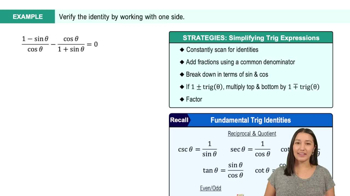
Trigonometric Identities
Trigonometric identities are equations that hold true for all values of the variable within a certain domain. Common identities include the Pythagorean identities, reciprocal identities, and angle sum/difference identities. Understanding these identities is crucial for simplifying trigonometric expressions and verifying equations.
Recommended video:

Fundamental Trigonometric Identities
Algebraic Expansion
Algebraic expansion involves applying the distributive property to multiply expressions, such as binomials. In the context of the given equation, expanding (1 + sin x + cos x)² requires using the formula (a + b)² = a² + 2ab + b², which helps in simplifying the left-hand side of the equation for comparison with the right-hand side.
Recommended video:

Algebraic Operations on Vectors
Factoring
Factoring is the process of breaking down an expression into simpler components, or factors, that can be multiplied to obtain the original expression. In the context of verifying identities, recognizing common factors on both sides of the equation can simplify the verification process and help establish equality between the two sides.
Recommended video:

Factoring

 6:19m
6:19mWatch next
Master Even and Odd Identities with a bite sized video explanation from Callie Rethman
Start learningRelated Videos
Related Practice