Table of contents
- 0. Review of College Algebra4h 43m
- 1. Measuring Angles39m
- 2. Trigonometric Functions on Right Triangles2h 5m
- 3. Unit Circle1h 19m
- 4. Graphing Trigonometric Functions1h 19m
- 5. Inverse Trigonometric Functions and Basic Trigonometric Equations1h 41m
- 6. Trigonometric Identities and More Equations2h 34m
- 7. Non-Right Triangles1h 38m
- 8. Vectors2h 25m
- 9. Polar Equations2h 5m
- 10. Parametric Equations1h 6m
- 11. Graphing Complex Numbers1h 7m
3. Unit Circle
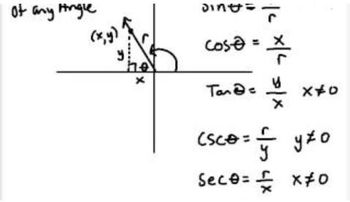
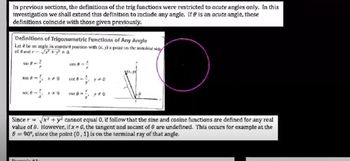
Trigonometric Functions on the Unit Circle
Problem 78
Textbook Question
Find a formula for the area of each figure in terms of s.
 Verified step by step guidance
Verified step by step guidance1
<insert step 1: Identify the type of figure you are dealing with. For example, it could be a square, triangle, or circle.>
<insert step 2: Recall the general formula for the area of the identified figure. For instance, for a square, the area is \( s^2 \), for a triangle, it is \( \frac{1}{2} \times \text{base} \times \text{height} \), and for a circle, it is \( \pi s^2 \) where \( s \) is the radius.>
<insert step 3: Substitute the given variable \( s \) into the general formula. For example, if the figure is a square, the area formula becomes \( s^2 \).>
<insert step 4: Simplify the expression if necessary. For example, if the figure is a triangle and \( s \) represents both the base and the height, the area formula becomes \( \frac{1}{2} s^2 \).>
<insert step 5: Verify that the formula is dimensionally consistent and makes sense for the given figure.>
Recommended similar problem, with video answer:
 Verified Solution
Verified SolutionThis video solution was recommended by our tutors as helpful for the problem above
Video duration:
3mPlay a video:
Was this helpful?
Key Concepts
Here are the essential concepts you must grasp in order to answer the question correctly.
Area of a Triangle
The area of a triangle can be calculated using the formula A = 1/2 * base * height. However, when the lengths of the sides are known, Heron's formula can be used, which states that the area A is given by A = √(s(s-a)(s-b)(s-c)), where s is the semi-perimeter and a, b, c are the lengths of the sides.
Recommended video:

Calculating Area of SAS Triangles
Semi-Perimeter
The semi-perimeter of a triangle is defined as half of the perimeter. It is calculated as s = (a + b + c) / 2, where a, b, and c are the lengths of the triangle's sides. The semi-perimeter is crucial in Heron's formula for calculating the area of a triangle when only the side lengths are known.
Recommended video:

Equations with Trigonometric Functions Example 3
Heron's Formula
Heron's formula provides a way to calculate the area of a triangle when the lengths of all three sides are known. It utilizes the semi-perimeter and is expressed as A = √(s(s-a)(s-b)(s-c)). This formula is particularly useful in trigonometry for finding areas without needing to know the height or angles of the triangle.
Recommended video:

Quadratic Formula

 6:34m
6:34mWatch next
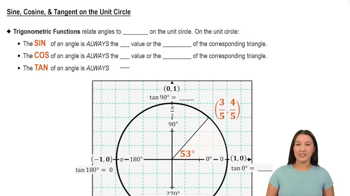
Master Sine, Cosine, & Tangent on the Unit Circle with a bite sized video explanation from Callie Rethman
Start learning