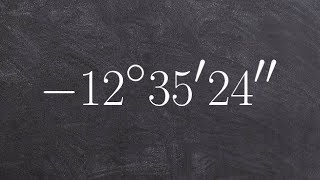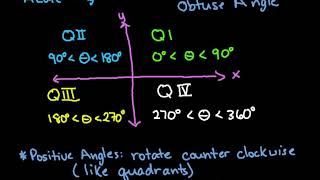Table of contents
- 0. Review of College Algebra4h 43m
- 1. Measuring Angles39m
- 2. Trigonometric Functions on Right Triangles2h 5m
- 3. Unit Circle1h 19m
- 4. Graphing Trigonometric Functions1h 19m
- 5. Inverse Trigonometric Functions and Basic Trigonometric Equations1h 41m
- 6. Trigonometric Identities and More Equations2h 34m
- 7. Non-Right Triangles1h 38m
- 8. Vectors2h 25m
- 9. Polar Equations2h 5m
- 10. Parametric Equations1h 6m
- 11. Graphing Complex Numbers1h 7m
1. Measuring Angles
Angles in Standard Position
Problem 117
Textbook Question
Concept Check Sketch each angle in standard position. Draw an arrow representing the correct amount of rotation. Find the measure of two other angles, one positive and one negative, that are coterminal with the given angle. Give the quadrant of each angle, if applicable. ―61 °
 Verified step by step guidance
Verified step by step guidance1
Step 1: Begin by sketching the angle -61° in standard position. Start from the positive x-axis and rotate clockwise since the angle is negative.
Step 2: Draw an arrow to represent the rotation of -61° from the positive x-axis. This angle will end in the fourth quadrant.
Step 3: To find a positive coterminal angle, add 360° to -61°. This will give you an angle that is coterminal and positive.
Step 4: To find a negative coterminal angle, subtract 360° from -61°. This will give you another angle that is coterminal and negative.
Step 5: Identify the quadrant for each angle: -61° is in the fourth quadrant, the positive coterminal angle will also be in the fourth quadrant, and the negative coterminal angle will be in the same quadrant as -61°.
Recommended similar problem, with video answer:
 Verified Solution
Verified SolutionThis video solution was recommended by our tutors as helpful for the problem above
Video duration:
2mPlay a video:
Was this helpful?
Key Concepts
Here are the essential concepts you must grasp in order to answer the question correctly.
Standard Position of an Angle
An angle is in standard position when its vertex is at the origin of a coordinate system and its initial side lies along the positive x-axis. The angle is measured from the initial side to the terminal side, with positive angles measured counterclockwise and negative angles measured clockwise. Understanding this concept is crucial for sketching angles accurately.
Recommended video:

Drawing Angles in Standard Position
Coterminal Angles
Coterminal angles are angles that share the same terminal side when drawn in standard position. To find coterminal angles, you can add or subtract multiples of 360 degrees (or 2π radians). For example, for an angle of -61°, adding 360° gives a positive coterminal angle of 299°.
Recommended video:
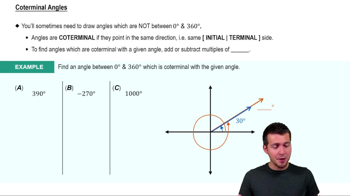
Coterminal Angles
Quadrants of the Coordinate Plane
The coordinate plane is divided into four quadrants, each defined by the signs of the x and y coordinates. Quadrant I has both coordinates positive, Quadrant II has a negative x and positive y, Quadrant III has both negative, and Quadrant IV has a positive x and negative y. Identifying the quadrant of an angle helps in understanding its position relative to the axes.
Recommended video:

Quadratic Formula

 5:50m
5:50mWatch next
Master Drawing Angles in Standard Position with a bite sized video explanation from Patrick Ford
Start learningRelated Videos
Related Practice