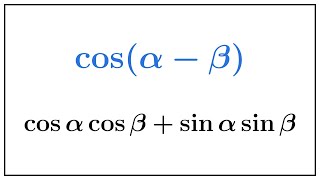Table of contents
- 0. Review of College Algebra4h 43m
- 1. Measuring Angles39m
- 2. Trigonometric Functions on Right Triangles2h 5m
- 3. Unit Circle1h 19m
- 4. Graphing Trigonometric Functions1h 19m
- 5. Inverse Trigonometric Functions and Basic Trigonometric Equations1h 41m
- 6. Trigonometric Identities and More Equations2h 34m
- 7. Non-Right Triangles1h 38m
- 8. Vectors2h 25m
- 9. Polar Equations2h 5m
- 10. Parametric Equations1h 6m
- 11. Graphing Complex Numbers1h 7m
6. Trigonometric Identities and More Equations
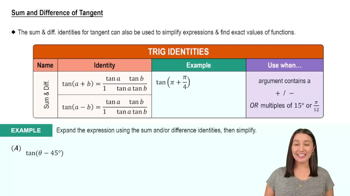
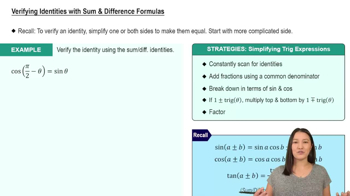
Sum and Difference Identities
Problem 5.24b
Textbook Question
Find the exact value of each expression.
sin (-13π/12)
 Verified step by step guidance
Verified step by step guidance1
<Step 1: Recognize the angle transformation> Start by recognizing that \(-\frac{13\pi}{12}\) is an angle in radians. Since it is negative, it indicates a clockwise rotation from the positive x-axis. To simplify, convert this angle to a positive angle by adding \(2\pi\) (a full circle) to it: \(-\frac{13\pi}{12} + 2\pi\).
<Step 2: Simplify the angle> Simplify the expression from Step 1 to find the equivalent positive angle. This will help in identifying the reference angle and the quadrant in which the angle lies.
<Step 3: Determine the reference angle> Once you have the positive angle, determine the reference angle. The reference angle is the acute angle that the terminal side of the given angle makes with the x-axis.
<Step 4: Identify the quadrant> Determine the quadrant in which the angle \(-\frac{13\pi}{12}\) lies. This is important because the sign of the sine function depends on the quadrant.
<Step 5: Use the reference angle to find the sine> Use the reference angle and the quadrant information to find the sine of the original angle. Remember that the sine function is positive in the first and second quadrants and negative in the third and fourth quadrants.
Recommended similar problem, with video answer:
 Verified Solution
Verified SolutionThis video solution was recommended by our tutors as helpful for the problem above
Video duration:
0m:0sPlay a video:
Was this helpful?
Key Concepts
Here are the essential concepts you must grasp in order to answer the question correctly.
Unit Circle
The unit circle is a circle with a radius of one centered at the origin of a coordinate plane. It is fundamental in trigonometry as it provides a geometric interpretation of the sine, cosine, and tangent functions. Angles measured in radians correspond to points on the unit circle, where the x-coordinate represents cosine and the y-coordinate represents sine.
Recommended video:

Introduction to the Unit Circle
Reference Angles
A reference angle is the acute angle formed by the terminal side of a given angle and the x-axis. It is used to simplify the calculation of trigonometric functions for angles greater than 90 degrees or negative angles. For example, to find sin(-13π/12), we can determine its reference angle by adding 2π until the angle is positive and then finding the sine of the corresponding acute angle.
Recommended video:

Reference Angles on the Unit Circle
Sine Function Properties
The sine function is periodic with a period of 2π, meaning sin(θ) = sin(θ + 2kπ) for any integer k. Additionally, sine is an odd function, which implies that sin(-θ) = -sin(θ). These properties allow us to evaluate sine for negative angles and angles outside the standard range, facilitating the calculation of sin(-13π/12) by using its positive equivalent.
Recommended video:

Graph of Sine and Cosine Function

 6:14m
6:14mWatch next
Master Sum and Difference of Sine & Cosine with a bite sized video explanation from Callie Rethman
Start learningRelated Videos
Related Practice