Hey everyone. We just learned about the unit circle, and we saw that every angle corresponds to a specific x, y coordinate on that unit circle. Like our angle of 0 degrees corresponds to the point 1,0. But how exactly are these two things related mathematically? Well, they're related using trigonometric functions, which specifically relate angles to points on our unit circle. Now you may have worked with these trig functions, sine, cosine, and tangent before using right triangles. So it may seem really strange here that we're now working with these trig functions using a circle, but it's actually the exact same thing because we can think of all of these angles as forming a right triangle. And it's actually going to be even easier to find trig values of an angle because they're just going to be equal to the x and y coordinates on our unit circle. And I'm going to show you exactly how that works here. So let's go ahead and get started. Now looking at our angle of 53 degrees here, I see that it corresponds to this point, 3545 on that unit circle. Now if I trace my x and my y values here, I have formed a right triangle. And this right triangle has a hypotenuse of 1 because that's the radius of the unit circle. Then it has a base of my x value and a height of that y value that we see in this coordinate right here. Now looking at this triangle, if I asked you to find the sine of 53 degrees and the cosine of 53 degrees using this triangle, you would actually find that the sine is just equal to that y value or the height of our triangle and the cosine is going to be equal to the x value or the base of our triangle. And this is actually how it's going to work for every single angle on our unit circle. The sine of an angle is always the y value or the height of our corresponding triangle, and the cosine of an angle is always the x value or the base of our corresponding triangle. Now you're not going to want to have to set up a triangle and work this out algebraically every single time. So I like to remember this by just looking at my ordered pair. Now we know that ordered pairs go in the order x, y. And I like to think about my trig functions as going in alphabetical order. I know that c comes before s in the alphabet, so cosine comes before sine here. My cosine is my x value and my sine is my y value. Now like I said, this works for any angle on our unit circle, so let's come down to our 0 degree angle measure here. Now, this can be kind of strange to think about as a triangle, but think about your triangle as being squished against that x axis to give it a height of 0. Now when looking at these values here, 1 and 0, I know that the cosine of 0 degrees is equal to 1 and the sine of 0 degrees is equal to 0, which makes sense because the height of my triangle is 0 here. Now we don't want to forget about tangent because we still need to find the value of our tangent. Now you might have calculated the tangent previously by dividing sine over cosine. But since our sine and our cosine correspond to our y and x values here, we can find the tangent of an angle by simply dividing y over x. So coming back over here to the tangent of 0 degrees, if I take my y value of 0 and divide it by my x value of 1, I end up getting that the tangent of 0 degrees is simply 0. Now let's go ahead and look at the tangent of our other 3 quadrantal angles on our unit circle starting with 90 degrees. So the tangent of 90 degrees, if I take my y value of 1 divided by my x value of 0, I end up with a fraction dividing by 0 which is not something that we can do. So this is actually an undefined value. Now if I come over here to 180 degrees, I will end up with 0 on the top of my fraction the same way I did with 0 degrees. So the tangent of 180 degrees is also equal to 0. Now let's come down here to the tangent of 270 degrees. We will end up dividing by 0 the way we did with 90 degrees. So this is again an undefined value. But these are not the only 4 points on our unit circle, right? We know that we have all of these other points actually forming the rest of our circle. So let's go ahead and take a look at the trig values of some other points along this circle. Now looking at 217 degrees here, first, I come down to this third quadrant looking at my angle of 217 degrees. Now we can go ahead and draw our triangle here in order to visualize this a little bit more. And then looking at this ordered pair, I remember, okay, x y c s, telling me which is my cosine and which is my sine. So looking at the sine of 217 degrees, I know that that's my y value. So the sine of 217 degrees is -35. Now it may seem strange here to have a negative value for a trig function, but this is totally fine because looking at the triangle that is on this graph, we see that we are in the negative y values because this is attached to a coordinate system, right? So it's totally fine to have a negative trig value. Now our cosine here is our x value. Remember negative 4 5ths which is also a negative value which makes sense looking at the triangle on our graph here. And then finally we have the tangent of 217 degrees. Now remember for the tangent of our angle we want to take our y value and divide it by our x value. So here we would take negative three-fifths and divide it by negative four-fifths. Now whenever we divide fractions, we're just really multiplying by the reciprocal, so this would be -35×-54. Now those fives are going to cancel, and the negatives will cancel as well, leaving me with an answer of 34. Now looking at this, you may have noticed that because these have the exact same denominator, we have effectively just divided their numerators. So you can think about that as kind of a shortcut here as we continue to work with dividing fractions. So this tells me the tangent of 217 degrees is 34. Now let's move on to our final example here. We have an angle measure of 60 degrees in that first quadrant. Now looking at my 60 degree angle, I can go ahead and draw that triangle if it makes you happy, and then we can go ahead and identify our trig values. Now remember looking at our ordered pair x, y, c, s, my cosine value is that 1/2 that I can go ahead and fill in and then my sine value is that y3/2. Now for the tangent, we again take y over x. And looking at these numbers, they again have the same exact denominator of 2. So effectively we're just going to be dividing their numerators. So if I take the numerator of each of them, 3/1, that just gives me a value of the square root of 3, and I'm done here. The tangent of 60 degrees is the square root of 3. Now that we've seen how trig values relate angles to their ordered pair on the unit circle, let's get a bit more practice together. Thanks for watching, and I'll see you in the next one.
- 0. Fundamental Concepts of Algebra3h 29m
- 1. Equations and Inequalities3h 27m
- 2. Graphs1h 43m
- 3. Functions & Graphs2h 17m
- 4. Polynomial Functions1h 54m
- 5. Rational Functions1h 23m
- 6. Exponential and Logarithmic Functions2h 28m
- 7. Measuring Angles39m
- 8. Trigonometric Functions on Right Triangles2h 5m
- 9. Unit Circle1h 19m
- 10. Graphing Trigonometric Functions1h 19m
- 11. Inverse Trigonometric Functions and Basic Trig Equations1h 41m
- 12. Trigonometric Identities 2h 34m
- 13. Non-Right Triangles1h 38m
- 14. Vectors2h 25m
- 15. Polar Equations2h 5m
- 16. Parametric Equations1h 6m
- 17. Graphing Complex Numbers1h 7m
- 18. Systems of Equations and Matrices1h 6m
- 19. Conic Sections2h 36m
- 20. Sequences, Series & Induction1h 15m
- 21. Combinatorics and Probability1h 45m
- 22. Limits & Continuity1h 49m
- 23. Intro to Derivatives & Area Under the Curve2h 9m
Trigonometric Functions on the Unit Circle: Study with Video Lessons, Practice Problems & Examples
 Created using AI
Created using AIThe unit circle connects angles to coordinates using trigonometric functions: sine, cosine, and tangent. For any angle, sine corresponds to the y-coordinate and cosine to the x-coordinate. Tangent is calculated as the ratio of sine to cosine (y/x). For example, at 53 degrees, sine is 4/5 and cosine is 3/5, leading to a tangent of 4/3. Understanding these relationships simplifies finding trig values across all quadrants, including negative values in the third quadrant, reinforcing the concept of angles as right triangles within the unit circle.
Sine, Cosine, & Tangent on the Unit Circle
Video transcript
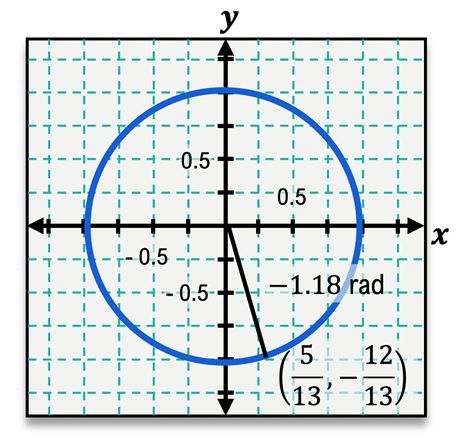
Find the sine, cosine, and tangent of each angle using the unit circle.
θ=−1.18 rad, (135,−1312)
sinθ=−1312,cosθ=135,tanθ=512
sinθ=−1312,cosθ=135,tanθ=−512
sinθ=1312,cosθ=135,tanθ=125
sinθ=135,cosθ=13−12,tanθ=125
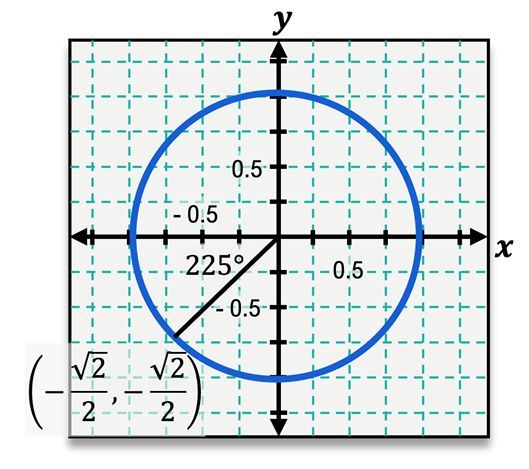
Find the sine, cosine, and tangent of each angle using the unit circle.
θ=225°,(−22,−22)
sinθ=−22,cosθ=−22,tanθ=2
sinθ=22,cosθ=−22,tanθ=−1
sinθ=−22,cosθ=−22,tanθ=1
sinθ=22,cosθ=22,tanθ=12
Here’s what students ask on this topic:
What are the sine and cosine values for common angles on the unit circle?
On the unit circle, common angles and their sine and cosine values are as follows:
- 0°: cos(0°) = 1, sin(0°) = 0
- 30°: cos(30°) = √3/2, sin(30°) = 1/2
- 45°: cos(45°) = √2/2, sin(45°) = √2/2
- 60°: cos(60°) = 1/2, sin(60°) = √3/2
- 90°: cos(90°) = 0, sin(90°) = 1
These values are derived from the coordinates of points on the unit circle corresponding to these angles.
 Created using AI
Created using AIHow do you find the tangent of an angle using the unit circle?
To find the tangent of an angle using the unit circle, you divide the sine value by the cosine value of that angle. Mathematically, this is expressed as:
For example, for 45°, the sine and cosine values are both √2/2. Therefore, the tangent is:
 Created using AI
Created using AIWhy are some trigonometric function values negative on the unit circle?
Trigonometric function values can be negative on the unit circle because the coordinates (x, y) can be negative depending on the quadrant. For example, in the third quadrant, both x and y are negative, making sine and cosine values negative. This reflects the coordinate system where angles are measured, and the signs of the coordinates change based on the quadrant.
 Created using AI
Created using AIHow do you use the unit circle to find the sine and cosine of 217 degrees?
To find the sine and cosine of 217 degrees using the unit circle, locate the angle in the third quadrant. The coordinates for 217 degrees are approximately (-4/5, -3/5). Therefore, the sine of 217 degrees is:
And the cosine of 217 degrees is:
 Created using AI
Created using AIWhat is the relationship between the unit circle and right triangles?
The unit circle and right triangles are related because any angle on the unit circle can be represented as a right triangle. The hypotenuse of this triangle is the radius of the unit circle (which is 1), and the legs are the x and y coordinates of the point on the circle. The sine of the angle is the y-coordinate (opposite side), and the cosine is the x-coordinate (adjacent side).
 Created using AI
Created using AI