Classify the triangle below according to its sides and angles.

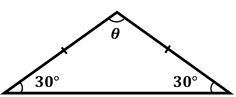
I. Equilateral
II. Isosceles
III. Scalene
IV. Acute
V. Obtuse
VI. Right

• A. I and IV
• B. I and V
• C. II and V
• D. II and IV
• E. III and VI
• F. Only one of I, II, III, IV, V, and V

Answer: (C)

Verified step by step guidance

1. Observe the triangle and note that it has two equal sides, indicated by the hash marks. This suggests that the triangle is isosceles.
2. Look at the angles provided: two angles are 30 degrees each. In a triangle, the sum of all angles is always 180 degrees.
3. Calculate the third angle, \( \theta \), by using the equation: \( 30^\circ + 30^\circ + \theta = 180^\circ \). Solve for \( \theta \).
4. After solving, you find that \( \theta = 120^\circ \). Since one angle is greater than 90 degrees, the triangle is classified as obtuse.
5. Based on the side and angle classifications, the triangle is isosceles (II) and obtuse (V).