Here are the essential concepts you must grasp in order to answer the question correctly.
Power
Power is the rate at which work is done or energy is transferred over time. In physics, it is calculated as the work done divided by the time taken. The unit of power is the watt (W), where 1 watt equals 1 joule per second. Understanding power is essential for determining how much energy the tractor expends to move the bale up the incline.
Recommended video:
Friction
Friction is the force that opposes the relative motion of two surfaces in contact. The coefficient of kinetic friction quantifies this force when objects are sliding against each other. In this scenario, the frictional force acting on the bale must be calculated to determine the total force the tractor needs to exert to overcome it and move the bale up the incline.
Recommended video:
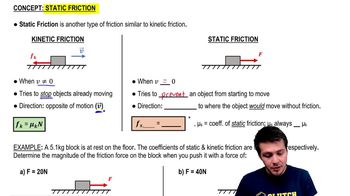
Static Friction & Equilibrium
Inclined Plane
An inclined plane is a flat surface tilted at an angle to the horizontal, which allows for the easier movement of objects. The angle of the incline affects the gravitational force component acting along the plane, which influences the net force required to move the object. In this problem, the 15° incline impacts both the gravitational force acting on the bale and the work done by the tractor.
Recommended video: