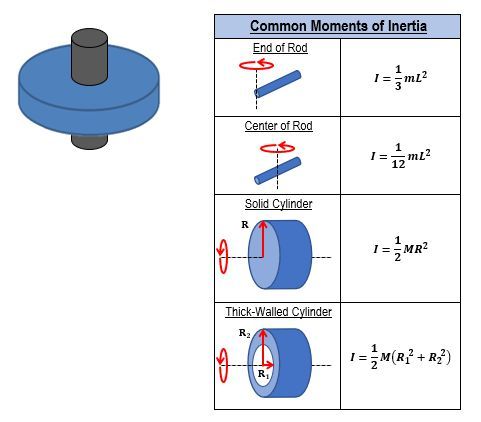
Hey, guys. So now that we've seen how to solve some basic torque acceleration questions, we're going to add motion equations into the mix which will generate a bunch of extra questions, problems we can solve. Let's check it out. So you may remember that when we had force problems, most force problems were solved using f=ma. You may remember that some of those problems would also involve our 3 or 4 equations of motion or kinematics equations. The same thing is going to happen here with rotation, where some torque problems will require both τ=Iα, which is the rotational version of Newton's second law, and rotational motion equations. Motion equations are UAM equations, uniformly accelerated motion, kinematics equations, motion equations, whatever you call it. We got 3 to 4 of these guys. So remember, just like how it was with linear motion, the variable that will connect τ=Iα to the rotational motion equations, which are these guys here, is going to be acceleration. Okay. Acceleration. Now because we're talking about rotation, this means α. Right? Notice how there's an α here and there's an α here, here and here. Okay. So we got more equations and more variables, but it's not harder. It's just more stuff. So let's check out this example here. I have a solid sphere and I give you the mass and the diameter. Solid sphere is the shape of the sphere. So I know that because it's a solid sphere, I am going to use the moment of inertia of a solid sphere, which is 25mr2. I got the mass. The mass is 200. And I have the diameter. Remember, in physics, you're never going to use diameter. So, as soon as I see diameter, I convert that immediately into radius which is half of that. So it's 3 meters. And it spins about an axis through its center. This is the regular rotation of a sphere, a solid sphere which is around itself. Okay. It says it does this with 180 RPM clockwise. So the RPM is 180. What's up with clockwise? Well, clockwise is going to mean that it is negative. So it's got an RPM of 180. Now remember, as we did in motion problems, rotational motion problems, whenever you're given RPM, a vast majority of the time, you're going to immediately convert that into omega because most of our equations have omega, little w, but not RPM in it. Right? So the first thing I'm gonna do here, or the next thing I'm gonna do here is convert this into w. So w=ω=2πf or 2π Remember, f frequency is, RPM over 60. K. So this is going to be 2πnegative 18060. This is going to be 3 right there, which means the whole thing will be negative 6π radians per second radians per second. Cool. So I got that. That's the initial speed. I wanna know how much torque is needed to stop this thing in just 10 seconds. So I'm asking what is the torque to stop it. Right. So that means that this is my initial omega and I want to have a final omega of 0. And I want to do this in just 10 seconds. So ∆t=10. So I hope you notice here, you start seeing all these motion variables. And remember, the way I solve motion problems is by setting up the curly braces and putting all 5 motion variables there. So let's do that. Omega initial equals, negative 6π. Omega final equals, we want it to be 0 alpha ∆θ and delta t. Now the t is 10, and these 2 guys, we don't have them. K. And they're also not what we're looking for. But since I saw all these variables, I decided, hey, let's start setting this up because I know this is coming. But really, what we're looking for is, is torque. So you might actually have started this question, instead of gone here. You might have just written that the sum of all torques =Iα, and that's perfectly fine as well. That's if anything, that's a more directed way to the answer. Right? More targeted which is fine. There's only, one torque here. We're assuming there's one torque. This thing is spinning and I guess you're applying a torque to it, to make it stop. So you can assume that there's only one torque. So the sum of all torques will become just the torque that you're looking for. And that is Iα. Okay. So if I can have I and I have α, I'm done and that will be my answer. So let's expand I=25,mr2 and α. Notice that I have m, I have r, but I don't have alpha. Right? So what you're gonna do is you're going to go over here and try to find alpha. Okay. So let me plug in these numbers. So were the only thing we're missing is alpha. M is 200. R is 3 squared. Alpha so as soon as we have alpha, we can plug it in there. Okay? Now back to the motion equations. So the basic idea is you get stuck and you go to the other side. Back to the motion equations, we have 3 variables, which means we can solve. This is my target, and this is my ignored variable. So I'm going to use the only equation that does not have a ∆θ in it. And the only equation that doesn't have a ∆θ in it is the first equation. Okay. So ωfinal=ωinitial+αt And we're looking for alpha. So alpha is going to be ωfinal-ωinitial/t. This, by the way, is the definition of alpha. It's the change in omega over the change in t. You could have started there as well. That would have worked. This is 0 minus negative 6π and the time is 10 seconds. So these cancel and end up with 6π over 10, positive 6π over 10, which is 1.88. I got a positive which should make sense even though I'm slowing down. Right? Let's talk about that real quick. My velocity is negative. If I'm slowing down, I'm trying to make my velocity positive. So my acceleration should be positive. I'm trying to make my velocity more positive. Another way to think about this that might be even easier is you have a negative omega and you're trying to slow down. So you to go in the other direction, the acceleration is to go in the other direction. So the acceleration would oppose it because you're trying to slow down and this is counterclockwise, which would be positive. Okay? Anyway, my acceleration is 1.8 radians per second squared. Now I can plug this in here and we are done. So if I multiply all of that, and then I multiply that by 1 point 88, I should get, I get 1354 Newton meter. 1354 Newton meter. And that's it. That's the final answer. So just to recap real quick, there's basically 2 parts to this. We were asked for torque. So you could have started here, and then you start plugging stuff in. And you realize you don't have alpha, but you have a bunch of motion equations, motion variables. So you can find alpha using one of the motion equations, plug it back in. Right? So it's the classic, standard type of physics question where you get stuck with something, go look for another variable, plug it in, come back with the value you got, and and solve. Alright? That's it. Hopefully, it makes sense. Let me know if you have any questions and let's keep going.
- 0. Math Review31m
- 1. Intro to Physics Units1h 23m
- 2. 1D Motion / Kinematics3h 56m
- Vectors, Scalars, & Displacement13m
- Average Velocity32m
- Intro to Acceleration7m
- Position-Time Graphs & Velocity26m
- Conceptual Problems with Position-Time Graphs22m
- Velocity-Time Graphs & Acceleration5m
- Calculating Displacement from Velocity-Time Graphs15m
- Conceptual Problems with Velocity-Time Graphs10m
- Calculating Change in Velocity from Acceleration-Time Graphs10m
- Graphing Position, Velocity, and Acceleration Graphs11m
- Kinematics Equations37m
- Vertical Motion and Free Fall19m
- Catch/Overtake Problems23m
- 3. Vectors2h 43m
- Review of Vectors vs. Scalars1m
- Introduction to Vectors7m
- Adding Vectors Graphically22m
- Vector Composition & Decomposition11m
- Adding Vectors by Components13m
- Trig Review24m
- Unit Vectors15m
- Introduction to Dot Product (Scalar Product)12m
- Calculating Dot Product Using Components12m
- Intro to Cross Product (Vector Product)23m
- Calculating Cross Product Using Components17m
- 4. 2D Kinematics1h 42m
- 5. Projectile Motion3h 6m
- 6. Intro to Forces (Dynamics)3h 22m
- 7. Friction, Inclines, Systems2h 44m
- 8. Centripetal Forces & Gravitation7h 26m
- Uniform Circular Motion7m
- Period and Frequency in Uniform Circular Motion20m
- Centripetal Forces15m
- Vertical Centripetal Forces10m
- Flat Curves9m
- Banked Curves10m
- Newton's Law of Gravity30m
- Gravitational Forces in 2D25m
- Acceleration Due to Gravity13m
- Satellite Motion: Intro5m
- Satellite Motion: Speed & Period35m
- Geosynchronous Orbits15m
- Overview of Kepler's Laws5m
- Kepler's First Law11m
- Kepler's Third Law16m
- Kepler's Third Law for Elliptical Orbits15m
- Gravitational Potential Energy21m
- Gravitational Potential Energy for Systems of Masses17m
- Escape Velocity21m
- Energy of Circular Orbits23m
- Energy of Elliptical Orbits36m
- Black Holes16m
- Gravitational Force Inside the Earth13m
- Mass Distribution with Calculus45m
- 9. Work & Energy1h 59m
- 10. Conservation of Energy2h 54m
- Intro to Energy Types3m
- Gravitational Potential Energy10m
- Intro to Conservation of Energy32m
- Energy with Non-Conservative Forces20m
- Springs & Elastic Potential Energy19m
- Solving Projectile Motion Using Energy13m
- Motion Along Curved Paths4m
- Rollercoaster Problems13m
- Pendulum Problems13m
- Energy in Connected Objects (Systems)24m
- Force & Potential Energy18m
- 11. Momentum & Impulse3h 40m
- Intro to Momentum11m
- Intro to Impulse14m
- Impulse with Variable Forces12m
- Intro to Conservation of Momentum17m
- Push-Away Problems19m
- Types of Collisions4m
- Completely Inelastic Collisions28m
- Adding Mass to a Moving System8m
- Collisions & Motion (Momentum & Energy)26m
- Ballistic Pendulum14m
- Collisions with Springs13m
- Elastic Collisions24m
- How to Identify the Type of Collision9m
- Intro to Center of Mass15m
- 12. Rotational Kinematics2h 59m
- 13. Rotational Inertia & Energy7h 4m
- More Conservation of Energy Problems54m
- Conservation of Energy in Rolling Motion45m
- Parallel Axis Theorem13m
- Intro to Moment of Inertia28m
- Moment of Inertia via Integration18m
- Moment of Inertia of Systems23m
- Moment of Inertia & Mass Distribution10m
- Intro to Rotational Kinetic Energy16m
- Energy of Rolling Motion18m
- Types of Motion & Energy24m
- Conservation of Energy with Rotation35m
- Torque with Kinematic Equations56m
- Rotational Dynamics with Two Motions50m
- Rotational Dynamics of Rolling Motion27m
- 14. Torque & Rotational Dynamics2h 5m
- 15. Rotational Equilibrium3h 39m
- 16. Angular Momentum3h 6m
- Opening/Closing Arms on Rotating Stool18m
- Conservation of Angular Momentum46m
- Angular Momentum & Newton's Second Law10m
- Intro to Angular Collisions15m
- Jumping Into/Out of Moving Disc23m
- Spinning on String of Variable Length20m
- Angular Collisions with Linear Motion8m
- Intro to Angular Momentum15m
- Angular Momentum of a Point Mass21m
- Angular Momentum of Objects in Linear Motion7m
- 17. Periodic Motion2h 9m
- 18. Waves & Sound3h 40m
- Intro to Waves11m
- Velocity of Transverse Waves21m
- Velocity of Longitudinal Waves11m
- Wave Functions31m
- Phase Constant14m
- Average Power of Waves on Strings10m
- Wave Intensity19m
- Sound Intensity13m
- Wave Interference8m
- Superposition of Wave Functions3m
- Standing Waves30m
- Standing Wave Functions14m
- Standing Sound Waves12m
- Beats8m
- The Doppler Effect7m
- 19. Fluid Mechanics2h 27m
- 20. Heat and Temperature3h 7m
- Temperature16m
- Linear Thermal Expansion14m
- Volume Thermal Expansion14m
- Moles and Avogadro's Number14m
- Specific Heat & Temperature Changes12m
- Latent Heat & Phase Changes16m
- Intro to Calorimetry21m
- Calorimetry with Temperature and Phase Changes15m
- Advanced Calorimetry: Equilibrium Temperature with Phase Changes9m
- Phase Diagrams, Triple Points and Critical Points6m
- Heat Transfer44m
- 21. Kinetic Theory of Ideal Gases1h 50m
- 22. The First Law of Thermodynamics1h 26m
- 23. The Second Law of Thermodynamics3h 11m
- 24. Electric Force & Field; Gauss' Law3h 42m
- 25. Electric Potential1h 51m
- 26. Capacitors & Dielectrics2h 2m
- 27. Resistors & DC Circuits3h 8m
- 28. Magnetic Fields and Forces2h 23m
- 29. Sources of Magnetic Field2h 30m
- Magnetic Field Produced by Moving Charges10m
- Magnetic Field Produced by Straight Currents27m
- Magnetic Force Between Parallel Currents12m
- Magnetic Force Between Two Moving Charges9m
- Magnetic Field Produced by Loops and Solenoids42m
- Toroidal Solenoids aka Toroids12m
- Biot-Savart Law (Calculus)18m
- Ampere's Law (Calculus)17m
- 30. Induction and Inductance3h 37m
- 31. Alternating Current2h 37m
- Alternating Voltages and Currents18m
- RMS Current and Voltage9m
- Phasors20m
- Resistors in AC Circuits9m
- Phasors for Resistors7m
- Capacitors in AC Circuits16m
- Phasors for Capacitors8m
- Inductors in AC Circuits13m
- Phasors for Inductors7m
- Impedance in AC Circuits18m
- Series LRC Circuits11m
- Resonance in Series LRC Circuits10m
- Power in AC Circuits5m
- 32. Electromagnetic Waves2h 14m
- 33. Geometric Optics2h 57m
- 34. Wave Optics1h 15m
- 35. Special Relativity2h 10m
Torque with Kinematic Equations: Study with Video Lessons, Practice Problems & Examples
 Created using AI
Created using AIIn rotational dynamics, torque tau is calculated using the equation \( \tau = I \alpha \), where \( I \) is the moment of inertia and \( \alpha \) is angular acceleration. For a solid sphere, \( I = \frac{2}{5} m r^2 \). To find \( \alpha \), use the motion equation \( \omega_f = \omega_i + \alpha t \). Converting RPM to angular velocity (\( \omega \)) is essential, as is understanding the relationship between angular acceleration and torque when stopping a rotating object. This synthesis of concepts enhances problem-solving in physics.
Torque with Kinematic Equations
Video transcript
A light, long rope is wrapped around a solid disc, in such a way that pulling the rope causes the disc to spin about a fixed axis perpendicular to itself and through its center. The disc has mass 40 kg, radius 2 m, and is initially at rest, and the rope unwinds without slipping. You pull on the rope with a constant 200 N. Use the rotational version of Newton's Second Law to calculate how fast (in rad/s) the disc be spinning after you pull 50 m of rope.
A system is made of two small, 3 kg masses attached to the ends of a 5 kg, 4 m long, thin rod, as shown. The system is free to rotate about an axis perpendicular to the rod and through its center. Two forces, both of magnitude F and perpendicular to the rod, are applied as shown below. What must the value of F be to the system from rest to 10 rad/s in exactly 8 complete revolutions?
3.8 N
6.0 N
7.7 N
15.4 N
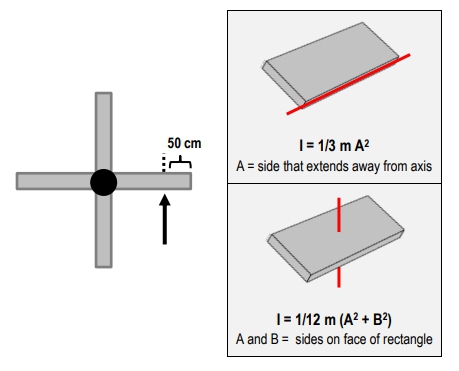
Two rotating doors, each 6.0 m long, are fixed to the same central axis of rotation, as shown (top view). When you push on one door with a constant 100 N, directed perpendicular from the face of the door and 50 cm from its outer edge, the rotating door system takes 8 s to complete a full revolution from rest. The doors can be modeled as thin rectangles (moments of inertia for thin rectangles, around two different axes, are shown for reference). Calculate the mass of the system.
Stopping flywheel with friction
Video transcript
Hey guys. So in this example, we have a flywheel that's spinning, and we're going to press an object against the wheel to cause it to stop. So imagine you have something spinning, and if you squeeze an object against it, if you push an object against it, it would stop. And we want to know how hard do you have to push against the flywheel so that it stops in a particular amount of time. Okay. So it says here, a flywheel is a rotating disc. This means we're going to use the moment of inertia of a disc, which is half mr2. Same as a solid cylinder. And it's used to store energy. Suppose this one has a mass of 8 × 104 kilograms and has a diameter of 5 meters, which means we're going to immediately change the diameter into a radius of 2.5. And it's set up vertically. So, we got this wheel that's vertical and it's free to spin around a fixed axis. So, there's a fixed axis perpendicular to the wheel. So basically, the wheel spins like this around a central axis. To slow down the flywheel, I mentioned this. You push this block here. So, there's a force that you apply. I want to point out that once you push here, it's going to have a contact. Therefore, there's going to be a normal force back, and this normal force will have the same magnitude as your force. Force. So, action-reaction, if you push with 10, the normal pushes back with 10. It says here that the coefficients of friction between the block and the wheel right here, the coefficients of friction are 0.8 and 0.6. So I'm going to write them here. Remember, when you have 2 coefficients of friction, the static coefficient is the greater one. So 0.8, and μkinetic is 0.6. Okay. And we want to know what is how hard do you have to push. So, I want to know what is f. It says here that the wheel is going to come to a complete stop. So Ωfinal will be 0 from an RPM of 300. So RPMinitial is 300, and it's going to do this in a Δt of 30 seconds. Remember, RPM almost always gets converted into Ω. So, I'm going to do that real quick. I'm going to say Ωinitial equals 2π RPM over 60. That's the equation to convert to 2 and if you multiply this you get 10 π. Okay. 10 π radians per second and notice that now we have I'm going to actually move that over here, and scratch this out just because I'm trying to list all my motion variables. And I'm trying to make the point to you that as of now, we already have 3 motion variables, which is good news. It means we could solve for the others. The other equations the other variables that are missing here are α and Δθ. Okay. So all I'm doing is grabbing the information and sort of fixing it up. So, how hard do you have to push? Again, this is a force that's going to cause a torque. Right? And so we're going to use the force, the sum of all torques equals I α equation, but I want to explain to you what's going on here. So, the idea is that the wheel spinning this way. Let's say with an Ωinitial. And when you push, there's now a normal force here. I had that drawn earlier. And what that means is because there's a normal force and there's friction, there's going to be friction acting against motion. So motion is rotating that way. Friction is going to try to stop the wheel, which means there's going to be a force of friction this way. Okay. So you got normal force this way, and there's a force of friction this way. What that force of friction does, it causes a torque like this. Torque of friction, which is opposite to my velocity. So it's trying to slow it down. It's trying to get it to stop. Okay? In this particular case, just because of the way I drew it, this is actually negative and then this would be positive. As long as they're opposite each other, you're fine. Okay. So there is a torque. There's an acceleration. This is a force problem with angular acceleration with torque. So we're going to start here, and we have to find what this f is. Okay. The only so let's expand this equation real quick. The only torque acting on this is a torque due to friction because you're pushing against this thing. So we're going to write torque of friction. The moment of inertia the moment of inertia it says here I didn't read this part to you but it says here you may assume the wheel's entire mass is concentrated at its outer rim. This means that this is not a, this is actually not a rotating disc. I apologize. This is not a rotating disk. This is going to be a hollow disk. Okay. So it's going to be hollow disk. So instead of half mr2, let me just delete this. Instead of half mr2, it's going to be just mr2. Okay. Where r is the radius. So I'm going to put this here, mr2 and α. We don't have α, but we could find α if we wanted to. Okay. Let's take this one step further and expand this. Torque is force little r sine of θ. Force in this case is friction. So it's little f. R is the distance from the axis of rotation to the point where the force happens. This is the distance that we are talking about. Okay. This is my r vector. Because the force happens here, and the axis is obviously in the middle here. The r vector is as long as the radius of the wheel because friction happens at the edge there. So we're going to have f big R and then sine of θ. The angle will be 90 degrees. Notice how they make an angle of 90 degrees because friction straight down as a result of the pushing against it. And by the way, friction is always in situations like this, friction is always going to make an angle of 90 degrees, and it's always going to happen at the edge. Right? So sine of 90. So that's nice. And mr2, the radius, we have that as well. We're going to be able to plug it in there and then α. And we're looking for f. Now don't get confused. We're not looking for little f. We're looking for big f. So what we're going to do is we're going to keep expanding this equation. Friction can be expanded. Friction is μnormal. So I could rewrite friction as μnormal. That's what we're going to do except there's one change, which is normal is the same thing as f. So I'm going to plug in f here, and I'm going to write friction as μf instead of normal's. It's the same thing. The reason we do that is because now, finally, our equation actually has our variable. Right? Until then, you haven't seen your variable around. So, that's that. Let's I'm going to rewrite this here. I'm going to cancel this r with this r. This is just a one. So it's going to be m μf equals mr α. So f is mr α over μ, and we know these numbers. M is or most of them, 8 × 104. Radius is 2.5. I got to go find α and then we do have μ. Which μ do you think we use? Kinetic or static? And I hope you're thinking kinetic because the block is rubbing against the disk. So, it's going to be 0.6, which is kinetic. Okay. And we have to go find α. So let's go do that real quick. So, we're going to go over here and look for α. To find α, I can use motion equations. I have 3 knowns. This is my target. This is my ignore variable, which means I can use the first equation to find α. Ωfinal equals Ωitial plus αt. We're looking for α. So let's move everything out of the way. You might notice that this is the definition of acceleration of angular acceleration, which is change in Ω over the tuning time. You could have started there as well. The final Ω is 0 because we're looking to stop. And the initial Ω is 10 pi radians per second. Now notice that the way we drew it, it's a negative. So let's plug it as a negative. And then the time the time we're going is 30 seconds. Okay? Now if you do this if you do this, you end up with an α of 1.05 radians per second squared. Highlight this here and that's what I'm going to put right here. 1.05. Right there. Now if you multiply it once you multiply this whole thing once you multiply this whole thing, let me make sure I got it right. Yep. You end up with 3.5 times 105 Newton's. Okay. So that's how much force you have to push against the block with, and push the block against the wheel so that this thing stops in 30 seconds. Alright. So that's it for this one. This question is fairly popular. It's a little bit tricky. But again, I think the key thing is to realize you have a force causing a torque, causing acceleration, that this is the starting place. The trickiest part, I think here, is to try to make a connection from, this initial equation and then how we're going to get to f. And sometimes you just have to trust that if you keep expanding the equation like we did here, and then we expanded the f here. You have to trust that if you keep expanding, the variable will show up. Alright. So that's it for this one. Let me know if you have any questions and let's keep going.
A 1,000 kg disc that has a 5 m outer radius is mounted on a vertical, inner axle 80 kg in mass and 1 m in radius. A motor acts on the axle to speed up or slow down the system. Suppose the motor stops functioning when the system is spinning at 70 rad/s. To bring it to a complete stop, you apply a constant 200 N friction to the surface of the axle. How many revolutions will the system take to stop?
5.8
2.6×104
1.6×105
2.5×105
Do you want more practice?
More setsHere’s what students ask on this topic:
How do you calculate torque in rotational dynamics?
In rotational dynamics, torque (τ) is calculated using the equation , where is the moment of inertia and is the angular acceleration. The moment of inertia depends on the shape and mass distribution of the object. For example, for a solid sphere, . Angular acceleration can be found using kinematic equations such as .
 Created using AI
Created using AIWhat is the relationship between torque and angular acceleration?
The relationship between torque (τ) and angular acceleration (α) is given by the equation , where is the moment of inertia. This equation is the rotational analog of Newton's second law of motion (F = ma). It shows that the torque applied to an object is directly proportional to its angular acceleration, with the moment of inertia acting as the proportionality constant.
 Created using AI
Created using AIHow do you convert RPM to angular velocity?
To convert RPM (revolutions per minute) to angular velocity (ω) in radians per second, use the formula . This conversion is necessary because most rotational motion equations use angular velocity in radians per second rather than RPM.
 Created using AI
Created using AIHow do you find angular acceleration using kinematic equations?
Angular acceleration (α) can be found using the kinematic equation . Rearrange this equation to solve for α: . Here, is the final angular velocity, is the initial angular velocity, and is the time interval.
 Created using AI
Created using AIWhat is the moment of inertia for a solid sphere?
The moment of inertia (I) for a solid sphere rotating about an axis through its center is given by the formula , where is the mass of the sphere and is its radius. This formula is specific to solid spheres and differs for other shapes and mass distributions.
 Created using AI
Created using AI