Impulse with Variable Forces: Videos & Practice Problems

Impulse is calculated using the area under a force versus time graph, where positive areas indicate positive impulse and negative areas indicate negative impulse. The impulse-momentum relationship states that impulse equals the change in momentum, expressed as $J^{=}(mv\_f-mv\_i)$. For a toy car, calculating the areas under the graph yields an impulse of 12.5 N·s, leading to a final speed of 6.25 m/s when starting from rest.

Impulse with Variable Forces

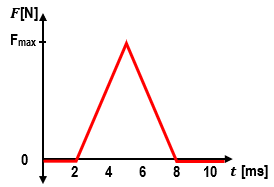
In physics, impulse is a crucial concept that relates to the change in momentum of an object. When analyzing force versus time graphs, the impulse can be determined by calculating the area under the curve of the graph. This is analogous to how work is calculated from force versus position graphs, where the area under the curve represents the work done. In the case of force versus time graphs, areas above the time axis correspond to positive impulse, while areas below indicate negative impulse.

To calculate impulse from a force versus time graph, one can break the graph into distinct geometric shapes, such as triangles and rectangles, and compute the area of each shape. For example, if a graph shows a force that varies over time, the impulse can be found by summing the areas of these shapes. The formula for the area of a triangle is given by:

$$ \text{Area} = \frac{1}{2} \times \text{base} \times \text{height} $$

And for a rectangle, it is simply:

$$ \text{Area} = \text{base} \times \text{height} $$

Once the areas are calculated, they can be added together to find the total impulse. For instance, if the total area above the time axis is 30 Newton-seconds and the total area below is -17.5 Newton-seconds, the overall impulse would be:

$$ \text{Impulse} = 30 + (-17.5) = 12.5 \text{ Newton-seconds} $$

This positive impulse indicates that the net effect of the forces applied over the time interval resulted in a forward momentum change.

Furthermore, impulse is directly related to momentum through the equation:

$$ J = \Delta p = m \cdot v_{\text{final}} - m \cdot v_{\text{initial}} $$

Where \( J \) is the impulse, \( m \) is the mass, and \( v \) represents velocity. If an object starts from rest, the initial velocity is zero, simplifying the equation to:

$$ J = m \cdot v_{\text{final}} $$

In a practical example, if a remote-controlled car has a mass of 2 kilograms and the calculated impulse is 12.5 Newton-seconds, the final velocity can be determined by rearranging the equation:

$$ v_{\text{final}} = \frac{J}{m} = \frac{12.5}{2} = 6.25 \text{ meters per second} $$

This calculation illustrates how impulse not only quantifies the effect of forces over time but also provides insight into the resulting motion of an object.

An object experiences a force given by the graph below. What value of F_max would give an impulse of 6 N⋅s?

• A. 1000 N
• B. 4000 N
• C. 2000 N
• D. 6000 N

Answer: (C)

Here’s what students ask on this topic:

How do you calculate impulse from a force vs. time graph?

To calculate impulse from a force vs. time graph, you need to find the area under the curve. The impulse (J) is the integral of the force (F) over the time interval (Δt). For a graph with varying force, break the area into geometric shapes like triangles and rectangles, calculate their areas, and sum them up. Positive areas above the x-axis contribute to positive impulse, while areas below the x-axis contribute to negative impulse. The total impulse is the net area under the curve.

What is the relationship between impulse and momentum?

The relationship between impulse (J) and momentum (p) is given by the impulse-momentum theorem, which states that the impulse applied to an object is equal to the change in its momentum. Mathematically, this is expressed as:

$J=\mathrm{\Delta p}=mv$_f-mv_i

where m is the mass, v_f is the final velocity, and v_i is the initial velocity. This means that the impulse is equal to the product of mass and the change in velocity.

How do you find the final velocity of an object using impulse?

To find the final velocity (v_f) of an object using impulse, you can use the impulse-momentum theorem. First, calculate the impulse (J) from the force vs. time graph. Then, use the equation:

$J=mv$_f-mv_i

If the object starts from rest (v_i = 0), the equation simplifies to:

$J=mv$_f

Solve for v_f by dividing the impulse by the mass (m):

$v$_f=Jm

What is the significance of positive and negative areas in a force vs. time graph?

In a force vs. time graph, the areas above the x-axis represent positive impulse, while the areas below the x-axis represent negative impulse. Positive impulse indicates that the force is acting in the positive direction, increasing the object's momentum in that direction. Negative impulse indicates that the force is acting in the negative direction, decreasing the object's momentum or changing its direction. The net impulse is the sum of these positive and negative areas, determining the overall change in momentum.

Why can't you use the formula J = FΔt directly for variable forces?

The formula J = FΔt assumes that the force (F) is constant over the time interval (Δt). For variable forces, the force changes over time, so this formula is not applicable. Instead, you need to calculate the impulse by finding the area under the force vs. time graph, which accounts for the varying force. This method involves breaking the graph into geometric shapes, calculating their areas, and summing them to find the total impulse.