LR Circuits - Online Tutor, Practice Problems & Exam Prep

An LR circuit consists of an inductor (L) and a resistor (R). When a switch closes, current grows, reaching a maximum value of $V/R$ due to the battery's influence. This growth is resisted by the inductor, described by an exponential function. Conversely, when the battery is disconnected, current decays through the resistor, also following an exponential decay pattern. The time constant $\tau =L/R$ determines the rate of these changes, influencing how quickly current stabilizes or dissipates.

LR Circuits

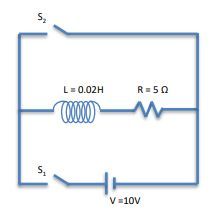
Hey, guys. So now that we know how inductors behave in circuits, we're going to take a look at a specific type of circuit that you're going to need to know about called an LR circuit. Let's check it out. So, an LR circuit or an RL circuit, as you'll see in some textbooks, is simply a circuit containing an inductor, and we know that inductors have the letter L and a resistor. And so, the resistor is the R part of the LR circuit. Right? So we've got this schematic that's up here that sort of labels a battery that is connected to an inductor and a resistor, and we have some switches. And the key thing here is that, depending on the switch positions, there are two different processes that can happen in this circuit. So let's check them out. Imagine that we have S2 that remains open. So that's this top guy right here. And if S2 remains open, then that means that this whole entire circuit, so it doesn't really matter. There's nothing that can flow through it. And S1 is now closed. So when you close S1, you've now created a continuous loop for electrons to flow. So, electrons are going to flow in this loop right here through the inductor and the resistor. Now because it's connected to this battery right here, this battery wants to supply a lot of electrons and it's going to supply the current. And what happens is that the current wants to increase in this phase. So we call this current growth. So this happens when S1 is closed. So now let's imagine that we take the circuit, we let it run for a very long time, and then at some point, we're going to close this S2 switch right here, and then we're going to open this S1. So now it's basically like this half of the circuit doesn't really exist and the top one does. So what happens now is when we close S2, so when S2 is closed, we now have a different circuit, a different loop that flows through the inductor and the resistor. And if this has been running for a long time, there's going to be some current that is built up in this loop. I'm just going to pretend that it goes in this direction. It could go in the other direction. We don't really know. So we have all this current right here, and the resistor is going to bleed off that current. So it's going to slowly dissipate that current, and without the battery in the top half of the loop, the current is eventually going to decrease. So we call this process current decay. This is basically an overview of the two types of processes that you can see. We're going to take a look at each one of them a lot more carefully in this next section right here.

Current growth is again when you have the battery that is connected, and that's like the dead giveaway that you have current growth. So when you have or when you're told information that the battery is connected or you have a schematic in which there's a switch or something like that, and you close that circuit and now the battery is connected, that's your dead giveaway that you have current growth. Now this battery, what it wants to do is it wants to basically supply this current throughout the circuit. But remember, so this, you know, this current is going to start off as a very very slow value and it wants to or as a very low value, it's actually going to be 0. And this current wants to very very quickly reach its maximum value. But remember that the function of an inductor is that inductors always resist large changes in currents. The self-induced EMF is equal to, or it's proportional to ΔI/Δt. So the larger the change in the current through this circuit, the larger the EMF that's going to be backward that's going to fight against it. So what happens is this inductor basically resists any large changes in currents. So this inductor is going to resist that growing current, and the equation that governs that is going to be this exponential equation right here. In fact, it's very very similar to the exponential equation that we saw for RC circuits with just a few small differences here. So what ends up happening is that as time goes on, as t goes on, there's now more of the current that's built up in the circuit. There's less ΔI Δt. The inductor gets weaker and weaker and weaker, and eventually, this current reaches its maximum value. So what we're going to see is that as t goes to some very very large numbers, I'm going to write infinity over here. This exponential, e^−t/τ, eventually just goes to 0. So if you plug in a very large number inside of this exponential, this basically just goes away. This term goes away. So the phrase that you might see in problems is that, after some long time. So this is usually what you'll see. So after a long time, and what that phrase just means is that the system is sort of able to get to its sort of final state. Right? So it's able to do this, and this exponential term is going to go away. So after a long time, what ends up happening is that the current will eventually reach its maximum value of V/R.

And so if you were to plot this equation out, it looks again very similar to the way that charges build up on capacitors in an RC circuit. So we have the current that starts at 0 and then at some later time, we're going to reach this maximum value of V/R. So it's going to kinda look like this. So it wants to sort of rush out all this current, but the inductor is slowing it down. And then after a long time, it sort of levels out and then reaches its maximum value. So that is current growth. So then if you were to sort of run this circuit for a very long time and let all of that current build up to its maximum value, and then you were to disconnect it from the battery. Now we move on to the second phase, which is current decay. So the easiest way you can tell that you have current decay is that, unlike we had current growth, the battery is disconnected. So we have no battery here. There is nothing left to supply those electrons or that current that's going through the battery. And so what ends up happening is that this resistor is eventually going to dissipate that current away. Now again, just like the inductor was trying to resist the growing currents, this inductor is trying to resist the weakening current or the decreasing current from this resistor. So what happens here is that we have some maximum current that's going through this circuit, so this is going to be I_max. So we're going to start off with some maximum value like this. And what happens is that this current is going to eventually get dissipated through the resistor like we said, but this inductor is going to resist the decreasing current. And the equation that governs that is now this exponential right here. Looks very similar to the other one, but again we don't have that one minus sign in there. That one minus e^−t/τ. So what happens here is that as t gets very very big, so we have as t approaches some very large number after a long time, this exponential, e^−t/τ, eventually becomes 0. So if this term eventually becomes 0, then this whole entire thing will eventually become 0 after a very very long time. So this current will eventually get bled off by the resistor and the inductor was told to resist the decreasing current less and less and less. So that means after a very long time, you're going to asymptotically approach 0. Now in both of these problems, it's basically the two different kinds of exponentials that you'll see. Again, it's very similar to how RC circuits work. And the constant that appears in this is the time constant. That's the τ right here. And so, unlike RC circuits, this τ is given as the inductance L over R. And basically what this time constant does is it just determines how quickly the growth and decay cycles occur. So a lower time constant, meaning that L, your inductance is lower or the resistance is higher, means that these changes in either growth or decay happen much much much faster. So if you have a lower time constant, it means that not as much time needs to pass in order for these cycles to occur. Whereas if you have a much higher inductance or the resistance is lower, so if you have a higher L or a lower R, that just means that these changes happen much slower, because the inductor is able to sort of resist these changes happening a lot better. Okay? So that's basically a summary of how current growth, how current decay works in an LR circuit. Let's go ahead and take a look at some problems.

Unknown Resistance in an LR Circuit

Hey, guys. So let's get some more practice with LR circuits. We have an LR circuit with a time constant, and it's initially connected to a battery. And then after some very, very small amount of time, it is disconnected from the battery. The current is something, and we're supposed to figure out what the resistance of this circuit is. So hopefully, you guys realized that this word here, it's disconnected from the battery, means that we are working with decay. So we're working with current decay right here. So let's see. We have a time constant which is tau, that's equal to 0.025. We have a voltage, that's v is equal to 10 volts. We have time, which is equal to 0.005 seconds. And we have the currents at that specific times, that's I when t is equal to this value right here. And we're supposed to figure out what is the resistance of this circuit. So hopefully, you guys realize that if we're working with current decay, then the equation that's going to tie all these things together is the current decay equation. That's I(t) is equal to v/r, and then we just have the inverse exponential e to the minus t over tau. The one minus sign is for current growth because eventually what happens is we want that v/r to sort of be like away. You know, we want it to be isolated. Right? So this is the equation that is eventually going to go to 0 as time gets really large. Got it. So here's what we have. We have the resistance of the circuit. That's going to be our target variable right here. And let's see. We have the current as a function of time, and we actually have what the current is at a specific, value in time, so when t is a specific number. So all we have to do is Let's see. I have I(t). I know what the voltage is. I know what the time is. The time I'm plugging in is just this number right here, and I have the current at that specific time, and I have what the time constant is. So all I have to do is just move this over to the other side. So basically what happens is that just like sort of an algebraic equation, this r can go up to the top and this I(t) can actually go down to the bottom and basically just trade places with it. So that means that r is equal to v over I when t is equal to, you know, 0.005. And by the way, I'm just evaluating the current at a specific time. And then we have e to the minus t over tau. So let's plug in all of our numbers. R is equal to the voltage, which is 10. I at the specific time is just equal to 0.5, then we have e to the minus, and then t which is equal to 0.005, and then we have over tau, and tau is 0.025. Okay. So hopefully you guys plug this in. You can actually, you know, you could take an extra step and sort of, like, simplify this a little bit. 10 over 0.5 actually just becomes 20 and this exponential right here is actually e-0.2. As you plug this into your calculator, you guys should get 16.4, and that's going to be in ohms. So that's the resistance of this circuit. Let me know if you guys have any questions.

Consider the LR circuit shown below. Initially, both switches are open. Switch 1 is closed. a) What is the maximum current in the circuit after a long time? Then, S₁ is opened and S₂ is closed. b) What is the current in the circuit after 0.05s?

• A. (a) i_max=0.5 A; (b) i (0.05s)=1.86×10⁻⁶ A
• B. (a) i_max=2 A; (b) i (0.05s)=7.45×10⁻⁶ A
• C. (a) i_max=0.5 A; (b) i (0.05s)=0.14 A
• D. (a) i_max=2 A; (b) i (0.05s)=0.57 A
• E. (a) i_max=0.5 A; (b) i (0.05s)=0.46 A A
• F. (a) i_max=2 A; (b) i (0.05s)=1.8 A

An LR circuit with L = 0.1 H and R = 10 Ω are connected to a battery with the circuit initially broken. When the circuit is closed, how much time passes until the current reaches half of its maximum value?

• A. 6.9×10⁻⁵ s
• B. 6.9×10⁻³ s
• C. 0.69 s
• D. Not enough information to tell

Here’s what students ask on this topic:

What is an LR circuit and how does it work?

An LR circuit, also known as an RL circuit, consists of an inductor (L) and a resistor (R) connected in series with a power source. When a switch in the circuit is closed, current begins to flow, and the inductor initially resists changes in current due to its property of self-inductance. This results in an exponential growth of current, described by the equation:

$I=\frac{V}{R}(1-e^{\frac{-}{t}})$

where $\tau =\frac{L}{R}$ is the time constant. When the battery is disconnected, the current decays exponentially through the resistor, following:

$I=I{\max }_e{-}^{\frac{t}{\tau }}$

where $I{\max }_{}$ is the initial current. The time constant $\tau$ determines the rate of current growth and decay.

What is the time constant in an LR circuit and how is it calculated?

The time constant in an LR circuit, denoted as $\tau$, determines the rate at which current grows or decays in the circuit. It is calculated using the formula:

$\tau =\frac{L}{R}$

where $L$ is the inductance of the inductor in henries (H) and $R$ is the resistance of the resistor in ohms (Ω). The time constant represents the time it takes for the current to reach approximately 63% of its maximum value during growth or to decay to about 37% of its initial value during decay. A smaller time constant means faster changes, while a larger time constant indicates slower changes.

How does current growth occur in an LR circuit?

Current growth in an LR circuit occurs when a switch is closed, connecting the circuit to a battery. Initially, the current is zero, but it begins to increase as the battery supplies electrons. The inductor resists this change due to its property of self-inductance, causing the current to grow exponentially rather than instantaneously. The growth of current is described by the equation:

$I=\frac{V}{R}(1-e^{\frac{-}{t}})$

where $V$ is the voltage of the battery, $R$ is the resistance, $t$ is time, and $\tau$ is the time constant $\tau =\frac{L}{R}$. The current eventually reaches a maximum value of $\frac{V}{R}$.

What happens during current decay in an LR circuit?

Current decay in an LR circuit occurs when the battery is disconnected, and the switch is positioned to allow current to flow only through the inductor and resistor. Without the battery, the current begins to decrease as the resistor dissipates the energy stored in the inductor. The inductor resists this decrease, causing the current to decay exponentially. The decay of current is described by the equation:

$I=I{\max }_{}{e}^{\frac{-}{t}}$

where $I{\max }_{}$ is the initial current, $t$ is time, and $\tau$ is the time constant $\tau =\frac{L}{R}$. The current eventually approaches zero as the energy is fully dissipated.

How is the behavior of an LR circuit similar to an RC circuit?

The behavior of an LR circuit is similar to an RC circuit in that both involve exponential growth and decay processes. In an LR circuit, the inductor resists changes in current, leading to exponential current growth and decay, described by:

$I=\frac{V}{R}(1-e^{\frac{-}{t}})$ (growth) and $I=I{\max }_{}{e}^{\frac{-}{t}}$ (decay).

In an RC circuit, a capacitor resists changes in voltage, leading to exponential voltage growth and decay, described by:

$V=\frac{V}{0_{}}(1-e^{\frac{-}{t}})$ (growth) and $V=V{0}_{}{e}^{\frac{-}{t}}$ (decay).

In both circuits, the time constant $\tau$ determines the rate of these changes, with $\tau =\frac{L}{R}$ for LR circuits and $\tau =\mathrm{RC}$ for RC circuits.