Electric Flux: Videos & Practice Problems

Electric flux measures how much electric field passes through a surface, akin to water flowing through a ring. It depends on the angle between the electric field and the surface's normal vector. The equation for electric flux is Φ = EAcosθ. Positive flux occurs when the electric field and normal point in the same direction, while negative flux occurs when they point in opposite directions. The total flux through a closed surface is the sum of individual fluxes across its surfaces.

Electric Flux

Electric flux is a fundamental concept in electrostatics, crucial for understanding and applying Gauss's law. It quantifies how much of an electric field passes through a given surface, similar to measuring the flow of water through a ring placed in a river. The electric flux (\(\Phi_E\)) can be visualized as the number of electric field lines that intersect a surface area.

To define electric flux mathematically, we use the equation:

\[ \Phi_E = E \cdot A \cdot \cos(\theta) \]

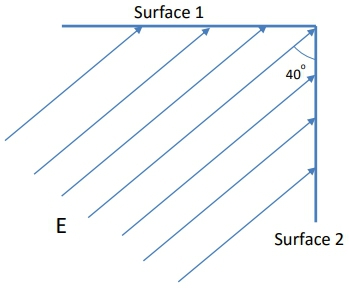
Here, \(E\) represents the magnitude of the electric field, \(A\) is the area of the surface, and \(\theta\) is the angle between the electric field lines and the normal (perpendicular) vector to the surface. The normal vector is crucial as it helps determine how effectively the electric field penetrates the surface.

Electric flux can vary based on the orientation of the surface relative to the electric field. If the surface is aligned with the field (i.e., \(\theta = 0^\circ\)), the flux is maximized. Conversely, if the surface is perpendicular to the field (i.e., \(\theta = 90^\circ\)), no electric field lines pass through, resulting in zero flux. When the surface is at an angle, the flux is a partial amount, depending on the cosine of the angle.

When considering a closed surface, such as a cube, the total electric flux through the surface is the sum of the electric fluxes through each individual face. This is expressed as:

\[ \Phi_{\text{net}} = \Phi_1 + \Phi_2 + \Phi_3 + \Phi_4 + \Phi_5 + \Phi_6 \]

In this context, positive flux indicates that electric field lines are exiting the surface, while negative flux indicates that they are entering. The directionality of the electric field relative to the normal vector determines the sign of the flux. For example, if the electric field and the normal vector point in the same direction, the flux is positive; if they point in opposite directions, the flux is negative.

To illustrate, if you calculate the total flux through a cube with individual flux values of \(100\), \(20\), \(-40\), and \(-80\), the net flux would be:

\[ \Phi_{\text{net}} = 100 + 20 - 40 - 80 = 0 \]

This example demonstrates how to sum the contributions of each face to find the overall electric flux through a closed surface. Understanding electric flux is essential for solving problems in electrostatics and applying Gauss's law effectively.

The electric flux through each surface of a cube is given below. Which surfaces of the cube does the electric field run parallel to? 
Φ₁ = 100 Nm² /C      Φ₄ = 0 Nm² /C
Φ₂ = 20 Nm² /C       Φ₅ = −40 Nm² /C
Φ₃ = 0 Nm² /C        Φ₆ = −80 Nm² /C

• A. 1 and 2
• B. 3 and 4
• C. 5 and 6
• D. 1, 2, 5, and 6

Answer: (B)

Flux Through Angled Surface

To calculate the electric flux through a surface, we utilize the formula for electric flux, which is given by:

\(\Phi_E = E \cdot A \cdot \cos(\theta)\)

In this equation, \(\Phi_E\) represents the electric flux, \(E\) is the magnitude of the electric field, \(A\) is the area of the surface, and \(\theta\) is the angle between the electric field and the normal (perpendicular) vector to the surface.

In this scenario, we know the electric field strength is 100 Newtons per Coulomb and the area of the surface is 1 square meter. The challenge lies in determining the correct angle \(\theta\). It is crucial to note that \(\theta\) should be the angle between the electric field and the normal to the surface, not the angle between the electric field and the surface itself.

Given that the angle between the electric field and the surface is 30 degrees, we can find the angle between the electric field and the normal by recognizing that the normal is perpendicular to the surface. Therefore, the angle we need is:

\(\theta = 90^\circ - 30^\circ = 60^\circ\)

Now, substituting the known values into the electric flux formula:

\(\Phi_E = 100 \, \text{N/C} \cdot 1 \, \text{m}^2 \cdot \cos(60^\circ)\)

Since \(\cos(60^\circ) = \frac{1}{2}\), we can simplify the calculation:

\(\Phi_E = 100 \cdot 1 \cdot \frac{1}{2} = 50 \, \text{N m}^2/\text{C}\)

Thus, the magnitude of the electric flux through the surface is 50 Newton meters squared per Coulomb. This value represents the total electric field passing through the specified area, taking into account the orientation of the surface relative to the electric field.

Flux Through Cube

To determine the electric flux through each side of a cube placed in a uniform electric field, we start by understanding the concept of electric flux, which is given by the formula:

Electric Flux (Φ) = E × A × cos(θ)

Here, E represents the electric field strength, A is the area of the surface, and θ is the angle between the electric field and the normal vector to the surface.

In this scenario, we have a cube with a side length of 2 centimeters, which translates to an area of:

A = (0.02 m) × (0.02 m) = 0.0004 m²

Next, we analyze the electric flux through each face of the cube:

1. **Right Side**: The electric field is aligned with the normal vector, making the angle θ = 0°. Thus, the electric flux through the right side is:

Φ_right = E × A × cos(0°) = E × A

2. **Left Side**: The normal vector points in the opposite direction to the electric field, resulting in θ = 180°. Therefore, the electric flux through the left side is:

Φ_left = E × A × cos(180°) = -E × A

3. **Top Side**: The normal vector points upward while the electric field is horizontal, leading to θ = 90°. Consequently, the electric flux through the top side is:

Φ_top = E × A × cos(90°) = 0

4. **Bottom Side**: Similar to the top side, the electric flux through the bottom side is also:

Φ_bottom = E × A × cos(90°) = 0

5. **Front and Back Sides**: For both the front and back sides, the normal vectors are perpendicular to the electric field, resulting in θ = 90°. Thus, the electric flux through both these sides is:

Φ_front = E × A × cos(90°) = 0

Φ_back = E × A × cos(90°) = 0

In summary, the only sides with non-zero electric flux are the right and left sides. If we assume an electric field strength of 100 N/C, the calculations yield:

Φ_right = 100 N/C × 0.0004 m² = 0.04 Nm²/C

Φ_left = -100 N/C × 0.0004 m² = -0.04 Nm²/C

Thus, the electric flux through the right side is +0.04 Nm²/C, while the flux through the left side is -0.04 Nm²/C. The top, bottom, front, and back sides all have an electric flux of 0 Nm²/C.

Where does the normal vector point for a spherical shell?

• A. Radially inward
• B. Radially outward
• C. Perpendicular to the radius
• D. A normal vector does not exist

Answer: (B)

Flux Through Spherical Shell due to Point Charge

To determine the electric flux through a spherical shell of radius \( R \) surrounding a point charge \( Q \) located at its center, we start with the electric flux formula, which is given by:

\[ \Phi_E = E \cdot A \cdot \cos(\theta) \]

In this scenario, the electric field \( E \) due to a point charge is directed radially outward from the charge. The normal vector of the spherical shell also points outward, meaning that the angle \( \theta \) between the electric field and the normal vector is always \( 0^\circ \). Consequently, the cosine of \( \theta \) is:

\[ \cos(0) = 1 \]

This simplifies our electric flux equation to:

\[ \Phi_E = E \cdot A \]

Next, we need to calculate the electric field \( E \) at the surface of the spherical shell. The electric field due to a point charge is expressed as:

\[ E = \frac{k \cdot Q}{R^2} \]

where \( k \) is Coulomb's constant, approximately \( 8.99 \times 10^9 \, \text{N m}^2/\text{C}^2 \). The surface area \( A \) of the spherical shell is given by:

\[ A = 4\pi R^2 \]

Substituting these values into the electric flux equation, we have:

\[ \Phi_E = \left(\frac{k \cdot Q}{R^2}\right) \cdot (4\pi R^2) \]

Upon simplifying, the \( R^2 \) terms cancel out, leading to:

\[ \Phi_E = 4\pi k Q \]

Additionally, we can express Coulomb's constant \( k \) in terms of the permittivity of free space \( \epsilon_0 \) as follows:

\[ k = \frac{1}{4\pi \epsilon_0} \]

Substituting this relationship into our expression for electric flux results in:

\[ \Phi_E = 4\pi \left(\frac{1}{4\pi \epsilon_0}\right) Q \]

After canceling the \( 4\pi \) terms, we arrive at the final expression for electric flux:

\[ \Phi_E = \frac{Q}{\epsilon_0} \]

This result is significant and will be revisited in the context of Gauss's Law, illustrating the fundamental relationship between electric flux and charge enclosed within a closed surface.

What is the total flux through the two surfaces depicted in the following figure? Note that surface 1 has an area of 50 cm² and surface 2 has an area of 100 cm² , and E = 500 N/C.

• A. 513 N•m²/C
• B. 3.74 N•m²/C
• C. 3.16 N•m²/C
• D. 5.13 N•m²/C
• E. 5.44 N•m²/C

Answer: (D)

Here’s what students ask on this topic:

What is electric flux and how is it calculated?

Electric flux is a measure of how much electric field passes through a given surface. It is analogous to the amount of water flowing through a ring. The electric flux (Φ) depends on the electric field (E), the area of the surface (A), and the angle (θ) between the electric field and the normal to the surface. The formula for electric flux is:

$\Phi =EAcos\left(\theta \right)$

What is the significance of the angle in the electric flux equation?

The angle (θ) in the electric flux equation is the angle between the electric field (E) and the normal (perpendicular vector) to the surface. This angle determines how much of the electric field passes through the surface. When θ is 0 degrees, the electric field is perpendicular to the surface, and the flux is maximized. When θ is 90 degrees, the electric field is parallel to the surface, and the flux is zero. The flux is calculated using the cosine of this angle:

$\Phi =EAcos\left(\theta \right)$

How do you calculate the total electric flux through a closed surface?

The total electric flux through a closed surface is the sum of the electric fluxes through each individual surface that makes up the closed surface. For example, if you have a box with six surfaces, you calculate the flux through each surface and then sum them up. The formula is:

$\Phi {\mathrm{total}}_{}=\sum _{}^{i=1}{\Phi }_i$

Positive flux occurs when the electric field and the normal point in the same direction, while negative flux occurs when they point in opposite directions.

What are the units of electric flux?

The units of electric flux are derived from the units of the electric field (E) and the area (A). The electric field is measured in newtons per coulomb (N/C) or volts per meter (V/m), and the area is measured in square meters (m²). Therefore, the units of electric flux are:

$\Phi =EA=\frac{N}{C}·m^2$

What is the difference between positive and negative electric flux?

Positive electric flux occurs when the electric field (E) and the normal to the surface point in the same direction. This is because the cosine of the angle (θ) between them is positive. Negative electric flux occurs when the electric field and the normal point in opposite directions, resulting in a negative cosine value. In simpler terms, if the electric field lines are exiting a surface, the flux is positive; if they are entering a surface, the flux is negative.