Electric Field: Videos & Practice Problems

Electric fields are created by charges, exerting forces on other charges within their vicinity. A positive charge generates an electric field that radiates outward, while a negative charge produces an inward field. The electric field (E) can be calculated using the equation $K^Q/r^2$. The force (F) on a charge (Q) in an electric field is given by $F=QE$. Understanding these concepts is crucial for solving problems related to electric forces and fields.

Intro to Electric Fields

Electric fields are fundamental concepts in physics that describe how charges exert forces on one another. When a charge, referred to as \( Q \), is present, it generates an electric field that extends in all directions. This electric field influences other charges placed within it, resulting in either attractive or repulsive forces, as described by Coulomb's Law.

The relationship between the force experienced by a charge and the electric field can be expressed with the equation:

\[ F = Q \times E \]

In this equation, \( F \) represents the force in Newtons, \( Q \) is the charge feeling the force (the secondary charge), and \( E \) is the electric field strength measured in Newtons per Coulomb (N/C). It is crucial to note that the charge \( Q \) used in this equation is always the one that is experiencing the force due to the electric field created by another charge.

For example, consider a scenario with a 2 Coulomb charge and a 3 Coulomb charge separated by a distance \( r \). If the electric field at the location of the 2 Coulomb charge is 10 N/C, we can calculate the force acting on it. Here, the 2 Coulomb charge is the one feeling the force, so we apply the formula:

\[ F = 2 \, \text{C} \times 10 \, \text{N/C} = 20 \, \text{N} \]

This calculation shows that the force on the 2 Coulomb charge is 20 Newtons. Understanding how electric fields operate and how to apply the relevant equations is essential for solving problems related to electric forces and fields.

A 1.5μC charge, with a mass of 50g, is in the presence of an electric field that perfectly balances its gravity. What magnitude does the electric field need to be, and in what direction does it need to point?

• A. 3.27×10⁻⁷ downwards
• B. 3.27×10² upwards
• C. 3.27×10⁴ upwards
• D. 3.27×10⁵ upwards

Answer: (D)

Electric Field due to a Point Charge

Understanding the electric field produced by point charges is essential in physics. A positive point charge generates an electric field that radiates outward, while a negative point charge creates an electric field that points inward. This convention, established long ago, helps us visualize how charges interact with their surroundings.

The electric field \( E \) created by a point charge \( Q \) at a distance \( r \) is described by the equation:

\( E = \frac{k \cdot Q}{r^2} \)

Here, \( k \) is Coulomb's constant, approximately \( 8.99 \times 10^9 \, \text{N m}^2/\text{C}^2 \). This formula indicates that the electric field strength decreases with the square of the distance from the charge. Unlike Coulomb's Law, which describes the force between two charges, this equation focuses solely on the field produced by a single charge.

When a second charge \( q \) is placed in the electric field of the first charge, it experiences a force given by:

\( F = q \cdot E \)

Substituting the expression for \( E \) into this equation reveals a direct relationship to Coulomb's Law, illustrating how the electric field and electric force are interconnected. This relationship emphasizes that both charges exert forces on each other, adhering to Newton's third law of motion.

To analyze electric fields at different points, consider two distances \( x \) and \( y \) from a charge \( Q \). The electric fields at these points can be expressed as:

\( E_x = \frac{k \cdot Q}{x^2} \)

and

\( E_y = \frac{k \cdot Q}{y^2} \)

To find the ratio of the electric fields at these two points, divide the two equations:

\( \frac{E_x}{E_y} = \frac{y^2}{x^2} \)

Taking the square root of both sides gives:

\( \sqrt{\frac{E_y}{E_x}} = \frac{x}{y} \)

This ratio allows for the comparison of electric field strengths at different distances from the charge, providing insight into how the electric field varies with distance.

For example, if \( E_y = 10 \, \text{N/C} \) and \( E_x = 20 \, \text{N/C} \), substituting these values into the ratio yields:

\( \sqrt{\frac{10}{20}} = \frac{x}{y} \)

Calculating this gives a ratio of approximately \( 0.71 \), indicating that the distance \( x \) is about 71% of the distance \( y \). This analysis illustrates the fundamental principles of electric fields and their dependence on charge and distance, essential for understanding electrostatics.

Zero Electric Field due to Two Charges

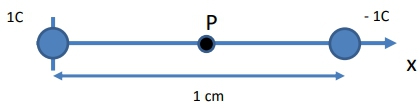
To determine the point along the X-axis where the electric field is zero due to two charges, one positive and one negative, we need to analyze the behavior of electric fields produced by these charges. Positive charges generate electric field lines that radiate outward, while negative charges create electric field lines that point inward toward the charge. In this scenario, we have a positive charge (2 Coulombs) and a negative charge (3 Coulombs) positioned on the X-axis.

We begin by identifying potential regions where the electric fields from both charges could cancel each other out. The three regions to consider are to the left of the positive charge, between the two charges, and to the right of the negative charge. In the middle region, the electric field from the positive charge points to the right, while the electric field from the negative charge also points to the right, meaning they will always add together rather than cancel out. Therefore, the middle region cannot be the location where the electric field is zero.

Next, we examine the right region. Here, the electric field from the positive charge still points outward (to the right), while the electric field from the negative charge points inward (to the left). However, due to the larger magnitude of the negative charge and its proximity, the electric field from the negative charge will always be greater than that from the positive charge, preventing cancellation in this region as well.

Thus, we focus on the left region, where it is possible for the electric fields to balance out. We denote the distance from the positive charge to the point of interest as \(X\). The condition for the electric fields to cancel is that the magnitudes of the electric fields from both charges must be equal and opposite. The electric field \(E\) due to a point charge is given by the formula:

\(E = k \frac{Q}{r^2}\

where \(k\) is Coulomb's constant, \(Q\) is the charge, and \(r\) is the distance from the charge to the point of interest. For the positive charge, the electric field at distance \(X\) is:

\(E_{+} = k \frac{2}{X^2}\

For the negative charge, the distance to the point of interest is \(X + 0.7\) meters (since the negative charge is 0.7 meters to the right of the positive charge), and its electric field is:

\(E_{-} = k \frac{3}{(X + 0.7)^2}\

Setting the magnitudes equal gives us:

\(k \frac{2}{X^2} = k \frac{3}{(X + 0.7)^2}\

We can cancel \(k\) from both sides, leading to:

\(\frac{2}{X^2} = \frac{3}{(X + 0.7)^2}\

Cross-multiplying yields:

2 \((X + 0.7)^2 = 3X^2\

Expanding and rearranging gives:

2(X^2 + 1.4X + 0.49) = 3X^2\

2X^2 + 2.8X + 0.98 = 3X^2\

0 = X^2 - 2.8X - 0.98\

To solve for \(X\), we can use the quadratic formula:

\(X = \frac{-b \pm \sqrt{b^2 - 4ac}}{2a}\

where \(a = 1\), \(b = -2.8\), and \(c = -0.98\). Plugging in these values gives:

\(X = \frac{2.8 \pm \sqrt{(-2.8)^2 - 4(1)(-0.98)}}{2(1)}\

Calculating the discriminant:

\(X = \frac{2.8 \pm \sqrt{7.84 + 3.92}}{2}\

\(X = \frac{2.8 \pm \sqrt{11.76}}{2}\

\(X = \frac{2.8 \pm 3.43}{2}\

This results in two potential solutions for \(X\): one positive and one negative. The negative solution indicates a position to the left of the positive charge. After calculations, we find:

\(X \approx -0.31\) meters, or -31 centimeters.

Thus, the point where the electric field is zero is located 31 centimeters to the left of the positive charge.

If two equal charges are separated by some distance, they form an electric dipole. Find the electric field at the center of an electric dipole, given by the point P in the following figure, formed by a 1C and a −1C charge separated by 1 cm.

• A. 1.80×10¹⁴ N/C
• B. 3.60×10¹² N/C
• C. 3.60×10¹⁴ N/C
• D. 7.19×10¹⁴ N/C

Answer: (D)

Electric Field Above Two Charges (Triangle)

In this scenario, we are analyzing the electric field created by two charges: one positive and one negative. To determine the electric field at a specific point of interest, we first need to understand the direction of the electric fields produced by each charge. The electric field generated by a positive charge points away from the charge, while the electric field from a negative charge points towards it.

To calculate the electric field, we use the formula:

$$E = k \frac{Q}{r^2}$$

where:

• E is the electric field strength,
• k is Coulomb's constant, approximately \(8.99 \times 10^9 \, \text{N m}^2/\text{C}^2\),
• Q is the charge, and
• r is the distance from the charge to the point of interest.

In this case, we have a right triangle formed by the distances from the charges to the point of interest, with one leg measuring 5 cm and the other 8 cm. Using the Pythagorean theorem, we find the hypotenuse:

$$r = \sqrt{(5^2 + 8^2)} = 9.4 \, \text{cm}$$

Given the symmetry of the problem, we can simplify our calculations. The electric fields from both charges will have equal magnitudes but opposite vertical components, which will cancel each other out. Therefore, we only need to consider the horizontal components of the electric fields.

To find the horizontal component, we use the cosine of the angle θ formed by the triangle:

$$\cos(\theta) = \frac{\text{adjacent}}{\text{hypotenuse}} = \frac{5}{9.4} \approx 0.53$$

Now, we can express the net electric field at the point of interest as:

$$E_{\text{net}} = 2 \cdot E \cdot \cos(\theta)$$

Substituting the values into the equation:

$$E_{\text{net}} = 2 \cdot \left(8.99 \times 10^9 \, \frac{\text{N m}^2}{\text{C}^2} \cdot \frac{1 \, \text{C}}{(0.094 \, \text{m})^2}\right) \cdot 0.53$$

After performing the calculations, we find that the net electric field is:

$$E_{\text{net}} \approx 1.8 \times 10^{12} \, \text{N/C}$$

This net electric field points purely in the horizontal (X) direction. Understanding the symmetry in this problem allows for a more efficient calculation of the electric field, highlighting the importance of recognizing patterns in physics problems.

4 charges are arranged as shown in the following figure. Find the magnitude of the electric field at the center of the arrangement, indicated by the point P.

• A. 8.99×10⁹ N/C
• B. 1.21×10¹⁰ N/C
• C. 3.24×10¹⁰ N/C
• D. 8.09×10¹⁰ N/C
• E. 1.94×10¹¹ N/C

Answer: (C)

Balancing a Pendulum in Electric Field

In this scenario, we analyze a charge suspended at the end of a pendulum within a uniform electric field. The key concept here is that the system is in equilibrium, meaning the sum of all forces acting on the charge in both the x and y directions equals zero. This balance of forces allows us to determine the charge at the end of the pendulum.

To find the charge \( Q \), we utilize the relationship between electric force and electric field, given by the equation:

\[ F = Q \cdot E \]

Rearranging this formula gives us:

\[ Q = \frac{F}{E} \]

Here, \( F \) represents the electric force acting on the charge, and \( E \) is the magnitude of the electric field. To calculate \( F \), we need to consider the forces acting on the charge, which include the tension in the string and the gravitational force due to the mass of the charge.

We start by drawing a free body diagram to visualize the forces. The tension \( T \) in the string can be broken down into its x and y components, where:

\[ T_x = T \cdot \cos(\theta) \]

\[ T_y = T \cdot \sin(\theta) \]

In the x-direction, the equilibrium condition can be expressed as:

\[ F_{electric} - T_x = 0 \quad \Rightarrow \quad F_{electric} = T \cdot \cos(\theta) \]

In the y-direction, the equilibrium condition is:

\[ T_y - mg = 0 \quad \Rightarrow \quad T \cdot \sin(\theta) = mg \]

From this, we can solve for the tension:

\[ T = \frac{mg}{\sin(\theta)} \]

Substituting the known values, where the mass \( m = 0.3 \, \text{kg} \) and \( g = 9.8 \, \text{m/s}^2 \), and using \( \theta = 75^\circ \), we find:

\[ T = \frac{0.3 \cdot 9.8}{\sin(75^\circ)} \approx 0.30 \, \text{N} \]

Next, we can calculate the electric force:

\[ F_{electric} = T \cdot \cos(75^\circ) \approx 0.30 \cdot \cos(75^\circ) \approx 0.078 \, \text{N} \]

Now, substituting \( F_{electric} \) back into the equation for charge:

\[ Q = \frac{0.078}{100} \approx 7.8 \times 10^{-4} \, \text{C} \]

This result gives us the charge at the end of the pendulum, demonstrating how the balance of forces in an electric field can be analyzed to find unknown quantities. Understanding these principles is crucial for solving similar problems in electrostatics and mechanics.

In the following figure, a mass m is balanced such that its tether is perfectly horizontal. If the mass is m and the angle of the electric field is θ, what is the magnitude of the electric field, E, expressed in terms of m, q, and θ?

• A. Ε = qmg/sinθ
• B. Ε = T/(q cosθ)
• C. E = q sinθ/(mg)
• D. Ε = mg/(q sinθ)
• E. E = mg/(q cosθ)

Answer: (D)

Here’s what students ask on this topic:

What is an electric field and how is it created?

An electric field is a region around a charged particle where other charges experience a force. It is created by electric charges, with positive charges generating fields that radiate outward and negative charges producing fields that point inward. The strength and direction of the electric field (E) at a point can be calculated using the equation:

$E=\frac{kQ}{r^2}$

where k is Coulomb's constant, Q is the charge, and r is the distance from the charge to the point of interest.

How do you calculate the force on a charge in an electric field?

The force (F) on a charge (Q) in an electric field (E) can be calculated using the equation:

$F=QE$

This equation states that the force is the product of the charge and the electric field. The units of the electric field are Newtons per Coulomb (N/C), and the force is measured in Newtons (N).

What is the relationship between Coulomb's law and the electric field?

Coulomb's law describes the force between two point charges, given by:

$F=\frac{kQq}{r^2}$

where k is Coulomb's constant, Q and q are the charges, and r is the distance between them. The electric field (E) created by a single charge (Q) is given by:

$E=\frac{kQ}{r^2}$

When another charge (q) is placed in this field, it experiences a force (F) given by F = qE, which is consistent with Coulomb's law.

How do positive and negative charges affect the direction of the electric field?

Positive charges create electric fields that radiate outward from the charge, while negative charges create fields that point inward toward the charge. This convention was established to standardize the direction of electric fields. The direction of the electric field is crucial for determining the force experienced by other charges placed within the field.

How do you determine the electric field at a point due to multiple charges?

To determine the electric field at a point due to multiple charges, you need to calculate the electric field contribution from each charge separately and then vectorially add them. The electric field due to a single charge (Q) at a distance (r) is given by:

$E=\frac{kQ}{r^2}$

For multiple charges, calculate the electric field from each charge at the point of interest and then add the vector components of these fields to get the resultant electric field.