Electric Field - Online Tutor, Practice Problems & Exam Prep

Electric fields are created by charges, exerting forces on other charges within their vicinity. A positive charge generates an electric field that radiates outward, while a negative charge produces an inward field. The electric field (E) can be calculated using the equation $K^Q/r^2$. The force (F) on a charge (Q) in an electric field is given by $F=QE$. Understanding these concepts is crucial for solving problems related to electric forces and fields.

Intro to Electric Fields

Hey, guys. So in this video, I want to talk about electric fields. It's something that you'll see in your textbooks. You'll need to know how to solve problems with it. But it's also to answer a very important question, which is how do charges exert forces on one another. And the basic answer is that they do this by setting up these things called electric fields. So basically a single charge, which I'll call big Q, is going to produce an electric field. You might see some confusing definitions in textbooks, but basically the idea is it's kind of like information this big Q is setting up this thing called an electric field right that exists in all directions. And then, if you place another charge at some distance, little r away from this produced electric field, then this charge will feel that field and actually have a force on it. So, in other words, it will have a repulsive or attractive force here, which we know from Coulomb's law. So basically what happens is this thing, regardless of whether there's another test charge or another secondary charge to feel it, this thing produces an electric field in all directions. And then when you place a charge inside of that field, it feels a force. And that force that it's felt with the electric field, which is set up by big Q, is just Q times E, in which E has units of Newtons per Coulomb, right? You probably don't need to know that, but there it is anyway. So E has units of Newtons per Coulomb. Okay, now which Q are we going to use in these questions? Well, that Q is always the one that's feeling the force. So the words the secondary charge right here. Now, some of you might be wondering, Whoa, isn't the opposite also happening? Doesn't this Q also produce its own electric field and therefore this one is felt by it, and the answer is yes. So this little Q also produces an electric field that is either felt as an attractive or repulsive force. And this is basically how charges exert forces on one another. You have action-reaction because of these things called electric fields. Okay, so again, I just want to reiterate in problems the Q that you're going to use inside of this F=QU equation is always going to be the one that feels the charge or the electric force. At some distance, little r okay. It's as simple as that. Let's go ahead and take a look at an example. We've got two Coulombs and three Coulombs charge. They're separated by some distance r. So I'm going to go ahead and set these up. I've got two Coulombs, three Coulombs. They're separated by a distance of little r in such a way that the electric field at this point is 10 Newtons per Coulomb. In other words, we have an electric field here at this distance. Oh, sorry, actually have it backward. So this is actually going to be the three Coulomb charge, and this is going to be the two Coulomb charge. Now, the electric field at this distance is just 10 Newtons per Coulomb. And now we have to figure out what is the force on this two Coulomb charge. What does that mean? That means that this is the Q, and this is the big Q. So it all depends on which one is the feeling charge and which one is the producing charge. So this one, the two Coulomb charge, is the feeling charge. So in order to figure out the force, we just need Q times E. So the force is going to be Q, which is two Coulombs times the electric field at this particular point, which we're told is 10 Newtons per Coulomb. And that's just equal to 20 Newtons. Okay, so that's just a simple example. Let's go ahead and get into some more examples involving electric fields.

A 1.5μC charge, with a mass of 50g, is in the presence of an electric field that perfectly balances its gravity. What magnitude does the electric field need to be, and in what direction does it need to point?

• A. 3.27×10⁻⁷ downwards
• B. 3.27×10² upwards
• C. 3.27×10⁴ upwards
• D. 3.27×10⁵ upwards

Electric Field due to a Point Charge

Hey, guys. So now that we've seen what the electric field is and how charges respond to electric fields, we're going to look a little bit more closely at the electric fields of point charges. Okay, you don't need to know that. So basically, the idea is we know that charges produce electric fields, and in previous videos, we were sort of like drawing squiggly lines here. But the reality is, instead of squiggly lines, these positive charges, positive point charges actually produce an electric field that points radially outward from it. The way I like to remember this is that positive people are very outgoing people. So this is the electric field produced by a positive charge, like one coulomb, or something like that. Whereas negative charges are just the opposite. And we just chose this convention, by the way, hundreds of years ago. So, this is just what we established as positive and negative electric fields. So, these things produce fields that point inwards. That's the main difference between them. We've got outgoing and inward fields like this, but you'll definitely need to know that now.

We saw how a single charge, whether it was like this \( Q \), or this \( \frac{Q}{r^2} \) over here, was basically producing an electric field, and that when you brought a secondary charge closer to it. So, for instance, if I brought a \( Q \) like this, then it would feel a force, whether it's in this direction or this direction, depending on the magnitude of that. Well, the magnitude of the electric field now has an equation, and it's \( k \times \frac{Q}{r^2} \). Notice how this kind of looks like Coulomb's Law, except it's missing one of the little \( Q \)s. So the main thing is that remember, the electric field is set up only just by one charge, the producing charge \( Q \). And the other thing is that this little \( r \) distance, instead of being the distance between two charges like we had in Coulomb's Law. Now it's the distance to the point of interest. So what that means is, that problem will ask you what is the electric field at a certain number, or sometimes you'll be given a diagram of charges, you'll need to figure out an electric field at a specific point. So that's how you're going to get that little \( r \) distance anyway,

So we have this equation of the electric field generated by a producing charge \( Q \). And we said that a second charge, if it's moved closer to it that little \( Q \), it felt a force from that electric field. That equation was given by \( F = Q \times E \). What we could do is basically plug in this expression. Now we can actually come up with something interesting. We know that \( E \) is just \( k \times \frac{Q}{r^2} \). So if you multiply times this \( Q \), If you recognize this formula, all this is just Coulomb's law. So this really brings the relationship between the electric field and the electric force. So a producing charge \( Q \) produces an electric field. And then when you bring a smaller charge or doesn't have to be smaller, a second charge \( Q \), they both feel a force, but the opposite is also true, So you could actually flip \( Q \) and \( Q \). One could be the producing. The other one could be the feeling charge, and they're going to be the same. That's why we have action-reaction pairs between two charges because they both exert mutual forces on each other through their electric fields. So now we get the complete picture between the relationship between Coulomb's law and the electric field. Let's go ahead and take a look at some examples right here. We've got a distance \( X \) and the electric field is some number at another distance. Here we have 10 coulombs, and now what is the ratio between \( X \) and \( Y \)? So here's what I want to do. I basically want to draw this little producing charge, which I'll call \( Q \) right here, Now at some distance away so that I'm going to call this \( X \). I'm told that the \( E \) field here \( E_X \) is equal to Newtons per Coulomb, right, And then at some other distance. So you keep going over here this other distance right here, the electric field is going to be equal to 10. By the way, this doesn't necessarily have to be half. It's not to scale or whatever. So this whole entire distance here is equal to \( Y \). And now I need to figure out what is the ratio of \( X \) and \( Y \) given those electric field intensities. Okay, so basically what happens is, I know that, at a certain point, at, for instance, point \( X \). The equation for that is going to be \( k \times \frac{Q}{X^2} \). Whereas this guy over here, the electric field at point \( Y \) is \( k \times \frac{Q}{Y^2} \). Now what happens is we actually don't know what the producing charge, what the magnitude of the producing charge is. All we need to do is just get the relationship between \( X \) and \( Y \). So to do that, you have to divide. We're going to need a ratio. Right? So what I'm going to do is I'm going to divide \( E_X \) by \( E_Y \) so you're going to get \( \frac{k \times Q}{X^2} \) all over \( \frac{k \times Q}{Y^2} \) now? The reason this is important is that we can actually cancel out the \( k \)s and the \( Q \)s that appear on both sides. Right. If you do copy dot flip. If you manipulate this equation, you're just going to get the same thing on the numerator and the denominator. So what we end up with when you do this fraction is you're going to get \( \frac{E_X}{E_Y} \) is equal to \( \frac{\frac{1}{X^2}}{\frac{1}{Y^2}} \). So this is actually going to go all the way up to the top, And it's going to be \( \frac{Y^2}{X^2} \). Okay, so now what can do is we want to get rid of this \( X^2 \) over here. So we want to square root both sides. So we're just going to get this square root. We make some room for that. We've got the square roots of \( \frac{E_X}{E_Y} \) is equal to the square roots are actually when you when you take the square root of these \( Y^2 \) and \( X^2 \), they're just going to turn into \( Y \) over \( X \). Okay, so now we've got something that kind of looks like our ratio here. We've got \( Y \) over \( X \). All we need to do is we need to flip it because we need \( X \) over \( Y \). So all we have to do is just flip both sides, and I'm going to move this over here, actually, so I get the square root of \( \frac{E_Y}{E_X} \) is going to equal \( \frac{X}{Y} \) right. So all I did was I just flip that this was the \( X \) over \( E_Y \). Now it's \( E_Y \) over \( E_X \), and I would just flip. This is well, right. So you could do that. You could just flip both sides of them cool. So I know I have to plug this stuff in, so I know that \( E_Y \) over here is 10. And I know the \( X \) is 20 Newtons per Coulomb, and that's just \( E = \frac{X}{Y} \). And if you plug that in, it's just going to be a ratio, and you should get I get 0.71. So, in other words, that is how far \( E_X \) is relative \( T.Y. \). So this is about 0.71 of the distance between this whole entire line segment here. Okay, So let me know if you guys have any questions, let's keep moving on.

Zero Electric Field due to Two Charges

Hey, guys, let's tackle this problem together. So we've got these two charges which lie on the X-axis, and we're supposed to figure out at what distance or at what point on the X-axis is the electric field going to be zero? But I want you to remember that for this problem, we're going to be using the fact that outward or positive charges produce outward-going electric field lines, they always want to go away. Whereas, negative charges produce inward-going electric field lines.

This is going to be useful because we actually have a positive charge right here and a negative charge right here. So we're going to need to remember which directions those electric fields go in. We've done these kinds of problems before when we were looking at Coulomb's law. So, in order to figure out, basically before we start plugging in numbers, we have to figure out in which region it is possible for the electric fields to cancel out. So, I've got three basic choices: I've got on the left, I've got in the middle, and I've got on the right. Now, if you look at the middle, what's going to happen at this point of interest, this point right here, the electric field from this positive Coulomb charge and the electric field lines are going to point away. So, that means that at this position right here, the electric field from that positive charge is going to be towards the right. You see, it always wants to go away. Whereas the electric field from the negative charge always wants to go towards that charge. So, that means that at this point, it wants to go to the right as well. So, basically, this is the electric field from the negative charge. And there's never going to be a situation in which these things will cancel out. They're always going to add together, no matter where I am along this line between them. So that means that it can't be in this area because they never cancel out.

So let's take a look at the right region now. We have the inward-going electric field lines, so that's going to be E_-. And from this positive charge, we have an outward-going electric field line. Now, you might be tempted to say we might be able to find a place where these things cancel out. But I want you to notice that the electric fields for any charges are just k times big Q divided by little r². So, that means it's the magnitude of the charge divided by the distance between them squared. So this three Coulomb charge is always going to be at a smaller distance from this point of interest relative to this entire distance to the weaker charge. What this means is that at this region here, the magnitude of the negative electric field is always going to be bigger than this positive electric field because it's a larger magnitude of a charge, and it's always going to be a smaller distance because this distance right here is always going to be larger and it's a weaker charge. So, the magnitude of this electric field is always going to be bigger than the magnitude of that electric field. So it's never going to cancel, so it can't be this, and instead, we're going to have to use our last scenario, which is on the left. The magnitude of the electric force goes in this direction, the direction of the electric field.

Wow, and we've got the inward-going electric field from the negative three Coulomb charge. Now, there is possibly a scenario where I could find a distance in which these will cancel out: the weaker charges at a smaller distance, the larger charges at a larger distance so there might be a way for them to balance out. The variable I'm looking for is this distance right here, which is X at what point on the X-axis is the electric field equal to zero? And what I'm going to do is I'm just going to set the origin over here to be zero. So this is just going to be X equals zero, which means I'm probably going to get a negative number, or I'm just going to have to specify 'to the left of the Coulomb charge' or something like that. The condition that I'm trying to solve for is that these things should be equal but opposite in direction to cancel out. So, in other words, the magnitude of the electric field from the positive charge should be equal and opposite to the magnitude of the negative electric field.

All right, so we know what the magnitudes of the electric fields are for This guy over here, it's going to be K. We've got the producing charge, which is the two Coulomb charge. And this is going to be at a distance of X squared. Now, that has to equal the inward-going electric field. That's going to be K times the three Coulombs. Notice how I didn't put the negative sign there because I'm just working with the magnitudes of them. So just set their magnitudes equal to each other. And now this distance right here is actually not going to be X. But it's going to be X plus 7 centimeters squared, so I could just write that. So, I've got X plus 0.07 squared. That's 7 centimeters. Just written in SI.

Right, so now what I can do is I can try to solve for this X variable over here. So, I got this equation and I could go ahead and cancel some stuff out, I got the K's. And now, in order to get this X over on one side, I have to move this thing over, and I have to move the two down, so basically, they just have to trade places. And if I do that, I'm going to just continue writing all this stuff in black because it's going to be a pain if I try to switch colors. So, I've got X plus 0.07 squared, divided by X squared equals 3/2.

Great, now, rather than starting to foil all of this mess out and getting a whole bunch of quadratic terms, things like that, what you can do is realize that both of these terms on the top and bottom are squared, which means that we can take the square root of each side. So, we're going to take the square root, take the square root, and then it just becomes X plus 0.07 over X equals the square root of 3/2. Okay, so now all we have to do is start isolating X. I'm just going to move it over to the other side. This becomes X plus 0.07 equals square root of 3/2 times X. And now all I have to do is subtract this X to the other side. So, by subtracting X, I have 0.07 equals square root of 3/2 X minus X. Now what I can do is pull this X out as a common factor because it appears in both of these terms, so basically, I have 0.07 equaling X times (square root of 3/2 minus 1). Notice how if you distribute this X back inside of both of these terms, you're just going to get back to the expression that you had right above, so it's a good shortcut to basically just pull out that greatest common factor.

Okay, and now what we could do is we could basically just move that over and divide. So we have 0.07 divided by the square root of 3/2 minus one, and if you just plug that stuff in, you should get the X distance, and you should get approximately 0.31 meters or that's just 31 centimeters. But remember we said that it's going to be to the left of that two Coulomb charge. So we just have to specify it's to the left of the two Coulomb charge. And this is the answer. So if there were like an actual coordinate system here, this would be like minus 31 centimeters anyway, but this is actually perfectly fine. If you just left it like that. Let me know if you guys have any questions. And if not, we're going to keep going with some more examples.

If two equal charges are separated by some distance, they form an electric dipole. Find the electric field at the center of an electric dipole, given by the point P in the following figure, formed by a 1C and a −1C charge separated by 1 cm.

• A. 1.80×10¹⁴ N/C
• B. 3.60×10¹² N/C
• C. 3.60×10¹⁴ N/C
• D. 7.19×10¹⁴ N/C

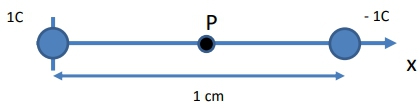
Electric Field Above Two Charges (Triangle)

Hey, guys, let's go ahead and work this one out together. We have these two charges right here, one positive one negative. And we're supposed to find out what the electric field is at this point of interest over here. All right, so let's go ahead and do that. We've got a positive charge over here. A negative charge over here. So at this point of interest, we just need to figure out without any just calculations where the electric fields are going to point. So we know from this, uh, charge right here. We know the electric field is going to point off in this direction because it's going outwards, right? It wants to point away from that charge. Whereas over here, the electric field is going to point in this direction towards the negative charge. And that's going to be e minus.

In order to figure out those electric fields, I need the formula. I've got e=kq/r2. So I need to know the distances between the source charge, or the producing charge, and the point of interest right here. Right. So I've got this little r distance over here and I have a triangle. I've got five centimeters and I've got eight centimeters here for the vertical piece. So I can figure out the hypotenuse of this triangle using the Pythagorean theorem. So you work this out, you're going to get 9.4 centimeters. And if you were to do the same thing over here, you would have to do the same exact thing. You know, five and eight, and you have to figure out the hypotenuse. So this is actually starting to look like we have a lot of symmetry in this problem. So we have five and eight, we basically have this sort of, like isosceles triangle.

So let's go ahead and see if we can use symmetry just like we did in the last video, to sort of reduce the amount of work that we have to do. So we need these same charges. So, in other words, the same magnitude of the charges we got the plus one Coulomb and the minus one Coulomb at the same distances. Which means they're going to produce the same electric field, right? And if these things are symmetrically placed around this, these two charges then that means that when we go ahead and break up these electric fields into their components, so e_x and e_y, we're going to have the angle θ. That's going to be the angle, θ is gonna help us break up these things into the components. But you're also going to have components in the Y direction and the X direction from the negative Coulomb's, the negative piece of the electric field. And that's going to be at an angle θ as well. So in other words, if these things, these test charges and the point of interest, are symmetrically placed, then we're going to actually have the same exact θ for both of them, and what that allows us to do is to eliminate the use of the vertical components. We don't have to worry about them because they're going to cancel out. And instead, what that tells us is that the net electric field is going to point off in this direction. And it's just going to be two times the electric field of the X components in which the X component of that electric field, or the X component of anything, is the magnitude times the cosine of the angle as long as your angle is relative to the X-axis.

So that means that our E_net is just going to be 2×kq/r2×cos(θ). So I have all of these numbers: k is constant, q is the charge, and r is this distance right here. This 9.4 centimeters, all I have to do is to go ahead and figure out what the cosine of this angle in this triangle is. So I have to go and use the triangle. So instead of using this angle right here, we'll have a whole bunch of stuff written out. We can also say that this angle right here is θ because these lines right here are parallel. So this is the same angle θ in the triangle and in any triangle, the way we figure out cosine of θ is if we have the angle, we can just plug it in. But if not, we can use it by relating the sides of the triangle together so we have cosine is the adjacent over hypotenuse. The adjacent side is five, the hypotenuse is 9.4.

Notice how I don't have to convert it to meters or anything like that; I could just plug it in, as meters or centimeters, because this thing is a ratio anyway. So I've got 0.53 for my cosine. So that means that I can actually just plug in this number inside for the cosine of θ. All right? And so now let's go ahead and just plug in all my numbers. I've got 2×8.99×109,1...r distance right here is going to be 0.094...and now for this cosine of θ right here, all you have to do is just substitute 0.53... If you work this out, the net electric field is going to be 1.8×1012N/C. So that's the final answer, and this net electric field points purely in the X direction.

So this is one way that you guys can use symmetry. Get very familiar with it. Watch this video a couple of times. Just so you understand how I was able to work through this symmetry and I'll see you guys in the next one, let me know if you have any questions.

4 charges are arranged as shown in the following figure. Find the magnitude of the electric field at the center of the arrangement, indicated by the point P.

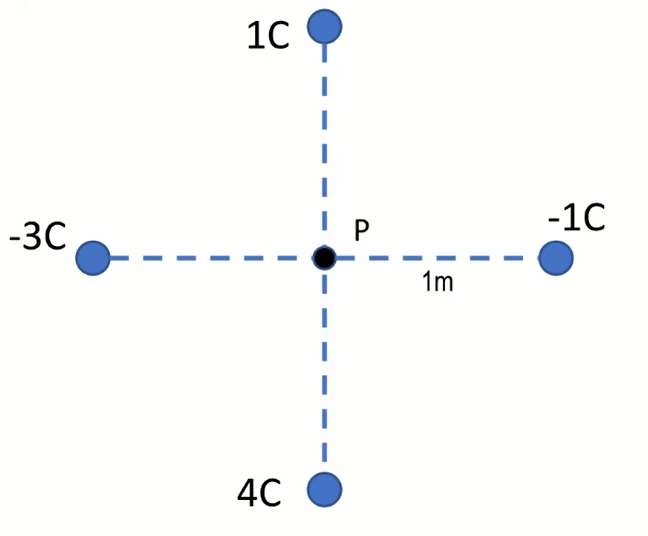
• A. 8.99×10⁹ N/C
• B. 1.21×10¹⁰ N/C
• C. 3.24×10¹⁰ N/C
• D. 8.09×10¹⁰ N/C
• E. 1.94×10¹¹ N/C

Balancing a Pendulum in Electric Field

Hey, guys, let's get more practice involving electric fields. So we have a charge that's at the end of a pendulum. And this pendulum, we're told, is in equilibrium and suspended inside of a uniform electric field. We are told what the electric field magnitude is and our job is to figure out what is the charge on the end of this pendulum? There are a couple of important things to pick out from this problem. The fact that it's in equilibrium means that the sum of all forces in the X and Y directions is equal to zero. All the forces basically cancel out. We're going to see how we can use the forces to solve this problem. But if we're solving for the charge, Q, there's basically one of two equations that we can use. We can use Coulomb's Law, but we don't have another charge, right? There's no second charge over here. So instead, what we're going to do is we're going to use the electric force is equal to Q times E, the charge times the electric field, which we actually have. So, we're going to use this formula to solve for Q. If we go and rearrange this, the Q is just equal to F divided by the electric field. If I can figure out what this electric force is, then I could basically just plug that in and solve for the charge.

To solve that force, there are a bunch of forces acting on this, so let's go ahead and draw a free body diagram for this. We have an electric field on a point charge, so we know that the force is going to be in the same direction. The reason that it doesn't just fly off is that it's held in place partly by this string that it's attached to, which has some tension. There is also another force due to gravity, because this thing has some mass, mg. Since this object is in equilibrium, which means that all the forces are going to cancel out. In order to set this up first, we have to break up this tension into its components. So we have an X and a Y component, and we get those components T_y and T_x by relating the tension with the cosine or the angle θ that it makes with the X axis. Now, this angle, if you sort of project out this horizontal line, this is a 90-degree angle, this is a 15-degree angle, which means this has to make up the difference between 180 degrees. So this angle is actually 75 degrees. So, the tension in the X direction is going to be T cos(θ) and the T in the Y direction is going to be T sin(θ).

Now we have these components, let's go ahead and set up our equilibrium conditions in the X direction. I have that the sum of all forces in the X direction is equal to zero. So, starting off my forces in the X direction, I have the electric force, which is really what I'm looking for, minus the tension or the X component of the tension. In other words, the electric force here is just equal to the tension times the cosine of the angle. The problem is, I know what the cos(θ) is, I don't know what the tension is. So remember, think back to Newton's laws, whenever we had a situation where we needed one variable in the X direction, we usually went to the Y direction to solve for it. So, we are going to do that. In the Y direction, we have the same exact condition, the sum of all forces in the Y direction is equal to zero. Your T_y, which is going to be your T sin(θ), is equal to mg once you move this to the other side. So now, T is actually equal to mg divided by the sin(θ), and I actually have all these numbers: the mass, g and also sin(θ). So, T equals mg/sin(θ) gives you 0.30 Newtons. Now you can just take this number and then plug it back inside of this formula. The electric force is just equal to 0.30 Newtons times the cosine of the angle, which is 75 degrees. So that means that my electric force is equal to 0.78 Newtons. So now what I can do is I can take this electric force and I just have to plug it back into my initial equation to solve for the charge. Remember, that's my final variable. So, Q is equal to 0.78 divided by 100 Newtons per Coulomb. You should get 7.8 times 10^-4 Coulombs. This is, by the way, our final answer.

Let me know what you think about this problem. Let me know if you have any questions. I'll happily explain any steps, and I'll see you guys in the next one.

In the following figure, a mass m is balanced such that its tether is perfectly horizontal. If the mass is m and the angle of the electric field is θ, what is the magnitude of the electric field, E, expressed in terms of m, q, and θ?

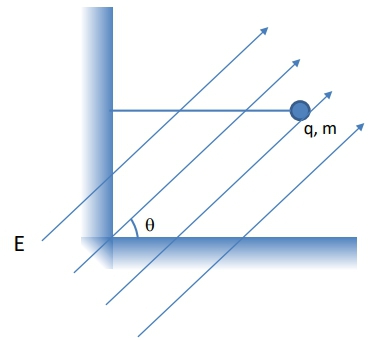
• A. Ε = qmg/sinθ
• B. Ε = T/(q cosθ)
• C. E = q sinθ/(mg)
• D. Ε = mg/(q sinθ)
• E. E = mg/(q cosθ)

Here’s what students ask on this topic:

What is an electric field and how is it created?

An electric field is a region around a charged particle where other charges experience a force. It is created by electric charges, with positive charges generating fields that radiate outward and negative charges producing fields that point inward. The strength and direction of the electric field (E) at a point can be calculated using the equation:

$E=\frac{kQ}{r^2}$

where k is Coulomb's constant, Q is the charge, and r is the distance from the charge to the point of interest.

How do you calculate the force on a charge in an electric field?

The force (F) on a charge (Q) in an electric field (E) can be calculated using the equation:

$F=QE$

This equation states that the force is the product of the charge and the electric field. The units of the electric field are Newtons per Coulomb (N/C), and the force is measured in Newtons (N).

What is the relationship between Coulomb's law and the electric field?

Coulomb's law describes the force between two point charges, given by:

$F=\frac{kQq}{r^2}$

where k is Coulomb's constant, Q and q are the charges, and r is the distance between them. The electric field (E) created by a single charge (Q) is given by:

$E=\frac{kQ}{r^2}$

When another charge (q) is placed in this field, it experiences a force (F) given by F = qE, which is consistent with Coulomb's law.

How do positive and negative charges affect the direction of the electric field?

Positive charges create electric fields that radiate outward from the charge, while negative charges create fields that point inward toward the charge. This convention was established to standardize the direction of electric fields. The direction of the electric field is crucial for determining the force experienced by other charges placed within the field.

How do you determine the electric field at a point due to multiple charges?

To determine the electric field at a point due to multiple charges, you need to calculate the electric field contribution from each charge separately and then vectorially add them. The electric field due to a single charge (Q) at a distance (r) is given by:

$E=\frac{kQ}{r^2}$

For multiple charges, calculate the electric field from each charge at the point of interest and then add the vector components of these fields to get the resultant electric field.