Solving Capacitor Circuits: Videos & Practice Problems

In capacitor circuits, series connections share the same charge, while parallel connections share the same voltage. The equivalent capacitance for series is calculated using the inverse sum rule, while for parallel, it’s a simple sum. To find charge (Q) and voltage (V), use the relationship Q = C × V. For example, in a circuit with a 10V battery and capacitors, the equivalent capacitance can be determined, followed by calculating the charge and voltage across each capacitor. Understanding these principles is essential for solving complex capacitor circuit problems.

Solving Capacitor Circuits

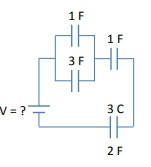
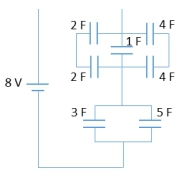
Understanding capacitor circuits involves mastering the concepts of equivalent capacitance, charge, and voltage across capacitors in both series and parallel configurations. In series connections, the equivalent capacitance can be calculated using the inverse sum rule, which states that the inverse of the equivalent capacitance (\(C_{EQ}\)) is equal to the sum of the inverses of the individual capacitances:

\[ \frac{1}{C_{EQ}} = \frac{1}{C_1} + \frac{1}{C_2} + \ldots + \frac{1}{C_n} \]

In a series circuit, all capacitors share the same charge. For example, if one capacitor has a charge of 3 coulombs, all capacitors in that series will also have 3 coulombs. This shared charge is crucial for analyzing the circuit.

On the other hand, in parallel connections, the equivalent capacitance is simply the sum of the individual capacitances:

\[ C_{EQ} = C_1 + C_2 + \ldots + C_n \]

In parallel circuits, capacitors share the same voltage across their terminals. For instance, if one capacitor has a voltage of 2 volts, all capacitors in that parallel configuration will also have 2 volts across them. This distinction between charge and voltage sharing is essential for solving problems involving mixed configurations of capacitors.

To solve capacitor circuit problems, follow a systematic approach. First, determine the equivalent capacitance of the entire circuit. Once you have \(C_{EQ}\), you can find the total charge (\(Q\)) using the relationship:

\[ Q = C \times V \]

Where \(V\) is the total voltage supplied by the battery. After calculating the total charge, you can work backwards to find the charge and voltage across each individual capacitor. For capacitors in series, remember that they all have the same charge, while for capacitors in parallel, they will have the same voltage.

For example, if you have a circuit with a 6 farad capacitor in series with a parallel combination of a 1 farad and a 2 farad capacitor, you would first calculate the equivalent capacitance of the parallel capacitors (which would be 3 farads), then find the total equivalent capacitance of the series combination (which would be 2 farads). With the total voltage known, you can find the total charge and subsequently the individual voltages and charges across each capacitor.

By organizing your calculations and keeping track of the relationships between charge, capacitance, and voltage, you can effectively analyze and solve complex capacitor circuit problems.

What is charge and voltage across each capacitor below?

• A. Q_2F=10 C, V_2F=10 V,
  Q_3F=20 C, V_3F=10 V
• B. Q_2F=9.8 C, V_2F=5 V,
  Q_3F=7.3 C, V_3F=10 V
• C. Q_2F=4.9 C, V_2F=10 V,
  Q_3F=7.3 C, V_3F=10 V
• D. Q_2F=10 C, V_2F=5 V,
  Q_3F=30 C, V_3F=10 V

Answer: (D)

Find Charge of One Capacitor (Simple)

In analyzing a capacitor circuit, it's essential to understand the relationship between capacitance, voltage, and charge. The charge \( Q \) on a capacitor can be calculated using the formula:

\( Q = C \cdot V \)

where \( C \) is the capacitance in farads and \( V \) is the voltage in volts. In this scenario, we are particularly interested in the charge on a 3 farad capacitor within a circuit powered by a 5-volt battery.

One key point to note is that the position of the battery in the circuit does not affect the overall behavior of the circuit. Whether the battery is placed at the top or in the middle, the voltage across the capacitors remains the same. This means that if we have capacitors arranged in parallel, they will all experience the same voltage across them.

In this case, since the 3 farad capacitor is in parallel with other capacitors, it shares the same voltage of 5 volts. Therefore, we can directly apply the formula to find the charge:

\( Q = 3 \, \text{F} \cdot 5 \, \text{V} = 15 \, \text{C} \)

This calculation shows that the charge on the 3 farad capacitor is 15 coulombs. Understanding these principles allows for efficient problem-solving in capacitor circuits without needing to simplify the entire circuit into a single equivalent capacitor.

What is the voltage of the battery below?

• A. V = 0.80 V
• B. V = 1.75 V
• C. V = 5.25 V
• D. V = 15.8 V

Answer: (C)

What is the charge on the 5 F capacitor? (hint:be careful with series vs parallel)

• A. Q₅=2.6 C
• B. Q₅=9.5 C
• C. Q₅=13.4 C
• D. Q₅=14.4 C

Answer: (C)

Here’s what students ask on this topic:

How do you calculate the equivalent capacitance of capacitors in series?

To calculate the equivalent capacitance (C_eq) of capacitors in series, you use the inverse sum rule. The formula is:

$\frac{1}{(\frac{1}{C_1}+\frac{1}{C_2}+...+\frac{1}{C_{\mathrm{n}}})}$

Where C₁, C₂, ..., C_n are the capacitances of the individual capacitors. The equivalent capacitance is always less than the smallest individual capacitor in the series.

How do you calculate the equivalent capacitance of capacitors in parallel?

To calculate the equivalent capacitance (C_eq) of capacitors in parallel, you simply sum the capacitances of the individual capacitors. The formula is:

$C_{\mathrm{eq}}=C_1+C_2+...+C_{\mathrm{n}}$

Where C₁, C₂, ..., C_n are the capacitances of the individual capacitors. The equivalent capacitance is always greater than the largest individual capacitor in the parallel arrangement.

What is the relationship between charge, capacitance, and voltage in a capacitor circuit?

The relationship between charge (Q), capacitance (C), and voltage (V) in a capacitor circuit is given by the equation:

$Q=C\times V$

This means that the charge stored in a capacitor is equal to the product of its capacitance and the voltage across it. This relationship is fundamental in analyzing and solving capacitor circuit problems.

How do capacitors in series and parallel share charge and voltage?

In a series connection, capacitors share the same charge (Q) but have different voltages (V) across them. The total voltage is the sum of the individual voltages. In a parallel connection, capacitors share the same voltage (V) but have different charges (Q) stored in them. The total charge is the sum of the individual charges. Understanding these principles is crucial for solving complex capacitor circuit problems.

How do you find the charge and voltage across each capacitor in a mixed series-parallel circuit?

To find the charge and voltage across each capacitor in a mixed series-parallel circuit, follow these steps:

1. Calculate the equivalent capacitance (C_eq) of the entire circuit.
2. Determine the total charge (Q) using Q = C_eq × V, where V is the total voltage.
3. Work backwards to find the charge and voltage for each capacitor, using the rules for series and parallel connections.

For series, capacitors share the same charge. For parallel, capacitors share the same voltage. This step-by-step process helps in solving for the desired variables in the circuit.