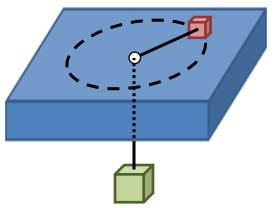
Hey, guys. So here's another classic example of conservation of angular momentum. And here we have this weird setup where you have an object, a little block that spins on a horizontal table. So imagine you got a table and you have an object spinning on top of it, but there's a tiny little hole through the table and the object spins because it's being pulled by a string that is pulled through that hole. You might be wondering what's up with that setup. Right? The reason we have these weird setups is because we need a way to change the length of the cable which will in turn change how quickly the block on top spins. The block at the bottom is just being used to hold and give this cable some tension. So this guy here is being pulled by mg. And therefore, there's a tension here, which provides the centripetal force for this guy here to spin. OK. So that's the standard setup. And basically, what's going to happen in these problems is the length of the cable will get reduced. Which means the distance of rotation around the central axis will get reduced, which means the omega will go up. The object will spin faster and faster.
Let's check this out. It says here we have a small object. The red one over here has a mass of 2 kilograms. It is on a smooth table, no friction attached to a light string, no mass on the string, like always, that runs through a hole in the table. The other end of the string attaches to a hanging weight, this guy over here, m2. So m1 is 2 and m2 doesn't get mentioned yet. It says when the small object is given some speed, so you give this guy some velocity, the v, by the way. It's tangential to the circle. I drew it this way. It doesn't mean that it's flying off the table. It's just going this way on the table. Right? So they have some velocity. Maybe I should draw it sort of like this. It doesn't look like it's going away.
Alright. So when it's given some speed, it spins around a circular path, around the hole with the tension from the hanging weights providing a centripetal force. I explained that this mass here pulls down which, the block pulls on the rope, providing the tension. And this tension is what keeps you spinning because it provides the force that keeps you around the circle. It's supposed that the object spins at 120 RPM when the radial distance, when it has a radial distance of 10 centimeters from the hole. So when this distance l or r is 0.10 or 0.1, it has an RPM of 120. So this is how the problem starts. Therefore, I'm gonna call this my r initial and my RPM initial.
So the first question is how fast in RPM would it spin if the radial distance was reduced to 6? In other words, if our final is instead of 10, it is 6. So 0.06 because it's got to be meters. What would be my RPM final? OK. So that's part a. So let's do this real quick. Conservation of angular momentum, l_initial equals l_final. I'm going to expand this to be Iω = I0ω initial_final_final_final_final. This object is a small object, which means we're gonna treat it as a point mass. So instead of I, we're going to expand I into mr². And notice that I have RPMs. So I don't want omegas. I want RPMs. Remember that ω is 2πRPM/60. So I'm going to rewrite ω, expand ω as 2πRPM_initial/60. This is mass initial, though the masses don't really change in the radius initial or the distance initial. On the other side, I have the same thing, but it's gonna be m_final r_final² and then 2πRPM_final, which is the target variable, by the way, divided by 60. When I write this, notice that I can cancel some stuff. First, the mass isn't chained. So this mass cancels with this mass. The 2π cancels with 2π, 60 cancels with 60. So we're left with rI² RPM_initial equals r_final² RPM_final. And this is what we're looking for. So I can solve for RPM_final by moving everything, by moving this guy to the other side. So it's gonna be RPM_final is rI² / r_final² RPM_initial.