An ornamental structure consists of a hinged rod and a supporting wire. Determine the tension (T) in the supporting cable and the magnitude and direction of the force exerted on the rod by the hinge when you hang a decoration that has twice the weight as the supporting rod, w. A free-body diagram is helpful.

An advertising sign of mass 2 kg hangs from the right end of a uniform aluminum bar. The bar of mass 8 kg and length 2 m, held in equilibrium, is attached to a wall by a supporting cord, as shown in the image. Find the tension in the cord.

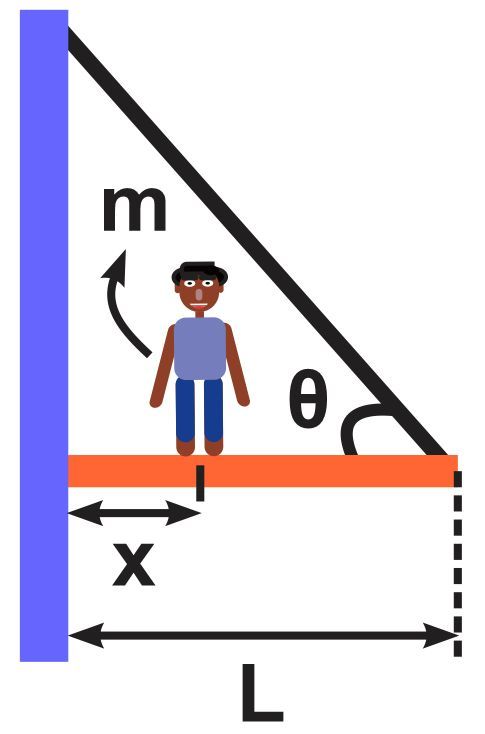
A diving board of uniform density has a mass M and length L, is fixed at one end to the pool deck. It is supported by a wire at the other end, which is attached to a point on the deck making an angle $\theta$ with the horizontal. A diver of mass m walks to the end of the board, which is a distance x from the fixed end. Determine, as a function of x, the tension in the wire required to keep the board level.

Cranes lift objects using the boom. A 10,000 N boom is pivoted about a frictionless axle at its lower end. A cable attached at 5.0 m from the upper end making an angle of 18° is used to support the boom. The boom is 25 m long and non-regular. The center of gravity is located 11 m from the axle measured parallel to the boom. The boom is directed at 60° above the horizontal, suspending a 4500 N container using a 1.8 m long massless rope. What is the tension in the cable?

A 33,000 kg dump truck carrying gravel is passing across an 88.0 m flyover span from north to south. The flyover span can be considered a uniform structure of mass 200,000 kg. The center of mass of the truck is moving at a constant speed of 65.0 km/h. Determine the magnitude of the vertical force F_s(t) acting on the south end of the span during the travel of the truck as a function of time. (Assume at t = 0, the center of mass of the truck is at the north end on which the force F_n(t) acts.)

A uniform 87-kg column is held still by a cable that is attached to it at a point that is 3/4 of its length from the ground. The other end of the cable is attached to the ground which makes an angle of 33° with the horizontal. Given that the length of the column is 16 m and the angle between the column and the ground is 83°, calculate the tension in the cable.

A female worker in the workplace has a safe limit to lift objects weighing 160 N (16 kg) at places of work. The worker can lift weights greater than 160 N using a trolley. You may consider a 1.5 m long trolley, weighing 100 N, and the center of gravity located at 0.6 m from the axle. Assume the load's center of mass is located 0.6 m from the axle. What is the source of the force that enables the worker to lift more weight using the trolley?

Consider a long thin wooden slab 5m in length. The slab is of negligible mass and is in equilibrium. The forces acting on it are shown in the figure below. Find the magnitudes of forces F₁ and F₂.

The limb in a cast weighs 16.0 kg and its centre of gravity is 36.0 cm from the pivot point. Calculate the mass required to balance the limb if the suspension cord is attached 79.0 cm from the pivot point.