Everyone, in this problem, we're going to calculate the total pressure when we have 2 liquids. There's something a little bit different about this problem. Hopefully, you guys try it on your own. Let's check it out. We have a tall cylindrical beaker that's 10 centimeters in radius. In other words, the radius of this cylinder, I'm going to say this is r=0.1m. We're going to mix 2 different liquids inside. We're going to pour 5 liters of a liquid with a density of 8000 kg/m3, and then 10 liters of a liquid with a density of 6000 kg/m3. Remember, when you have 2 different densities, the higher one's going to settle towards the bottom. So, to simplify, I'm going to call these liquids 1 and 2.
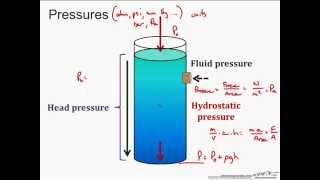
To calculate the total pressure at the bottom of the beaker, termed Pbottom, we begin with the equation Pbottom=Ptop+ρgh, starting from the top of the liquids and considering that Ptop is equal to the standard air pressure. This allows us to account for the pressure added by each liquid layer:
Pbottom=Ptop+ρ1gh1+ρ2gh2
To directly determine the heights h1 and h2 from the known volumes of the liquids, we use the cylinder volume formula and rearrange it to find each height:
h1= 10 liters divided by πr2 , h2= 5 liters divided by πr2Using correction factors to deal with units, heights h1 and h2 are calculated to be 0.32 meters and 0.16 meters, respectively.
Thus, the final calculation for Pbottom becomes:
Pbottom=101,000+6000×9.8×0.32+8000×9.8×0.16
This calculation shows how the pressure at the bottom of the beaker is 1.33×105 Pascals, illustrating the substantial impact of the densities on the pressure in even a small amount of liquid. That's it for this one, folks. Let me know if you have any questions.