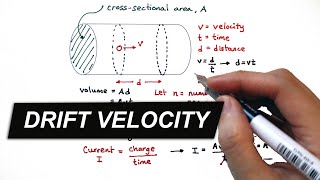
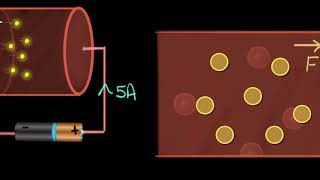
Idea is the following: when we have a wire. All right, our wire looks like this, it's got a cross-sectional area of A, it's got some length associated with it L. And when current flows down the wire, current I, we know what that means. Right? What that means is that little electrons in there are actually drifting to the left. They're actually bouncing around all over the place but there is some net drift V sub d to the left. Okay? And that's what's called the drift velocity. Okay, they actually bounce up and down a lot and move slowly to the left as we're gonna see the drift velocity can be rather slow. So, how do we calculate this drift velocity? Well, let's go back to the idea of delta Q, right? Delta Q is the amount of charge that's going to move. And if I think about what that really means is, it's the number of charges N times the charge of each particle Q Okay? This is the number of charges and that's the charge on each one. All right. But, the number of charges we can write as something else. We can write the number of charges as a number: density times a volume. And this n is the number density. It's the number per unit volume. And this is of course, the volume of our sample. All right. Q is the charge on each one and that is, of course, one electron. Okay, and we know what that is, that's 1.6 times 10 to the minus 19 coulombs. And, it's got a negative sign on it. Okay. If that is the amount of charge, can we write it in terms of things that we know here like the drift velocity? And, let's redraw this picture and talk about that. Here is a portion of our wire: has cross-sectional area A and it has a length l. L is related to the drift velocity because if I think about an electron moving along at Vd, it's going to cover l in an amount of time delta t. And so, that distance l is just the drift velocity times delta t. Alright. But if I do that, then I can calculate the volume of this cylinder. The volume is just the area times the length. And so, the volume becomes A times Vd delta t. And now, we can put that into this equation over here. We said the number of charges N is equal to number density times volume. And so, it looks like it's just n times A times Vd delta t. And now, we can write all this in terms of the current. I we said was: delta Q over delta t. And Delta Q we said was: N -- little n AVd delta t. And we're going to multiply by the charge of the electron. Don't worry if it's positive or negative. And then, we're gonna divide by delta t. And so, look what happens. The delta t cancels out and we get a nice little relationship. Current is equal to nAV sub D times e. Okay, this is the current in the wire. Depends on the number density. It depends on the cross-sectional area. It depends on the drift velocity and it depends on the charge on that electron. Don't worry if this is positive or negative we're just talking about magnitudes here. All right. With this information, can we attack problem 1855? So, let's take a look at 1855. In my problem it says the following: I have a wire that has a particular diameter, d. And, my number is d equals 0. millimeters. And, it says it carries a current of 2.3 micro amps. The molar mass of copper is 600. Oh. 63.5 grams per mole. Okay? So, there is some mass density which is 63.5 grams per mole. And, it's I'm sorry that's the -- that's the molar mass which I think they were using Rho sub D for that. And then, the mass density is 89 hundred kilograms per cubic meter. And then finally, of course, they tell us Avogadro's number, which is 6.02 times 10 to the 23. And that is the particles in a mole. Okay. Do we have enough information to solve this thing for the drift velocity? Well, we have I. We have the current. We don't really have A yet. But, they give us a diameter so we can probably calculate A. We, of course, know the electron charge. We're solving for V sub d -- the drift velocity. And what is not really clear yet is can we get this number density n? All right. Let's rewrite this equation and we want to solve for drift velocity. So let's do that. V sub d is going to be I divided by little n times A times e. And now, we have to worry about what these different things are. Okay. Little n. Little n is going to be how many charges there are in a given volume. We know how many charges are in one mole. So if we can calculate the volume of one mole, then we'll have it. And, the volume of one mole is going to be related to the mass density and the molar density. And so, it's going to be 8900 kilograms. And then, we have to have 63.5 grams per mole. That's not really SI unit. So, let's convert this, 63.5 times 10 to the minues 3 kilograms per mole. Anything else not in SI units, yeah current. Right? 2.3 times 10 to the minus 6 amps. d is also not, it's 0.65 times ten to the minus 3 meters. And then, everything else is good. Okay. So, down in the bottom we're gonna need this 63. times 10 to the minus kilograms per mole. That's gonna go right there. And then, dividing that we're gonna need the mass density, rho sub mass. And let's plug in some numbers here and let's see if the units work out right. Okay. So, we've got 6.02 times 10 to the 23. And then rho d, we've got 63.5 times 10 to the minus kilograms per mole And we're going to divide by kilograms per cubic meter. And, it looks like the kilograms are gonna cancel out and we're going to end up with something per cubic meter. And so, somebody punch those numbers into your calculator and tell me what we get. And, it looks like the units are gonna work out just fine. Okay. So, that is our little number n. What about A? A is, of course, the cross-sectional area of the wire which is pi r squared, which is pi times d over 2 quantity squared. And, we know what d is. So, this becomes pi times 0.65 times 10 to the minus 3. And, we're gonna divide that by 2 and we're gonna square that whole thing. And if somebody gets a number for that one, let me know. Would -- anybody get a number for this one? >> (student speaking) 8.4. >> 8.4. >> (student speaking) Times 10 to the 28. times 10 to the 28. And the units are meters to the minus 3. Right? That's good because this is number density so it should be number per volume. Okay. Can everybody see that okay on the right side of the board? Okay. Let me know if I go off-screen over there . And now, what about this one right here? Anybody get a number for that, the area? >> (student speaking) 3.3 times 10 to the negative 7. Somebody else confirm that? That it sounds about right. And then, that's gonna be square meters. Okay. And so now, we should be able to put all of this stuff in for the drift velocity. So we get: Drift velocity is current over nAe which is 2.3 times 10 to the minus 6, divided by the number density which is 8.4 times 10 to the 28. A we said was 3. times ten to the minus seven. And, e is, of course, 1. times 10 to the minus 19. So, somebody run those numbers in your calculator. I'll approximate it here. Let's see. We've got 8 times 3. That's 8.4 times 3. That's got to be close to 30, times 1.5. That's got to be around 45. So, we've got 2 over 45. 2.3 over 45, which is like 2 over 40. And then, we've got 10 to the what? Down on the bottom we've got 28, we're gonna subtract 7, so we get 21. And then, we're going to subtract 19 so we get a 10 to the 2. Is that right? And if we subtract 2 from that 1, we should get 10 to the minus 8. So, 2 over 40 is pretty close to 0. times 10 to the minus 8. So, I'm going to say this is about 4 times 10 to the minus 10. Anybody get a real number? >> (student speaking) 5.2. 5? 5.2 times 10 to the minus 10. Okay. And, that is SI units meters per second. Okay? Let's try it, see if we're right. There's a lot of numbers here. 5. and then we got to do our times 10 to the minus 10. And, the units are meters per second. Should we click submit? Let's try it. We got the green box. Correct. All right. It's very exciting when you get that green box isn't it? Okay. 5.2 times 10 to the minus 10 meters per second. If you're still having trouble with this one, go to the book and look at example because it does this problem nearly explicitly right there. So, is this a big number or a small number? It says that that electron is going to drift at 5.2 times 10 to the minus 10 meters per second. That's barely moving at all. Right? If I had to go one meter, it would take me like 10 to the 10 seconds to go one meter. So, does that mean when you turn on your light bulb, that it's going to take forever for the light bulb to actually turn on because those electrons have to travel all the way from the switch up to the light bulb? No. That's not what happens. Right? What happens? Why does your light bulb turn on right away when you turn that switch on? This is another one of your homework problems, by the way. So, a wire, of course, has atoms everywhere. So if I put an electron right there, there's gonna be another electron right next to it and another one right next to it, and another one right next to it. So, that first electron that's going to move, all it has to do is bump into the next one And if it bumps into the next one, then that one bumps into the next one and that one bumps into the next one. And eventually, one of those is going to light up your light bulb. And that happens very quickly. Okay? Electric signals travel fast. Okay. They are pretty close to the speed of light, C. When you turn on the switch, that signal propagates down the wire at nearly the speed of light, even though every individual electron doesn't move very fast at all. Okay? Really, really slowly. And so when you turn on the switch, that last electron by the filament that gets pushed hits the next one, hits the next one. All of those heat up in the filament create light. So, you don't have to send that electron all the way to the filament. It's going to take a long time to get to the filament, but the electrical signal is gonna propagate very quickly. Okay. Any questions about that one? Did you guys find that one challenging? Hopefully, that was supposed to be one of the more challenging ones on the homework. If you're totally bored, let me know and we'll get some harder homework problems. No problem.
Table of contents
- 0. Math Review31m
- 1. Intro to Physics Units1h 23m
- 2. 1D Motion / Kinematics3h 56m
- Vectors, Scalars, & Displacement13m
- Average Velocity32m
- Intro to Acceleration7m
- Position-Time Graphs & Velocity26m
- Conceptual Problems with Position-Time Graphs22m
- Velocity-Time Graphs & Acceleration5m
- Calculating Displacement from Velocity-Time Graphs15m
- Conceptual Problems with Velocity-Time Graphs10m
- Calculating Change in Velocity from Acceleration-Time Graphs10m
- Graphing Position, Velocity, and Acceleration Graphs11m
- Kinematics Equations37m
- Vertical Motion and Free Fall19m
- Catch/Overtake Problems23m
- 3. Vectors2h 43m
- Review of Vectors vs. Scalars1m
- Introduction to Vectors7m
- Adding Vectors Graphically22m
- Vector Composition & Decomposition11m
- Adding Vectors by Components13m
- Trig Review24m
- Unit Vectors15m
- Introduction to Dot Product (Scalar Product)12m
- Calculating Dot Product Using Components12m
- Intro to Cross Product (Vector Product)23m
- Calculating Cross Product Using Components17m
- 4. 2D Kinematics1h 42m
- 5. Projectile Motion3h 6m
- 6. Intro to Forces (Dynamics)3h 22m
- 7. Friction, Inclines, Systems2h 44m
- 8. Centripetal Forces & Gravitation7h 26m
- Uniform Circular Motion7m
- Period and Frequency in Uniform Circular Motion20m
- Centripetal Forces15m
- Vertical Centripetal Forces10m
- Flat Curves9m
- Banked Curves10m
- Newton's Law of Gravity30m
- Gravitational Forces in 2D25m
- Acceleration Due to Gravity13m
- Satellite Motion: Intro5m
- Satellite Motion: Speed & Period35m
- Geosynchronous Orbits15m
- Overview of Kepler's Laws5m
- Kepler's First Law11m
- Kepler's Third Law16m
- Kepler's Third Law for Elliptical Orbits15m
- Gravitational Potential Energy21m
- Gravitational Potential Energy for Systems of Masses17m
- Escape Velocity21m
- Energy of Circular Orbits23m
- Energy of Elliptical Orbits36m
- Black Holes16m
- Gravitational Force Inside the Earth13m
- Mass Distribution with Calculus45m
- 9. Work & Energy1h 59m
- 10. Conservation of Energy2h 54m
- Intro to Energy Types3m
- Gravitational Potential Energy10m
- Intro to Conservation of Energy32m
- Energy with Non-Conservative Forces20m
- Springs & Elastic Potential Energy19m
- Solving Projectile Motion Using Energy13m
- Motion Along Curved Paths4m
- Rollercoaster Problems13m
- Pendulum Problems13m
- Energy in Connected Objects (Systems)24m
- Force & Potential Energy18m
- 11. Momentum & Impulse3h 40m
- Intro to Momentum11m
- Intro to Impulse14m
- Impulse with Variable Forces12m
- Intro to Conservation of Momentum17m
- Push-Away Problems19m
- Types of Collisions4m
- Completely Inelastic Collisions28m
- Adding Mass to a Moving System8m
- Collisions & Motion (Momentum & Energy)26m
- Ballistic Pendulum14m
- Collisions with Springs13m
- Elastic Collisions24m
- How to Identify the Type of Collision9m
- Intro to Center of Mass15m
- 12. Rotational Kinematics2h 59m
- 13. Rotational Inertia & Energy7h 4m
- More Conservation of Energy Problems54m
- Conservation of Energy in Rolling Motion45m
- Parallel Axis Theorem13m
- Intro to Moment of Inertia28m
- Moment of Inertia via Integration18m
- Moment of Inertia of Systems23m
- Moment of Inertia & Mass Distribution10m
- Intro to Rotational Kinetic Energy16m
- Energy of Rolling Motion18m
- Types of Motion & Energy24m
- Conservation of Energy with Rotation35m
- Torque with Kinematic Equations56m
- Rotational Dynamics with Two Motions50m
- Rotational Dynamics of Rolling Motion27m
- 14. Torque & Rotational Dynamics2h 5m
- 15. Rotational Equilibrium3h 39m
- 16. Angular Momentum3h 6m
- Opening/Closing Arms on Rotating Stool18m
- Conservation of Angular Momentum46m
- Angular Momentum & Newton's Second Law10m
- Intro to Angular Collisions15m
- Jumping Into/Out of Moving Disc23m
- Spinning on String of Variable Length20m
- Angular Collisions with Linear Motion8m
- Intro to Angular Momentum15m
- Angular Momentum of a Point Mass21m
- Angular Momentum of Objects in Linear Motion7m
- 17. Periodic Motion2h 9m
- 18. Waves & Sound3h 40m
- Intro to Waves11m
- Velocity of Transverse Waves21m
- Velocity of Longitudinal Waves11m
- Wave Functions31m
- Phase Constant14m
- Average Power of Waves on Strings10m
- Wave Intensity19m
- Sound Intensity13m
- Wave Interference8m
- Superposition of Wave Functions3m
- Standing Waves30m
- Standing Wave Functions14m
- Standing Sound Waves12m
- Beats8m
- The Doppler Effect7m
- 19. Fluid Mechanics2h 27m
- 20. Heat and Temperature3h 7m
- Temperature16m
- Linear Thermal Expansion14m
- Volume Thermal Expansion14m
- Moles and Avogadro's Number14m
- Specific Heat & Temperature Changes12m
- Latent Heat & Phase Changes16m
- Intro to Calorimetry21m
- Calorimetry with Temperature and Phase Changes15m
- Advanced Calorimetry: Equilibrium Temperature with Phase Changes9m
- Phase Diagrams, Triple Points and Critical Points6m
- Heat Transfer44m
- 21. Kinetic Theory of Ideal Gases1h 50m
- 22. The First Law of Thermodynamics1h 26m
- 23. The Second Law of Thermodynamics3h 11m
- 24. Electric Force & Field; Gauss' Law3h 42m
- 25. Electric Potential1h 51m
- 26. Capacitors & Dielectrics2h 2m
- 27. Resistors & DC Circuits3h 8m
- 28. Magnetic Fields and Forces2h 23m
- 29. Sources of Magnetic Field2h 30m
- Magnetic Field Produced by Moving Charges10m
- Magnetic Field Produced by Straight Currents27m
- Magnetic Force Between Parallel Currents12m
- Magnetic Force Between Two Moving Charges9m
- Magnetic Field Produced by Loops and Solenoids42m
- Toroidal Solenoids aka Toroids12m
- Biot-Savart Law (Calculus)18m
- Ampere's Law (Calculus)17m
- 30. Induction and Inductance3h 37m
- 31. Alternating Current2h 37m
- Alternating Voltages and Currents18m
- RMS Current and Voltage9m
- Phasors20m
- Resistors in AC Circuits9m
- Phasors for Resistors7m
- Capacitors in AC Circuits16m
- Phasors for Capacitors8m
- Inductors in AC Circuits13m
- Phasors for Inductors7m
- Impedance in AC Circuits18m
- Series LRC Circuits11m
- Resonance in Series LRC Circuits10m
- Power in AC Circuits5m
- 32. Electromagnetic Waves2h 14m
- 33. Geometric Optics2h 57m
- 34. Wave Optics1h 15m
- 35. Special Relativity2h 10m
27. Resistors & DC Circuits
Microscopic View of Current
Video duration:
18mPlay a video:
Related Videos
Related Practice