Frost Circle: Study with Video Lessons, Practice Problems & Examples

Hückel's rule identifies stable aromatic compounds with 2, 6, 10, or 14 π electrons, which perfectly fill bonding molecular orbitals, enhancing stability. The polygon and circle method, or Frost circle, visualizes this by drawing polygons with one corner down, indicating molecular orbitals. Electrons fill from the lowest energy level according to the Aufbau principle and Hund's rule. Compounds with unfilled orbitals, like antiaromatic ones, exhibit instability. Understanding these principles clarifies why certain molecular structures, such as benzene, are particularly stable due to their electron configurations.

Inscribed Polygon Method

Have you ever wondered why the Huckel's rule number of pi electrons, 2, 6, 10, 14, make molecules so stable? Okay. Maybe you weren't wondering that, maybe it wasn't keeping you up at night, but in this video, I'm going to finally explain why these numbers are so important, why they are so special, and we're going to learn a visualization technique that will help us to understand molecular orbital theory better when it comes to aromatic molecules. Okay? So this is called the inscribed polygon method. It also goes by a lot of other names. It's also called the polygon and circle method or a Frost Circle, okay? If you see any of those terms, it's all referring to the same exact thing. It's a method that helps us to visualize the identities of pi electrons, okay? And this is going to explain to us. It's going to be a visual representation of why our Huckel's rule numbers are so special, why they are so important. Okay? So what we are going to do is we are going to do a worked example of 3 different molecules and then we'll go ahead and, you know, do some practice problems. Okay? So here we have 3 different molecules. It says use the polygon and circle method to predict the stability of the following molecules. I'm going to go ahead and add some stuff here. So for example, with this nitrogen, let's go ahead and make this a hydrogen. Okay? So let's go ahead and make it a nitrogen with one lone pair and a hydrogen. The cyclobutadiene, we are keeping just as is. The triangle, it's got a longer name that I don't want to complicate you with. But, the 3-membered ring has a negative charge. That's fine. So, what we're going to do is we are just going to do kind of steps and I am going to do all the steps with all 3 molecules so you can see how they work. The very first step of this method, whether you call it a Frost Circle or polygon and circle, your first method is your first step is always going to be to draw the polygon, whatever shape it is, with one corner facing down. So as you can see, I've already done that step. I've already drawn it every single shape with one of these corners facing down. Now, if you were given the shape like this, then it would simply be your responsibility to redraw it in a way that one corner is at the bottom. Think of it like it's standing on its very tip. Okay? Now, what we're going to do in the next step is you have to draw molecular orbitals on all corners of the ring. Guys, that sounds a lot harder than it is. It just means draw a line next to every atom of the ring. Okay? So, a 5-member ring gets 5 molecular orbitals. 4-member ring gets 4. 3-member ring gets 3. It's that easy. Okay? Now, we're going to draw a line that splits the polygon down the middle in a basically, you know, a horizontal line that's going to split it into 2 different halves. Okay? So, now for the square, that happens to be easy because a halfway line would just go right through the middle of both of those corners. Now, for a triangle, that's also easy because you're just going to put the dotted line somewhere in the middle, but now for a 5-membered ring or sometimes bigger rings, sometimes it can get confusing where to put the line. Obviously, when it's drawn like this, the line is going to go above 3 molecular orbitals and below 2 molecular orbitals. But some students because they're really bad at drawing, they just suck at drawing. I don't blame you. I used to be one of you. I had to get good because this is like my job now. Some students make the mistake of doing this. They like go below that molecular orbital. And you'd be surprised if you draw, let's say you draw your 5-member ring like this, No, like this. Okay? No, I'm messing up. But there's a way to draw it. There you go. So let's say you draw your 5-number ring like this. Well then when you draw the halfway point, you're going to think that it's actually below 4 orbitals and above only 1. But that's not the way it should be. You should If you're splitting with an uneven number of orbitals, you should make it so that they're as close together as possible. So instead of being 4 orbitals on top and 1 orbital on the bottom, it should be 2 on the top and 3 on the bottom like we have here. So hopefully, that kind of helps. If you ever see a completely unequal number of orbitals, that means you probably drew it wrong. You should have a relatively even number of orbitals on both sides. Okay? Excellent. So, we drew the line. Now you're going to insert the number of pi electrons that you have into your orbitals starting from your lowest energy orbital and working your way up, guys. This is called the Aufbau principle, right? Aufbau principle was the building up principle, just means that you have to always fill your lowest energy orbitals first. And we're saying that energy goes up. So energy increases the higher you go. So, let's just look at the 1st molecule. The 1st molecule is one that we already learned how to solve. It's a heterocycle. How many pi electrons does that molecule have? You have to think, will the nitrogen donate? Does it want to donate its lone pair? Yes, it does. Okay? Because we've got 2, 4 electrons, 4 pi electrons, that lone pair is going to make it 6. It's sp₃, so that works out. So that means I have 6 electrons to add. How do we add them? 1₂ here. Then my 3rd electron goes here. My 4th electron goes here. Okay. That's a whole other rule. Okay. That was actually Hund's rule. Hund's rule says that you can't, if you have orbitals of the same energy level, you have to fill them evenly. Okay? So, your 3rd and your 4th electrons go 1 a piece. But we have 6 total. So then my 5th goes here and my 6th goes there. That's it. So I'm done with that step. Let's move on to cyclobutadiene. How many pi electrons do I have for that one? Four. Okay. So it's going to be 1, 2, 3, 4 according to Aufbau principle and then according to Hund's rule I have those 2 even, energy orbitals, so I have to fill them evenly. Okay? Now, just so you guys know, there's a more technical term for orbitals that have the same energy level. Do you guys remember that name? That term, it's actually from chapter 1 of organic chemistry. They're called degenerate orbitals. Okay? So if you have degenerate orbitals, that means that you have to fill them evenly. Okay? That's it. You can't just put 2 on one side and 0 on the other. That doesn't make a lot of sense. Finally, what do we get for the triangle? Okay. The cyclopropenyl anion. So what we would get is 1, 2, 3, 4, right? Because again we've got 4 pi electrons and so we've got to have that even distribution at the top. Perfect. So what did we just do? You might be wondering, okay, Johnny, we drew all these arrows, but where is this going? Well, it turns out that we can use this diagram to understand why molecules are more stable or less stable, and we can understand the identities of the electrons and the identities of the orbitals. Because it turns out that that halfway point actually represents what we call the nonbonding line in the molecular orbital theory, where everything below that line represents a bonding molecular orbital. Okay? So bonding molecular orbitals are the first ones to get filled. They're the ones that contribute to bonding. They're the ones that contribute to things wanting to stay together. And then our antibonding orbitals are the ones that get filled up after all the bonding ones are full. Okay? You would never put something in the top orbital until all of your bottom ones are full. Okay? Well, it turns out that when you have filled molecular orbitals, remember molecular orbital would just be one of these. Right? If all of your molecular orbitals are filled, that's going to contribute to unique stability because remember that we learned a long time ago from chapter 1 of organic chemistry and from gen chem gen chem that orbitals love to be have 2 electrons. If an or if an orbital has 2 electrons, it's 2 electrons, it's happy. Okay? Now, what if all of the bonding orbitals have 2 electrons? That's going to make it uniquely stable because that means that basically all of your bonding orbitals are perfectly filled. Okay? Now, what if you have partially filled molecular orbitals? What if you have a weird number of electrons and you have some molecular orbitals that are hanging out with 1 electron apiece, that's going to contribute to unique instability, guys. That's going to make it unstable because now you have these unfilled orbitals that are trying to get filled with something. No orbital likes to only carry 1 electron. They always want to carry 2. Okay? So what does this mean back to our diagrams? Well, look what's going on. Normally, we would predict that what would be the aromatic of these molecules. Well, the first one would be aromatic. The second one is supposed to be antiaromatic. The last one is also supposed to be antiaromatic. Now are you seeing a pattern here? Notice, look what's going on. The aromatic molecule, the one with 6 pi electrons, Huckel's rule number happens to have all of its bonding orbitals perfectly filled, right? That's going to make it stable. That's going to make it really stable, right? Whereas the ones that are antiaromatic, the ones that have a non-Huckel's rule number or what we call Breslow's rule number, notice that they have this. What is that? They have these partially filled orbitals. Partially filled. Do you think that's going to make it stable? That's going to make it uniquely unstable. Look at these numbers. The electrons here are 4 electrons, the electrons over there for the first one is 6. So guys, the reason that the Huckel's rule numbers of 2, 6, 10, 14 are so stable is because those are the combinations of electrons that are always going to be required to perfectly fill your bonding orbitals. Any number off of that, an odd number. Right? Or instead of an odd number, a 4n number. These are going to kick electrons up to the antibonding orbitals and make unfilled partially filled orbitals that are going to contribute to instability. Okay? So hopefully, this helps you guys get a better grip on, wow, this wasn't just like a memory game. This actually made sense. These numbers are important. There's a reason that benzene is so stable and it's because this example here is benzene. It's because benzene has 6 pi electrons so all of its bonding orbitals are perfectly filled. Alright? So, you guys are going to do some practice so you guys can get a better idea of this, but hopefully that makes sense. Let me know if you have any questions. Let's move on to the next topic.

Inscribed Polygon Method

Hey guys, let's take a look at the following question. So here, apply the polygon circle method, which we also call Frost Circles, to the following compound. Does it show any special stability? If yes, why? So basically, we have to determine if this molecule is aromatic, non-aromatic, or anti-aromatic. Aromatic shows very high stability. Non-aromatic just means your normal compound. Anti-aromatic means you're super unstable. So if we take a look at the name, we have tropylium cation. Now, another name for tropylium just means that we have cycloheptatriene. The tropylium just means cycloheptatriene. Cyclo means ring. Hepta means 7 carbons. So, we have 7 carbons and triene means 3 double bonds. Now, I say the word cation, which means this carbon up here which is not double bonded like the others is positive. That will be our molecule. Now, here we're going to draw the frost circle. Now, we're going to draw an apex down the molecule, so the point part down. The larger these rings get, the harder it becomes to draw them. It takes practice, guys, to make sure you draw it correctly. So not the straightest but good enough. Now, we're going to cut this in half. So here, this is your nonbonding region. Down here is your bonding. Up here is your antibonding. Everywhere a carbon touches becomes a molecular orbital. Now, we're going to say how many pi electrons do we have? We have 2, 4, 6 pi electrons. So 1 up, 1 down. Up, up, down, down. We're going to say this molecule shows special stability because all the molecular orbitals in the bonding region are completely filled in, which would indicate that we have an aromatic compound. So tropylium cation is aromatic. Here we know it from the old rules that we talked about, but we also know it because we just did a frost circle and it proves it. The molecular orbitals in the bonding region are all completely filled in.

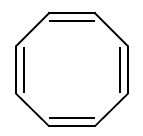
Draw the inscribed polygon for the following molecule.

Hey everyone. Let's take a look at the following example question. Here it says, first circle the 2 monosaccharides that will produce identical aldauric acids upon treatment with nitric acid. Then, label the products of these oxidations as optically active, meaning chiral, or optically inactive, meaning achiral. Alright. So let's do the first part. We know that treatment of these monosaccharides with nitric acid is going to oxidize not only their aldehydes but also the primary alcohols on the bottom. So these aldehydes, we're just going to say they're all going to become OH groups. So let's work on showing that. So all of these will become OH groups, and then all of these down here will become carboxylic acids. So we'll have a carboxylic acid, carboxylic acid, same here, same here. And then down here, we'll have our carboxylic acid. So their resutls are products or diacids that are created from oxidation with nitric acid. We have to look to see which ones would be identical. Here, it's not as easy to see both. So we had all of these monosaccharides. And if we take a look, we have L-gulose, which would undergo this oxidation to create this structure. And we have D-glucose, which is our first structure, it undergoes oxidation as well. It may not look like it, but they're actually identical. So let's just imagine that we took this and we flipped it. So the top part comes down here and this bottom part gets rotated up here. Doing that gives us what type of image? Well, if we flip it that way, let's draw what we would get. We get this carboxylic acid up here and then let's draw the rest of it. And then we get down here. So I'm flipping it this way. Alright. So when I flip it this way, these OH's now, they're going to be over here because I'm flipping it that way. Alright. And this H would be here and this OH would be down here. And flipping it that way, we would say that these hydrogens after we've flipped it, they would be up here, and then this OH would be down here, and this H would be here. If you look at that, what does that resemble? This structure here resembles this structure right here. So upon strong oxidation, we would say that the products, the oxidized products of the glucose in L-gulose, will be identical. So that's the first part of this question. We couldn't do that with either of the monosaccharides in the middle in the form of L-galactose or D-mannose. Right. So we're gonna say here for this first part, it's the D-glucose plus the L-gulose. Now, looking at our carboxylic acids that we've created, our dark carboxylic acids, let's answer this next part. It says, then label the products of these oxidations as optically active, meaning chiral or optically inactive. Now, to be optically inactive, where are the scenarios that help to create something as being optically inactive and therefore achiral? Well, first, they'd have to have either no chiral centers or they'd have to be a meso compound. The first one is out of the question because all of these have chiral centers. All 4 of these monosaccharides even in their oxidized forms would still have chiral centers. So we're not looking at that. What we're looking for is which one represents a meso compound. Remember, a meso compound has an internal plane of symmetry. The way we can check this is just cut these diacids down the middle and look, do we have some type of internal plane of symmetry? Does the top portion match the bottom portion? If we take a look, let's look at this top portion here. This top portion, doesn't look like the bottom portion. So let's make this one 1 and this one 1, and this 2 and this is 2, and this is 3, and this is 3. Okay. So the ones are the same, but that's not a reference to a chiral center. 2 and 2. Alright. So we would say that yes, both of them have an OH on the right so they are mirroring each other. But then, if we look at these as threes, they don't match up. It's not looking in a mirror. This OH is here, so there'd have to be an OH here. So there is no internal plane of symmetry and therefore, this is not a meso compound, meaning that it will be chiral overall and optically active. Let's go to the next one. Here's our mirror here. Let's look in the mirror. So we have 1,1,2,2,3,3. Looking in this mirror, there's an OH here And for 2, there's an OH here. Okay? For 3, there's an OH here and there's an OH here. So this thing is looking at itself in a mirror internally and it's seeing itself back, its own reflection. So there is an internal bit of symmetry within this, oxidized form of L-galactose. So in its diacid oxidized form, we would say that it's optically inactive because it is a meso compound. For the next one, 1, 1, 2, 2, 3, 3. Looking into the mirror, for 2, there's an OH on the right, but for this 2, it's on the left. So it doesn't match up automatically. It can't be a meso compound, so it's optically active. And then finally, this last one, 1, 1, 2, 2, 3, 3. Looking in the mirror for carbon 2, it sees an OH on the left. There's an OH on the left. Okay. For carbon 3, this one has its OH on the right. Oh, but this one does not. So there is no internal plane of symmetry where the top part matches perfectly with the bottom part, so this could not be a meso compound and therefore it's optically active. So going back to the beginning of this question, we would say that the 2 monosaccharides that form identical aldauric acids upon oxidation with nitric acid would be D-glucose and L-gulose. And we would say that all of these diacids, their oxidized forms, are optically active except for the diacid form of L-galactose. If we cut it right in the middle, we would say that the top part and the bottom part match. There's an internal plane of symmetry at play making this a meso compound and therefore optically inactive. Right? So just keep this in mind when you are faced with questions like this. Take a similar approach to determine if things are identical and whether they are chiral or achiral in nature. Doing this and applying these concepts will help you with questions further on.

Here’s what students ask on this topic:

What is the Frost Circle method in organic chemistry?

The Frost Circle method, also known as the inscribed polygon method or polygon and circle method, is a visualization technique used in organic chemistry to understand the stability of aromatic compounds. It involves drawing a polygon with one corner facing down inside a circle, where each vertex represents a molecular orbital. By filling these orbitals with π electrons according to the Aufbau principle and Hund's rule, one can predict the stability of the molecule. This method helps explain why certain numbers of π electrons (2, 6, 10, 14) make molecules particularly stable, as these numbers perfectly fill the bonding molecular orbitals.

How does the Frost Circle method explain Hückel's rule?

The Frost Circle method explains Hückel's rule by visually representing the molecular orbitals of a cyclic compound. When a polygon is inscribed in a circle with one vertex pointing down, each vertex corresponds to a molecular orbital. According to Hückel's rule, aromatic stability is achieved when the number of π electrons is 4n+2 (where n is a non-negative integer). These numbers (2, 6, 10, 14) perfectly fill the bonding molecular orbitals, leading to enhanced stability. The Frost Circle method shows that any deviation from these numbers results in partially filled or anti-bonding orbitals, causing instability.

Why are 2, 6, 10, and 14 π electrons considered special in Hückel's rule?

In Hückel's rule, 2, 6, 10, and 14 π electrons are considered special because they perfectly fill the bonding molecular orbitals of a cyclic compound, leading to maximum stability. These numbers follow the 4n+2 formula, where n is a non-negative integer. When these bonding orbitals are fully occupied, the molecule achieves a stable electronic configuration. The Frost Circle method visually demonstrates this by showing that these specific numbers of π electrons result in all bonding orbitals being filled, while any other number leads to partially filled or anti-bonding orbitals, causing instability.

What is the Aufbau principle and how is it applied in the Frost Circle method?

The Aufbau principle, also known as the building-up principle, states that electrons fill the lowest energy orbitals first before occupying higher energy levels. In the Frost Circle method, this principle is applied by filling the molecular orbitals of a cyclic compound starting from the lowest energy level. When drawing the polygon with one corner down, each vertex represents a molecular orbital. Electrons are added to these orbitals beginning from the lowest energy level, ensuring that the bonding orbitals are filled first. This helps predict the stability of the molecule based on its electron configuration.

How does the Frost Circle method help in predicting the stability of aromatic compounds?

The Frost Circle method helps predict the stability of aromatic compounds by visually representing their molecular orbitals and electron configurations. By drawing a polygon with one corner facing down inside a circle, each vertex corresponds to a molecular orbital. Electrons are then added according to the Aufbau principle and Hund's rule. If the number of π electrons perfectly fills the bonding orbitals (following Hückel's rule), the compound is stable. If there are partially filled or anti-bonding orbitals, the compound is unstable. This method provides a clear visual explanation for the stability of aromatic compounds.