So we know that significant figures will play a role in the way we analyze data and analyze questions. We also know it's going to have an impact on the answers that we have at the end. Now there are levels of precision that are involved when it comes to significant figures. We're going to say here the more significant figures in a measurement, then the more precise it is. So, for example, a reading of 25.00 ml is more precise than just 25. That's because 25.00 ml has a decimal point. We move from left to right. Our first non-zero number here is 2, and count all the way to the end. This has 4 sig figs. 25, on the other hand, has no decimal point, so we move from right to left, and it only has 2 sig figs. Since 25.00 ml has more significant figures, it's more precise. It gives us more detail. Now, when it comes to recording measurements, significant figures have to be taken into account. So we're going to say when taking a measurement, you must include all of the known numbers plus an additional decimal place. Now we use what I call the eyeball test. It's based on an estimate or best guess from looking. So even when looking at a measuring tape or looking at a beaker, significant figures have to be taken into account. We can't just look at the measurements or the hash marks on these tools and say, that is my value, because there's some level of uncertainty there. So you have to add an additional decimal place to ensure you have the correct number of significant figures. Now that we've talked about this idea of precision in measurements, click on the next video, and let's put it to practice with an example question.
- 1. The Chemical World9m
- 2. Measurement and Problem Solving2h 25m
- 3. Matter and Energy2h 15m
- Classification of Matter18m
- States of Matter8m
- Physical & Chemical Changes19m
- Chemical Properties8m
- Physical Properties5m
- Temperature (Simplified)9m
- Law of Conservation of Mass5m
- Nature of Energy5m
- First Law of Thermodynamics7m
- Endothermic & Exothermic Reactions7m
- Heat Capacity17m
- Thermal Equilibrium (Simplified)8m
- Intensive vs. Extensive Properties13m
- 4. Atoms and Elements2h 33m
- The Atom (Simplified)9m
- Subatomic Particles (Simplified)12m
- Isotopes17m
- Ions (Simplified)22m
- Atomic Mass (Simplified)17m
- Periodic Table: Element Symbols6m
- Periodic Table: Classifications11m
- Periodic Table: Group Names8m
- Periodic Table: Representative Elements & Transition Metals7m
- Periodic Table: Phases (Simplified)8m
- Periodic Table: Main Group Element Charges12m
- Atomic Theory9m
- Rutherford Gold Foil Experiment9m
- 5. Molecules and Compounds1h 50m
- Law of Definite Proportions9m
- Periodic Table: Elemental Forms (Simplified)6m
- Naming Monoatomic Cations6m
- Naming Monoatomic Anions5m
- Polyatomic Ions25m
- Naming Ionic Compounds11m
- Writing Formula Units of Ionic Compounds7m
- Naming Acids18m
- Naming Binary Molecular Compounds6m
- Molecular Models4m
- Calculating Molar Mass9m
- 6. Chemical Composition1h 23m
- 7. Chemical Reactions1h 43m
- 8. Quantities in Chemical Reactions1h 16m
- 9. Electrons in Atoms and the Periodic Table2h 32m
- Wavelength and Frequency (Simplified)5m
- Electromagnetic Spectrum (Simplified)11m
- Bohr Model (Simplified)9m
- Emission Spectrum (Simplified)3m
- Electronic Structure4m
- Electronic Structure: Shells5m
- Electronic Structure: Subshells4m
- Electronic Structure: Orbitals11m
- Electronic Structure: Electron Spin3m
- Electronic Structure: Number of Electrons4m
- The Electron Configuration (Simplified)20m
- The Electron Configuration: Condensed4m
- Ions and the Octet Rule9m
- Valence Electrons of Elements (Simplified)5m
- Periodic Trend: Metallic Character4m
- Periodic Trend: Atomic Radius (Simplified)7m
- Periodic Trend: Ionization Energy (Simplified)9m
- Periodic Trend: Electron Affinity (Simplified)7m
- Electron Arrangements5m
- The Electron Configuration: Exceptions (Simplified)12m
- 10. Chemical Bonding2h 10m
- Lewis Dot Symbols (Simplified)7m
- Ionic Bonding6m
- Covalent Bonds6m
- Lewis Dot Structures: Neutral Compounds (Simplified)8m
- Bonding Preferences6m
- Multiple Bonds4m
- Lewis Dot Structures: Multiple Bonds10m
- Lewis Dot Structures: Ions (Simplified)8m
- Lewis Dot Structures: Exceptions (Simplified)12m
- Resonance Structures (Simplified)5m
- Valence Shell Electron Pair Repulsion Theory (Simplified)4m
- Electron Geometry (Simplified)7m
- Molecular Geometry (Simplified)9m
- Bond Angles (Simplified)11m
- Dipole Moment (Simplified)14m
- Molecular Polarity (Simplified)7m
- 11 Gases2h 12m
- 12. Liquids, Solids, and Intermolecular Forces1h 11m
- 13. Solutions3h 1m
- 14. Acids and Bases2h 14m
- 15. Chemical Equilibrium1h 27m
- 16. Oxidation and Reduction1h 33m
- 17. Radioactivity and Nuclear Chemistry53m
Significant Figures: Precision in Measurements: Study with Video Lessons, Practice Problems & Examples
 Created using AI
Created using AISignificant Figures are used to discuss the level of precision in any measurement.
Recording Measurements
Significant Figures Precision Concept
Video transcript
Significant Figures Precision Example
Video transcript
Alright everyone, so here it says in this example question, "Determine the number of significant figures involved in measuring the length of the square." So in measuring the length of the square we have this measurement. We see that this here is the 3.0 centimeter hash mark, and this is 3.1 and this is 3.2, so we see 3.2 centimeters. Remember, with the right number of significant figures we have to add an additional decimal place. That would mean that the best answer would be 3.20 centimeters, making option C our best choice.
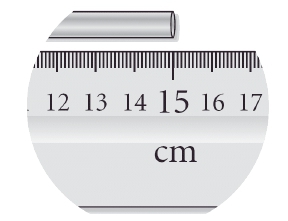
Read the length of the metal bar to the correct number of significant figures.
What is the correct reading for the liquid in the burette provided below?
Here’s what students ask on this topic:
What are significant figures and why are they important in scientific measurements?
Significant figures are the digits in a measurement that carry meaning contributing to its precision. They include all known digits plus one estimated digit. Significant figures are crucial because they indicate the precision of a measurement and help ensure that calculations based on these measurements are accurate. For example, a measurement of 25.00 mL (4 sig figs) is more precise than 25 mL (2 sig figs). This precision is essential in scientific experiments to ensure reliable and reproducible results, minimizing errors and uncertainties in data analysis.
 Created using AI
Created using AIHow do you determine the number of significant figures in a given measurement?
To determine the number of significant figures in a measurement, follow these rules: 1) All non-zero digits are significant. 2) Any zeros between significant digits are also significant. 3) Leading zeros (zeros before the first non-zero digit) are not significant. 4) Trailing zeros in a decimal number are significant. For example, in 25.00 mL, all four digits are significant, giving it 4 sig figs. In contrast, 0.025 has only two significant figures (2 and 5), as the leading zeros are not counted.
 Created using AI
Created using AIWhy is 25.00 mL considered more precise than 25 mL?
25.00 mL is considered more precise than 25 mL because it has more significant figures. 25.00 mL has four significant figures, indicating a higher level of detail and precision in the measurement. In contrast, 25 mL has only two significant figures. The additional digits in 25.00 mL reflect a more accurate measurement, reducing uncertainty and providing more reliable data for scientific analysis.
 Created using AI
Created using AIHow do you record measurements to ensure the correct number of significant figures?
To record measurements with the correct number of significant figures, include all known digits from the measuring instrument plus one estimated digit. This estimated digit accounts for the uncertainty in the measurement. For example, if a beaker shows a measurement between 25 and 26 mL, you might record it as 25.3 mL, where 25 is known, and 0.3 is estimated. This practice ensures that the measurement reflects the precision of the instrument and provides accurate data for analysis.
 Created using AI
Created using AIWhat is the 'eyeball test' in the context of significant figures?
The 'eyeball test' refers to the practice of estimating the last digit in a measurement based on visual inspection. When using measuring tools like a beaker or ruler, you record all known digits and add one estimated digit to account for uncertainty. For example, if a liquid level is between 25 and 26 mL, you might estimate it as 25.3 mL. This estimated digit ensures that the measurement includes the correct number of significant figures, reflecting the precision of the measurement.
 Created using AI
Created using AIYour Introduction to Chemistry tutor
- Use the following graph for problems1.23 and 1.24: d. How many minutes were needed to reach a temperature of...
- a. What is the specific gravity of the following solution?
- Assume that you are delivering a solution sample from a pipette. Figures (a) and (b) show the volume level bef...
- Measure the length of each of the objects in diagrams (a), (b), and (c) using the metric ruler in the figure. ...
- State the temperature on the Celsius thermometer to the correct number of significant figures: (2.3) A