Oftentimes, when dealing with calculations, we'll run into contact with the metric prefixes. Now, metric prefixes can be seen as modifiers that are multiples of 10. And we're going to say when dealing with these metric prefixes, we're going to have this chart here. This metric prefix multipliers chart ranges from 10 to the 12 to 10 to the negative 12. Now talk to your professor. Make sure you don't have to know anything beyond this point. For this level of chemistry, this is a pretty thorough range to deal with. But there, of course, there are terms 10 to the negative 15, 10 to the positive 15. Well, those are usually reserved for higher levels of chemistry. Now 10 to the 12 is called terra. Terra uses the variable of t, capital T. 10 to the 9 is giga, which is capital G. Mega is capital M, and it's 10 to the 6. 10 to the 3 is kilo. Now, at this point, we're gonna be dealing with lowercase letters, so lowercase k. You might have heard of kilometer. So kilometer has kilo in it. It's metric prefix label. 10 to the 2 is hecto, which is H. Now 10 to the 1 is related to deka, which is DA. Here is when we're dealing with our base unit, So our base unit like liters or seconds. We're gonna say here this is not a metric prefix, this is just the base, our base unit. Then we have deci. Dep and deci are pretty similar. To differentiate them, deci is D. Then we're gonna have centi, which is 10 to the negative 2. So that's gonna be C. You might have heard of centimeters. Then we have 10 to the negative 3 which is milli, milliliters. Then 10 to the negative 6 is micro. Micro is an interesting symbol, looks like μ. 10 to the negative 9 is nano, which is lowercase n, and then 10 to the negative 12 is pico, which is p. Now this is a lot of terms. This is a lot of symbols, but we have our first memory tool. So when we have memory tools, either just simple phrases sometimes or images that'll help us memorize a specific chemistry-related topic. Here in this case, this memory tool help us memorize the order for the metric prefix multipliers. Now we have King Henry from history, King Henry who kept on divorcing his wives until he they wanted them to compare him a son. And with King Henry, we have a trusty, memory tool to help us memorize the order of the metric prefixes. So we're gonna say the great monarch, King Henry's daughter Barbara, drinks chocolate milk until 9 pm. So we can see here that each one of these highlighted letters, each one of these highlighted letters here, corresponds to these metric prefix multipliers. And this memory tool also ranges from 10 to the 12, and it decreases all the way down to 10 to the negative 12 over here. So just remember, your metric prefixes for us, we're gonna range from 10 to 12 to 10 to the negative 12. Consult with your professor to make sure that that's the range all you that that you need to know in terms of this topic. And in terms of memorizing, rely on this memory tool. It'll help you remember the order that each metric prefix multiplier comes into play when it comes to this chart. Now that we've talked about the general idea of metric prefixes and this range, move on to the next video, and let's see how we can apply them to base units.
- 1. The Chemical World9m
- 2. Measurement and Problem Solving2h 25m
- 3. Matter and Energy2h 15m
- Classification of Matter18m
- States of Matter8m
- Physical & Chemical Changes19m
- Chemical Properties8m
- Physical Properties5m
- Temperature (Simplified)9m
- Law of Conservation of Mass5m
- Nature of Energy5m
- First Law of Thermodynamics7m
- Endothermic & Exothermic Reactions7m
- Heat Capacity17m
- Thermal Equilibrium (Simplified)8m
- Intensive vs. Extensive Properties13m
- 4. Atoms and Elements2h 33m
- The Atom (Simplified)9m
- Subatomic Particles (Simplified)12m
- Isotopes17m
- Ions (Simplified)22m
- Atomic Mass (Simplified)17m
- Periodic Table: Element Symbols6m
- Periodic Table: Classifications11m
- Periodic Table: Group Names8m
- Periodic Table: Representative Elements & Transition Metals7m
- Periodic Table: Phases (Simplified)8m
- Periodic Table: Main Group Element Charges12m
- Atomic Theory9m
- Rutherford Gold Foil Experiment9m
- 5. Molecules and Compounds1h 50m
- Law of Definite Proportions9m
- Periodic Table: Elemental Forms (Simplified)6m
- Naming Monoatomic Cations6m
- Naming Monoatomic Anions5m
- Polyatomic Ions25m
- Naming Ionic Compounds11m
- Writing Formula Units of Ionic Compounds7m
- Naming Acids18m
- Naming Binary Molecular Compounds6m
- Molecular Models4m
- Calculating Molar Mass9m
- 6. Chemical Composition1h 23m
- 7. Chemical Reactions1h 43m
- 8. Quantities in Chemical Reactions1h 16m
- 9. Electrons in Atoms and the Periodic Table2h 32m
- Wavelength and Frequency (Simplified)5m
- Electromagnetic Spectrum (Simplified)11m
- Bohr Model (Simplified)9m
- Emission Spectrum (Simplified)3m
- Electronic Structure4m
- Electronic Structure: Shells5m
- Electronic Structure: Subshells4m
- Electronic Structure: Orbitals11m
- Electronic Structure: Electron Spin3m
- Electronic Structure: Number of Electrons4m
- The Electron Configuration (Simplified)20m
- The Electron Configuration: Condensed4m
- Ions and the Octet Rule9m
- Valence Electrons of Elements (Simplified)5m
- Periodic Trend: Metallic Character4m
- Periodic Trend: Atomic Radius (Simplified)7m
- Periodic Trend: Ionization Energy (Simplified)9m
- Periodic Trend: Electron Affinity (Simplified)7m
- Electron Arrangements5m
- The Electron Configuration: Exceptions (Simplified)12m
- 10. Chemical Bonding2h 10m
- Lewis Dot Symbols (Simplified)7m
- Ionic Bonding6m
- Covalent Bonds6m
- Lewis Dot Structures: Neutral Compounds (Simplified)8m
- Bonding Preferences6m
- Multiple Bonds4m
- Lewis Dot Structures: Multiple Bonds10m
- Lewis Dot Structures: Ions (Simplified)8m
- Lewis Dot Structures: Exceptions (Simplified)12m
- Resonance Structures (Simplified)5m
- Valence Shell Electron Pair Repulsion Theory (Simplified)4m
- Electron Geometry (Simplified)7m
- Molecular Geometry (Simplified)9m
- Bond Angles (Simplified)11m
- Dipole Moment (Simplified)14m
- Molecular Polarity (Simplified)7m
- 11 Gases2h 12m
- 12. Liquids, Solids, and Intermolecular Forces1h 11m
- 13. Solutions3h 1m
- 14. Acids and Bases2h 14m
- 15. Chemical Equilibrium1h 27m
- 16. Oxidation and Reduction1h 33m
- 17. Radioactivity and Nuclear Chemistry53m
Metric Prefixes: Study with Video Lessons, Practice Problems & Examples
 Created using AI
Created using AIUnderstanding metric prefixes is essential for scientific calculations, as they represent multiples of ten. Key prefixes include tera (1012), giga (109), mega (106), kilo (103), and milli (10-3). These prefixes can be applied to base units like liters, seconds, and amperes, facilitating conversions such as milliliters and nanoseconds. A mnemonic, "King Henry's Daughter Barbara Drinks Chocolate Milk Until 9 PM," aids in memorizing the order of these prefixes, enhancing comprehension and application in chemistry.
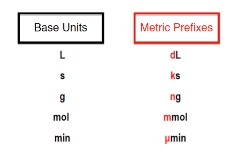
Metric Prefixes are “labels” that can be placed in front of base units.
Metric Prefix Multipliers
Metric Prefixes
Video transcript
Metric Prefixes
Video transcript
The metric prefixes themselves can act as labels that can be placed in front of various base units. So here we have base units for volume in the form of liters, in terms of time we use seconds, the base units for the amount of a substance are moles, and for electrical current, amperes. Notice that some of these common base units are also connected to our SI base units. Now, the metric prefixes themselves are the variables that we place in front of each of these base units. So for liters, we could plug in front of it milliliters, milli being the metric prefix that we're placing in front of the base unit of liters. For seconds, we could do nanoseconds. For moles, we could do gigamoles, and for amperes, we could do kiloamperes. Here, all that's happening is I'm taking each one of these metric prefixes and placing them in front of one of these base units. This is key when it comes to metric prefix conversions. So click on the next video, and let's take a look at how do we go from one metric prefix to a new one.
Metric Prefixes Example 1
Video transcript
So here if we take a look at this example, it says, convert the following value to desired units. We need to convert 694 kilograms to micrograms. Alright. Step 1, if the given value has a metric prefix, which it does because kilo is our metric prefix, then convert it to the base unit. The base unit is just a unit after you've removed the metric prefix. So the base unit here would just be grams. Alright. We're going to have 694 kilo is our metric prefix, grams. We're going to say in order to cancel out units, always make sure that they are on opposite levels. We want to cancel out these kilograms which are on the top, so we're going to place kilograms here on the bottom. And then we need to change this into our base units of grams. Now the next part is important. Always place the coefficient of 1 on the side with the metric prefix. Our metric prefix here is kilo, so we're going to say here 1 kilo, and remember that comes from what we saw up above. Based on the metric prefix multipliers chart, we saw that when it is kilo, it's 10 to the 3, so it'd be 103. Now because the kilograms are on opposite levels, they can cancel out. Next, we're going to say, if necessary, convert the metric the base unit to a new metric prefix. Now we didn't ask to find our answer in grams. We're asked to find it in micrograms. So we must continue onward. Grams are here on the top. In order to cancel them out, I put them here on the bottom, and then we need to get to micrograms, micro is our metric prefix, and then micrograms. Again, the coefficient of 1 is associated with the metric prefix, it's on the same side with it. So 1 micro is, according to the metric prefix multipliers chart above, it's 10-6. So now grams cancel out and we'll have at the end our value. So here that would be 694 times 103 divided by 10-6. Now, some of you, depending on your calculator, if you plug it in as you see it, you might get the wrong answer. So anytime you have 10 to a power, it's always best to put it in parentheses in your calculator. Otherwise, you may get the incorrect answer. So it'd be 694 times, in parentheses, 103, divided by, in parentheses, 10-6. If you do that correctly, you should get back in your calculator 6.941011 micrograms as your answer. And this represents our metric prefix conversions. We're just going from a metric prefix to either the base unit or a new metric prefix. Take to heart the steps that we've outlined here to approach any problem like this.
Now that we've done this example question, move on to some practice questions.
Which quantity in the following pair is smaller?
Use the prefix multipliers to express each measurement without any exponents.
a) 32 x 10-13 L
b) 7.3 x 106 g
c) 18.5 x 1011 s
Problem Transcript
Use scientific notation to express each quantity with only the base unit.
a) 83 µm
b) 193 kg
c) 2.7 mmol
Problem Transcript
If a room has a volume of 1.15 x 108 cm3, what is the volume in km3?
Here’s what students ask on this topic:
What are the metric prefixes and their corresponding powers of ten?
Metric prefixes are modifiers that represent multiples of ten. Key prefixes include:
- Tera (T): 1012
- Giga (G): 109
- Mega (M): 106
- Kilo (k): 103
- Hecto (h): 102
- Deka (da): 101
- Base unit: 100
- Deci (d): 10-1
- Centi (c): 10-2
- Milli (m): 10-3
- Micro (μ): 10-6
- Nano (n): 10-9
- Pico (p): 10-12
These prefixes are used to simplify the expression of large or small quantities in scientific calculations.
 Created using AI
Created using AIHow can I memorize the order of metric prefixes?
A useful mnemonic to memorize the order of metric prefixes is: "King Henry's Daughter Barbara Drinks Chocolate Milk Until 9 PM." Each highlighted letter corresponds to a metric prefix:
- K: Kilo (103)
- H: Hecto (102)
- D: Deka (101)
- B: Base unit (100)
- D: Deci (10-1)
- C: Centi (10-2)
- M: Milli (10-3)
- U: Micro (10-6)
- N: Nano (10-9)
- P: Pico (10-12)
This mnemonic helps in remembering the sequence from larger to smaller prefixes.
 Created using AI
Created using AIHow do metric prefixes apply to base units?
Metric prefixes are placed in front of base units to indicate multiples or fractions of those units. For example:
- Milliliters (mL): Milli (10-3) + Liters (L)
- Nanoseconds (ns): Nano (10-9) + Seconds (s)
- Gigamoles (Gmol): Giga (109) + Moles (mol)
- Kiloamperes (kA): Kilo (103) + Amperes (A)
This system simplifies the expression and conversion of large and small quantities in scientific measurements.
 Created using AI
Created using AIWhat is the significance of metric prefixes in scientific calculations?
Metric prefixes are crucial in scientific calculations as they provide a standardized way to express large and small quantities. They simplify the notation and conversion of units, making it easier to perform calculations and communicate measurements. For example, instead of writing 0.000001 meters, you can write 1 micrometer (μm). This standardization is essential for accuracy and consistency in scientific research and education.
 Created using AI
Created using AICan you provide examples of metric prefix conversions?
Sure! Here are some examples of metric prefix conversions:
- 1 kilometer (km) = 1,000 meters (m)
- 1 milligram (mg) = 0.001 grams (g)
- 1 gigabyte (GB) = 1,000,000,000 bytes (B)
- 1 nanosecond (ns) = 0.000000001 seconds (s)
These conversions are based on the powers of ten associated with each metric prefix, making it straightforward to switch between different units.
 Created using AI
Created using AIYour Introduction to Chemistry tutor
- Write the abbreviation for each of the following units: c. kilometer
- Write the abbreviation for each of the following units: b. deciliter
- Write the abbreviation for each of the following units: a. milligram
- Write the complete name for each of the following units: a. cL
- Write the complete name for each of the following units: b. kg
- Write the complete name for each of the following units: c. ms
- Write the numerical value for each of the following prefixes: c. milli
- Write the numerical value for each of the following prefixes: b. tera
- Write the numerical value for each of the following prefixes: a. centi
- Use a prefix to write the name for each of the following: b. 10⁶ m
- Use a prefix to write the name for each of the following: c. 0.001 m
- Use a prefix to write the name for each of the following: d. 10⁻¹² m
- For each of the following pairs, which is the larger unit? c. m or km
- For each of the following pairs, which is the larger unit? b. milliliter or microliter
- For each of the following pairs, which is the larger unit? a. milligram or kilogram
- Give the full name of the following units: a. cc b. dm c. mm d. nL e. mg f. m³
- Use metric conversion factors to solve each of the following problems: d. A balloon has a volume of 3500 cm³....
- Use metric conversion factors to solve each of the following problems: c. A hummingbird has a mass of 0.0055 ...
- Use metric conversion factors to solve each of the following problems: b. A cooler has a volume of 5000 mL. W...
- Use metric conversion factors to solve each of the following problems: d. A jar contains 0.29 kg of olives. H...
- Use metric conversion factors to solve each of the following problems: c. A package of chocolate instant pudd...
- Use metric conversion factors to solve each of the following problems: a. The Daily Value (DV) for phosphorus...
- Use metric conversion factors to solve each of the following problems: b. A glass of orange juice contains 3....
