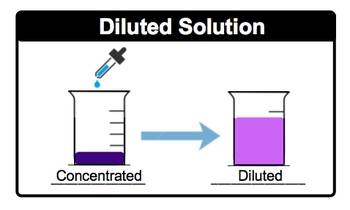
A standard, sometimes referred to as a stock solution, is a concentrated solution that will be diluted for some laboratory use later on. We're going to say dilution is just the addition of more solvent, usually water, to a solution in order to create a lower concentration. So, if we take a look here, we have our purple solvent here, which is actually a purple solution. It's pretty dark purple, meaning that it's concentrated. And what we're doing here is we're slowly adding more water to dilute it. As a result of this, it goes from being a dark purple to a lighter type of fuchsia or purple. That's showing us that it's not as concentrated as it was before. So here, this represents our diluted solution. Just remember, when we're talking about dilutions, we're just talking about adding water to our original solution to make it less concentrated.
- 1. The Chemical World9m
- 2. Measurement and Problem Solving2h 25m
- 3. Matter and Energy2h 15m
- Classification of Matter18m
- States of Matter8m
- Physical & Chemical Changes19m
- Chemical Properties8m
- Physical Properties5m
- Temperature (Simplified)9m
- Law of Conservation of Mass5m
- Nature of Energy5m
- First Law of Thermodynamics7m
- Endothermic & Exothermic Reactions7m
- Heat Capacity17m
- Thermal Equilibrium (Simplified)8m
- Intensive vs. Extensive Properties13m
- 4. Atoms and Elements2h 33m
- The Atom (Simplified)9m
- Subatomic Particles (Simplified)12m
- Isotopes17m
- Ions (Simplified)22m
- Atomic Mass (Simplified)17m
- Periodic Table: Element Symbols6m
- Periodic Table: Classifications11m
- Periodic Table: Group Names8m
- Periodic Table: Representative Elements & Transition Metals7m
- Periodic Table: Phases (Simplified)8m
- Periodic Table: Main Group Element Charges12m
- Atomic Theory9m
- Rutherford Gold Foil Experiment9m
- 5. Molecules and Compounds1h 50m
- Law of Definite Proportions9m
- Periodic Table: Elemental Forms (Simplified)6m
- Naming Monoatomic Cations6m
- Naming Monoatomic Anions5m
- Polyatomic Ions25m
- Naming Ionic Compounds11m
- Writing Formula Units of Ionic Compounds7m
- Naming Acids18m
- Naming Binary Molecular Compounds6m
- Molecular Models4m
- Calculating Molar Mass9m
- 6. Chemical Composition1h 23m
- 7. Chemical Reactions1h 43m
- 8. Quantities in Chemical Reactions1h 16m
- 9. Electrons in Atoms and the Periodic Table2h 32m
- Wavelength and Frequency (Simplified)5m
- Electromagnetic Spectrum (Simplified)11m
- Bohr Model (Simplified)9m
- Emission Spectrum (Simplified)3m
- Electronic Structure4m
- Electronic Structure: Shells5m
- Electronic Structure: Subshells4m
- Electronic Structure: Orbitals11m
- Electronic Structure: Electron Spin3m
- Electronic Structure: Number of Electrons4m
- The Electron Configuration (Simplified)20m
- The Electron Configuration: Condensed4m
- Ions and the Octet Rule9m
- Valence Electrons of Elements (Simplified)5m
- Periodic Trend: Metallic Character4m
- Periodic Trend: Atomic Radius (Simplified)7m
- Periodic Trend: Ionization Energy (Simplified)9m
- Periodic Trend: Electron Affinity (Simplified)7m
- Electron Arrangements5m
- The Electron Configuration: Exceptions (Simplified)12m
- 10. Chemical Bonding2h 10m
- Lewis Dot Symbols (Simplified)7m
- Ionic Bonding6m
- Covalent Bonds6m
- Lewis Dot Structures: Neutral Compounds (Simplified)8m
- Bonding Preferences6m
- Multiple Bonds4m
- Lewis Dot Structures: Multiple Bonds10m
- Lewis Dot Structures: Ions (Simplified)8m
- Lewis Dot Structures: Exceptions (Simplified)12m
- Resonance Structures (Simplified)5m
- Valence Shell Electron Pair Repulsion Theory (Simplified)4m
- Electron Geometry (Simplified)7m
- Molecular Geometry (Simplified)9m
- Bond Angles (Simplified)11m
- Dipole Moment (Simplified)14m
- Molecular Polarity (Simplified)7m
- 11 Gases2h 12m
- 12. Liquids, Solids, and Intermolecular Forces1h 11m
- 13. Solutions3h 1m
- 14. Acids and Bases2h 14m
- 15. Chemical Equilibrium1h 27m
- 16. Oxidation and Reduction1h 33m
- 17. Radioactivity and Nuclear Chemistry53m
Dilutions: Study with Video Lessons, Practice Problems & Examples
 Created using AI
Created using AIA stock solution is a concentrated solution that can be diluted by adding more solvent, typically water, to achieve a lower concentration. This process is represented by the equation 1
In Dilutions, a solvent (usually water) is added to a concentrated solution.
Concentrated & Diluted Solutions
Dilutions
Video transcript
Dilutions Example 1
Video transcript
In this example question, it says, if each sphere represents a mole of solute from the images provided below, arrange the solutions from least concentrated to most concentrated. Alright. So least concentrated is the same thing as saying the lowest molarity. Most concentrated means we have the highest molarity. Now remember, molarity itself represents moles of solute divided by liters of solution. So if we take a look here for a, a has in it 1, 2, 3, 4, 5 spheres. So that would be 5 moles of solute divided by 1 liter of solution, so that'd be 5 molar. For b, b is 1, 2, 3 spheres, so that's 3 moles of solute over 2 liters of solution, so that'd be 1.5 molar. And then finally, c, we have 1, 2, 3, 4, 5, 6 spheres, so 6 moles divided by 3 liters of solution, so that's 2 molar. So arranging it from lowest molarity to highest molarity, we're going to say the order would be b, then c, and then finally, a would have the highest molarity.
Dilutions
Video transcript
So at this point, we know that a dilution makes our solutions less concentrated. It takes us from a larger molarity value to a smaller molarity value. We're going to say dilution can be expressed by the following equation:
M1∙V1=M2∙V2Here, M1 and V1 represent the molarity and volume before dilution, while M2 and V2 are after the dilution. We are going to say here M1 is before a solvent is added. So M1, which is the more concentrated solution, is always larger than M2, which will be the diluted solution. Now V2 represents your final volume, and how exactly did we get to V2? Well, we started out with an initial volume and we added water to it. So:
V2=V1+the volume of solvent added
Dilutions Example 2
Video transcript
In this example question, it asks, "What volume in milliliters of 5.2 molar hydrobromic acid must be used to prepare 3.5 liters of 2.7 molar hydrobromic acid?" Now, how do we know this is a dilution question? Well, typically in a dilution question, we're only talking about one compound. And with that one compound, to be talking about dilution, we tend to deal with two molarities. So, the fact that we're dealing with just hydrobromic acid and we have two molarities associated with it is a strong indication that we're dealing with dilution. This means we're going to use M1V1=M2V2. Now, remember M1 is larger than M2 because M1 represents your concentrated solution before you've begun dilution.
Because of this, 5.2 molar has to be our M1. It's the larger molarity. Associated with M1 is V1. We don't see any number around it, so V1 is what we're looking for. Now remember, also that the word "of", when it's between two numbers, means multiply. We're going to say here that this is the smaller molarity, so this has to be M2, that's our diluted molarity. We're multiplying it with 3.5 liters, so based on the dilution equation, 3.5 liters has to be V2. We're going to isolate V1. So, divide both sides by 5.2 molar. The molarities cancel out and look, we'll have V1 but here it'll be in liters.
That comes out to be 1.8173 liters. We want the answer in milliliters so just do a quick metric prefix conversion. Liters on the bottom, milliliters on top. One milli is 10 to the negative third power, so liters cancel out, and that comes out to be 1817.3 milliliters. Here, 5.2, 3.5, and 2.7 all have two significant figures. So, if we wanted two significant figures here, we would just write this as 1800 milliliters. So just remember, when we're dealing with one compound and we have different molarities, that's a strong indication that we're dealing with a dilution equation. So use the dilution formula and solve for the missing variable.
To what final volume would 100 mL of 5.0 M KCl have to be diluted in order to make a solution that is 0.54 M KCl?
If 880 mL of water is added to 125.0 mL of a 0.770 M HBrO4 solution what is the resulting molarity?
A student prepared a stock solution by dissolving 25.00 g of NaOH in enough water to make 150.0 mL solution. The student took 20.0 mL of the stock solution and diluted it with enough water to make 250.0 mL solution. Finally taking 75.0 mL of that solution and dissolving it in water to make 500 mL solution. What is the concentration of NaOH for this final solution? (MW of NaOH:40.00 g/mol).
Here’s what students ask on this topic:
What is a stock solution in chemistry?
A stock solution in chemistry is a concentrated solution that is prepared for the purpose of being diluted to a lower concentration for various laboratory uses. By having a stock solution, you can easily prepare solutions of different concentrations by adding a specific amount of solvent, usually water. This is particularly useful in experiments where precise concentrations are required. The process of dilution is governed by the equation , where and are the molarity and volume before dilution, and and are after dilution.
 Created using AI
Created using AIHow do you calculate the final concentration after dilution?
To calculate the final concentration after dilution, you can use the equation . Here, is the initial molarity, is the initial volume, is the final molarity, and is the final volume. Rearrange the equation to solve for the unknown. For example, if you know the initial concentration and volume, and the final volume, you can find the final concentration using .
 Created using AI
Created using AIWhat is the purpose of diluting a solution in a laboratory setting?
The purpose of diluting a solution in a laboratory setting is to achieve a desired concentration that is suitable for a specific experiment or application. Dilution allows scientists to work with solutions that are less concentrated, which can be necessary for accurate measurements, reactions, or analyses. By starting with a stock solution and diluting it, you can prepare multiple solutions of varying concentrations efficiently. This is particularly important in experiments where precise control over the concentration of reactants is required to ensure reproducibility and accuracy.
 Created using AI
Created using AIWhat is the equation used to describe the dilution process?
The equation used to describe the dilution process is . In this equation, represents the initial molarity (concentration) of the solution, is the initial volume, is the final molarity after dilution, and is the final volume after dilution. This equation helps in calculating the new concentration or volume when a solution is diluted by adding more solvent.
 Created using AI
Created using AIHow does dilution affect the molarity of a solution?
Dilution affects the molarity of a solution by decreasing it. When you add more solvent to a solution, the number of moles of solute remains the same, but the total volume of the solution increases. This results in a lower concentration of solute per unit volume. The relationship between the initial and final concentrations and volumes is given by the equation . Here, and are the molarity and volume before dilution, and and are after dilution. As the volume increases, the molarity decreases proportionally.
 Created using AI
Created using AIYour Introduction to Chemistry tutor
- The Environmental Protection Agency has set the limit for arsenic in drinking water at 0.010 ppm. To what volu...
- Determine the final volume, in milliliters, of each of the following: b. a 2.0% (m/v) LiCl solution prepared ...
- You need 500. mL of a 5.0% (m/v) glucose solution. If you have a 25% (m/v) glucose solution on hand, how many ...
- An aqueous solution that contains 285 ppm of potassium nitrate (KNO₃) is being used to feed plants in a garden...
- What is the concentration of a NaCl solution, in (m/v)%, prepared by diluting 65 mL of a saturated solution, w...
