Write an equation for the formation of each compound from its elements in their standard states, and find ΔH°rxn for each in Appendix IIB. a. NO2(g)
Ch.6 - Thermochemistry
Chapter 6, Problem 85
Hydrazine (N2H4) is a fuel used by some spacecraft. It is normally oxidized by N2O4 according to the equation: N2H4 (l) + N2O4 (g) → 2 N2O (g) + 2 H2O (g) Calculate ΔH°rxn for this reaction using standard enthalpies of formation.
 Verified step by step guidance
Verified step by step guidance1
Identify the standard enthalpies of formation (ΔH°<sub>f</sub>) for each of the reactants and products involved in the reaction. These values are typically found in a chemistry reference or textbook. For this reaction, you need the ΔH°<sub>f</sub> for N<sub>2</sub>H<sub>4</sub> (l), N<sub>2</sub>O<sub>4</sub> (g), N<sub>2</sub>O (g), and H<sub>2</sub>O (g).
Write down the balanced chemical equation: N<sub>2</sub>H<sub>4</sub> (l) + N<sub>2</sub>O<sub>4</sub> (g) → 2 N<sub>2</sub>O (g) + 2 H<sub>2</sub>O (g).
Apply the formula for calculating the standard enthalpy change of the reaction (ΔH°<sub>rxn</sub>): ΔH°<sub>rxn</sub> = ΣΔH°<sub>f</sub>(products) - ΣΔH°<sub>f</sub>(reactants). Remember to multiply the ΔH°<sub>f</sub> of each substance by its stoichiometric coefficient in the balanced equation.
Substitute the standard enthalpies of formation into the equation. Make sure to use the correct signs (positive or negative) as provided by the standard enthalpies of formation values.
Calculate the sum of the standard enthalpies of formation for the products and reactants separately, then find the difference between these sums to determine ΔH°<sub>rxn</sub>.

Verified video answer for a similar problem:
This video solution was recommended by our tutors as helpful for the problem above.
Video duration:
5mWas this helpful?
Key Concepts
Here are the essential concepts you must grasp in order to answer the question correctly.
Standard Enthalpy of Formation
The standard enthalpy of formation (ΔH°f) is the change in enthalpy when one mole of a compound is formed from its elements in their standard states. It is a crucial value used in thermodynamics to calculate the overall energy change in chemical reactions. Each substance has a specific ΔH°f value, which can be found in tables, and is essential for determining the enthalpy change of a reaction.
Recommended video:
Guided course

Enthalpy of Formation
Hess's Law
Hess's Law states that the total enthalpy change for a reaction is the same, regardless of the number of steps taken to achieve the reaction. This principle allows for the calculation of the enthalpy change of a reaction by summing the enthalpy changes of individual steps, making it possible to use standard enthalpies of formation to find ΔH°rxn for complex reactions.
Recommended video:
Guided course
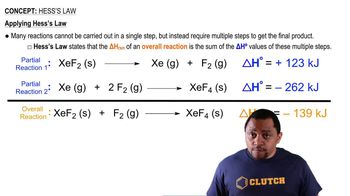
Hess's Law
Enthalpy Change of a Reaction (ΔH°rxn)
The enthalpy change of a reaction (ΔH°rxn) is the difference between the total enthalpy of the products and the total enthalpy of the reactants under standard conditions. It indicates whether a reaction is exothermic (releases heat, ΔH°rxn < 0) or endothermic (absorbs heat, ΔH°rxn > 0). Calculating ΔH°rxn using standard enthalpies of formation involves subtracting the sum of the ΔH°f values of the reactants from that of the products.
Recommended video:
Guided course

Enthalpy of Formation
Related Practice
Textbook Question
Textbook Question
Write an equation for the formation of each compound from its elements in their standard states, and find ΔH°rxn for each in Appendix IIB. b. MgCO3(s)
1906
views
Textbook Question
Write an equation for the formation of each compound from its elements in their standard states, and find ΔH°rxn for each in Appendix IIB. d. CH3OH(l)
435
views
Textbook Question
Pentane (C5H12) is a component of gasoline that burns according to the following balanced equation: C5H12(l) + 8 O2(g) → 5 CO2(g) + 6 H2O(g) Calculate ΔH°rxn for this reaction using standard enthalpies of formation. (The standard enthalpy of formation of liquid pentane is –146.8 kJ/mol.)
8542
views
3
rank
Open Question
Use standard enthalpies of formation to calculate ΔH°rxn for the reaction: CO(g) + H2O(g) → H2(g) + CO2(g)
Textbook Question
Use standard enthalpies of formation to calculate ΔH°rxn for each reaction. a. C2H4(g) + H2(g) → C2H6(g)
1002
views
