Here are the essential concepts you must grasp in order to answer the question correctly.
Beta Decay
Beta decay is a type of radioactive decay in which a beta particle (an electron or a positron) is emitted from an atomic nucleus. This process occurs when a neutron in the nucleus transforms into a proton, emitting an electron (beta-minus decay) or when a proton transforms into a neutron, emitting a positron (beta-plus decay). Understanding beta decay is crucial for writing balanced nuclear equations.
Recommended video:
Nuclear Equation
A nuclear equation represents the transformation of atomic nuclei during radioactive decay or nuclear reactions. It includes the symbols for the reactants and products, along with their atomic numbers and mass numbers. Balancing nuclear equations is essential to ensure that the number of nucleons (protons and neutrons) and charge are conserved during the reaction.
Recommended video:
Conservation of Mass and Charge
In nuclear reactions, the conservation of mass and charge states that the total mass number and total charge must remain constant before and after the reaction. This principle is fundamental when balancing nuclear equations, as it ensures that the number of protons and neutrons, as well as the overall charge, are equal on both sides of the equation.
Recommended video:
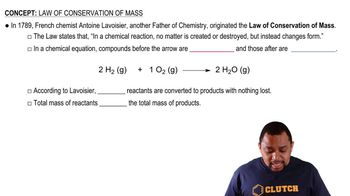
Law of Conservation of Mass