Graphing Exponential Functions - Online Tutor, Practice Problems & Exam Prep

Graphing exponential functions, such as \( f(x) = 2^x \), involves understanding key components like end behavior, intercepts, and asymptotes. The function is continuous and approaches a horizontal asymptote at \( y = 0 \) as \( x \) approaches negative infinity. Transformations, including shifts and reflections, can be applied to the parent function to graph more complex forms. The domain remains all real numbers, while the range depends on the asymptote's position, typically from \( k \) to infinity. Understanding these concepts is crucial for mastering exponential expressions and their properties.

Graphs of Exponential Functions

Hey, everyone. Whenever we graphed polynomial and rational functions, we had to consider a lot of different components. We looked at things like the end behavior and any intercepts of our graph and even had to consider and find multiple asymptotes sometimes when working with these functions. Now that we're faced with graphing exponential functions, you may be worried that there's going to be a lot of new and different things that we have to remember in order to fully graph them. But here we're going to walk through graphing the exponential function \( 2^x \) together, and I think you'll find that it's actually even more simple and more straightforward than graphing any polynomial or rational function. So let's go ahead and get started here.

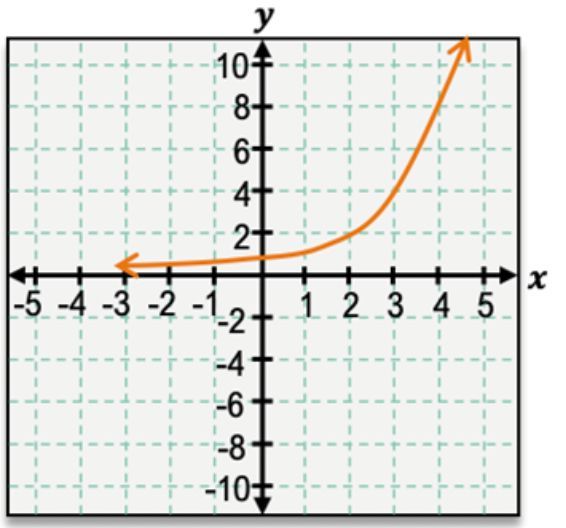
Now here we're going to be graphing \( 2^x \), so let's start by just plugging in some values for x and getting some ordered pairs to plot on our graph. So, starting with \( x = 0 \), if I plug that into my function, I get \( 2^0 \), which is simply 1 because anything to the power of 0 is 1. That first point I can plot is right at 1. Then moving on to \( x = 1 \), plugging that into my function, I get \( 2^1 \), which is simply 2. So, that next point is at 12 that I can plot on my graph. Then as x continues to get larger, I'm going to get \( 2^2 \), which is simply 4, and then \( 2^3 \) which is 8. And if I took it all the way up to \( x = 10 \), I would get an even larger number, this 1,024. So I can see that as x continues to increase, \( f(x) \) will continue to increase as well. So let's plot those last two points at 24 and at 38. Now looking at this side, I can go ahead and connect all of these points because I know it's going to increase. So I see that as x goes to infinity, \( f(x) \) also goes to infinity. That's my end behavior on that side.

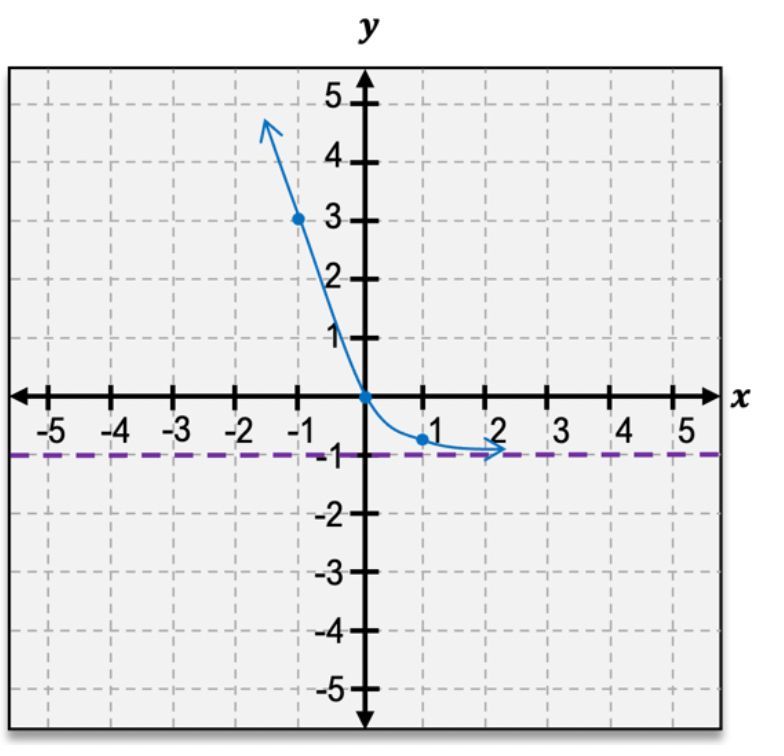
Now let's consider if x is negative. So starting with \( x = -1 \), if I plug that into my function, I get \( 2^{-1} \), which, knowing our rules for exponents, this is simply going to be \( \frac{1}{2} \). That point is at negative one, one half. And then as x gets more negative, \( -2 \) and \( -3 \), it continues to get smaller and smaller on that side. So we see that we're going to get really close to 0, but not quite 0. So I can go ahead and connect my points on this side. I see that I'm getting really close to that x-axis there. So as x approaches a negative infinity, I see that \( f(x) \) actually approaches 0. Now something I said there might have sounded familiar. We're going to get really close to 0 but not quite touch it because that's what we know as an asymptote. So we do have an asymptote. It is a horizontal asymptote right on that x-axis at y equals 0. Remember, we can plot asymptotes by using a dashed line. So here I have my dashed line right at y equals 0.

Now taking a full look at our graph here, looking first at this right side, I see that as we increase, we look kind of similar to what we've seen with polynomial functions. Now another similarity that our graphs of exponential functions have to polynomial functions is that they are always going to be continuous, meaning that there are no breaks in our graph. So we see that there are no breaks in this graph. It is continuous. Now looking at the other side of our graph here as we approach that asymptote, we know that we have asymptotes when dealing with rational functions. So another similarity that we may have to rational functions here is that our function is going to be 1 to 1 the way that some rational functions are. This just means that no 2 x's result in the same y or we could pass the horizontal line test. If I draw a horizontal line, it's only going to cross through one point on my graph.

Now looking at the complete shape of the graph of my exponential function, this is always going to be the shape. And a way that you can remember this is exponential function starts with e. So if I take a lowercase e and I just extend that tail out, it looks really similar to the shape of this graph. Now that we have a complete picture of our graph here, let's also consider the domain and range here. So the domain of any exponential function is actually always going to be the same. It's always going to go from negative infinity to positive infinity or simply all real numbers. This is the same for any exponential function. Now your range is a little bit more complicated, but it just depends on your asymptote. So it's always going to depend on the placement of our asymptote here. And here my asymptote is at 0, and I see that all of my y values are above that. So I know that my range here is going to go from 0 to infinity, but not including 0 because I am, of course, not touching that asymptote.

Now that we have a good picture of our graph of \( 2^x \), let's take a look at some more exponential functions. As we look at different exponential functions of the form \( f(x) = b^x \), we're going to see that the direction and the steepness of our graph is going to depend on the value of b, the base of our function. Looking at if b is greater than 1, like something like 2 or 5, I'm going to see that whenever I have that b greater than 1, my graph is always going to be increasing and going up on that side. Whereas if b is between 0 and 1, so it's something like 1 half or 1 fourth, I'm instead going to have a graph that decreases. Now, of course, also the steepness will depend on this value of b. And here we consider b greater than 1. You see that as we increase from 2 to 5, our graph gets steeper. And that's because for values of b, our base that are greater than 1 like 2 or 5, our graph is going to get steeper for larger values of b. Now it's actually the opposite when working with values of b between 0 and 1, those fractions, where we see that as we go from 1 half to 1 fourth on our graph, as we get smaller, it actually gets steeper there. So for values of b between 0 and 1, our graph is actually going to get steeper for smaller values of b, so the opposite of if b is greater than 1. So now that we have a complete picture of the graphs of our exponential functions, let's get some more practice. Thanks for watching and let me know if you have any questions.

Graphing Exponential Functions Example 1

Hey, everyone. In this problem, we're going to work through graphing the function f of x is equal to \( \frac{1}{2} \) to the power of x together. So let's go ahead and get started. Now we're just going to go through and plot some points by plugging in some values to our function. So let's start with x equals 0 and plug 0 into our function and see what we get. So if I take \( \frac{1}{2} \) and raise it to the power of 0, I know that anything raised to the power of 0 is 1 regardless of if it's a fraction or not. So my very first point is at 1, and I can go ahead and plot that on my graph. Then if I plug 1 in for x, I get \( \frac{1}{2} \) to the power of 1, which if it's raised to the power of 1, it just gets itself. So this is simply \( \frac{1}{2} \), and I could plot my second point at 1, \( \frac{1}{2} \). Then for 2, x equals 2, plugging that into my function, I get \( \frac{1}{2} \) raised to the power of 2. Now one raised to the power of 2 is just 1, so really I'm just doing \( \frac{1}{2^2} \), which is going to give me \( \frac{1}{4} \). So we're getting much smaller here, to \( \frac{1}{4} \) getting close to that x axis. And then finally, for x equals 3, if I put \( \frac{1}{2} \) and raise that to the power of 3, that's going to give me \( \frac{1}{8} \), which again is getting really small and close to that x axis over here. Now looking at our negative numbers, if I start to plug in some negative numbers for x starting with negative one, if I take \( \frac{1}{2} \) and raise it to the power of negative one, whenever we deal with whole numbers like 2 and raise them to a negative power, we end up with a fraction. Whenever we take a fraction and raise it to a negative power, we are instead going to end up with a whole number. So this is simply going to give me 2 because I just flipped the fraction. I get 2 over 1. That's just 2. So I can plot this point at negative 1, 2. Then if I take \( \frac{1}{2} \) and raise it to the power of negative 2, I'm going to get 4. So I can plot this point at negative 2, 4. Then for my last negative point, if I take \( \frac{1}{2} \) and raise it to the power of negative 3, I end up with 8 and I can, of course, go ahead and plot that point on my graph. Now we see that we have all of these whole numbers as we get into the negatives and then we had their corresponding fractions. We just flipped them under to the bottom of a fraction, and they're the same numbers because we're dealing with the same powers. Some are positive and some are negative. So some of these values will be fractions and some will be whole numbers, just like we saw for 2 to the power of x. Now, if you think about it, \( \frac{1}{2} \) to the power of x is really just 2 to the power of negative x. So that's why we're seeing so many similarities here. Let's go ahead and connect our graph. So on this side, going up and then on my right side here, I see that I'm getting really close to that x axis, not quite touching it though, which tells me I'm dealing with an asymptote. So I can go ahead and plot my asymptote here on this x axis because it is a horizontal asymptote right at y equals 0, and we can go ahead and denote that here our asymptote at y equals 0 plotted, of course, using a dashed line because it's an asymptote.

Now looking at our graph here, you might notice that it looks really similar to the graph of 2 to the power of x, which looks something like this. So now our graph of \( \frac{1}{2} \) to the power of x is kind of just this flipped, which makes perfect sense because we just said that this \( \frac{1}{2} \) to the power of x is the same exact thing as 2 to the power of negative x, so it makes sense that it's the same graph but flipped because it just underwent a transformation due to this negative sign here. Now let's go ahead and finish up here and get the rest of the information for this graph, like the domain and the range. So here, our domain of every single exponential function is always going to be the same. So here, it is the same as before, all real numbers. Now our range is dependent on where our asymptote is. And since our asymptote is again at y equals 0 and our graph is completely above that, we know that our range is going to go from our asymptote at 0 until infinity. So our range is 0 to infinity, using parentheses because the 0 is not included. Now that we have the entire picture of our graph of a \( \frac{1}{2} \) to the power of x, let's get some more practice.

Transformations of Exponential Graphs

Hey, everyone. We now know how to graph basic exponential functions of the form f(x)=bx, where b is just some number like 2 or 4 or 12 or whatever. But what if we're given a more complicated exponential function like this g(x) that we have here? How are we going to graph that? Well, you may be worried that we're going to have to take a bunch of different values for x and plug them in and do some messy calculations. But here, I'm going to show you that we're not going to have to do a single calculation because we can simply apply rules of transformations to our parent function bx that we already know how to graph. So all we're going to be doing here is taking the graph of a function that we already know how to get and picking it up and moving it around in order to get the graph of our new, more complicated function. So let's go ahead and get started here.

Now when working with this g(x) here, we see that it looks really similar to 2x but just with a couple more things added in. So we have this negative one and this negative four, both of which represent transformations. Now when working with exponential functions, we're going to be focused on 2 types of transformations in particular, reflections and shifts, because they are the most commonly occurring transformations when working with exponential functions. So just as a reminder, whenever we have a negative on the outside of our function, that represents a reflection over the x-axis, whereas a negative on the inside of our function represents a reflection over the y-axis.

Now when we're working with h and k, this h here represents a horizontal shift. Remember, h horizontal. And then k, of course, represents a vertical shift by some number of k units. So let's go ahead and start graphing our function g(x) here starting with our step 0. Now step 0 is actually to identify and graph our parent function. And this step is really important because we always, always want to graph our parent function first when transforming exponential functions.

So let's go ahead and take a look at our g(x) here. I said that it looks really similar to 2x, and that represents our parent function. So our parent function here is f(x)=2x. And let's go ahead and plot this using a couple of different points. So the first point that we have is going to be negative one, one over b. Now b is the base of our parent function, which here is 2. So my first point will be at negative one, one over 2.

Then my second point will be at 1, which will be the same no matter what your parent function is. And then finally, I'm going to plot one final point at 1b, which remember b is the base, so in this case, 2. So let's go ahead and plot these three points on our graph. So first, negative 1, 1-half, then 0, 1, and finally 1, 2. Now we wanna go ahead and connect all of these points using the shape of our exponential graph. And then we want to do one more thing to get that parent function completely graphed, and that is plot our horizontal asymptote at y=0. That asymptote will always be at y=0 no matter what your parent function is. So we're using a dash line here because it is an asymptote.

Now that we have that parent function graphed, we can go ahead and move on to actually graphing our new transformed function, g(x). So looking at that first step, step 1, we want to go ahead and shift our horizontal asymptote that we just drew. So looking at my function g(x), I'm going to want to shift my horizontal asymptote to y=k. Now when looking at a transform function, I know that k is just added to the end of my function. And here I have this negative 4, so I know that my k value is going to be negative 4. So let's go ahead and shift that horizontal asymptote all the way down to negative 4, of course, still using a dash line because it is an asymptote.

So with step 1 done, we have our horizontal asymptote plotted. Let's move on to step 2 and determine if there is a reflection happening. Now remember, a reflection happens when we have a negative on the inside or the outside of our function. And looking at my function, g(x), it looks like I did not have a negative get inserted into my new function, so I don't have to worry about a reflection. And I can move on to the second part of step 2, part b, to shift our test points by h and k. Now we're going to go ahead and identify h and k. We know that k is already negative 4 because we just moved our horizontal asymptote. And then for h, I have this x-1 here. Remember, when dealing with transformations, it's x-h. So here, my h value is simply 1.

Now we're going to go ahead and take those points from our parent function, our test points, and shift them 1 over to the right and 4 units down in order to get the points of our new transformed function. So starting with this first point here, I'm going to go one over and then 1, 2, 3, 4 down to get my new point. Then for my second point, one over again And then 1, 2, 3, 4 down to get my new point. And then my last point, 1 over. And then 1, 2, 3, 4 down to get my new point. So I have all of my new transformed points from my original function. And my very last step, my step 3 here, is just to go ahead and connect everything and, of course, approach my asymptote.

So here I can go ahead and connect my points and then approach my asymptote on this side. So here we can see that our original function just got picked up. It got shifted over and down a little bit in order to get our new function. So now that we have fully transformed our function, we have our graph of g(x), we can go ahead and identify our domain and our range. Now remember, the domain of any exponential function is always going to be the same. It's always going to go from a negative infinity to infinity or simply all real numbers.

Now for our range, this depends on our placement of our graph regarding our asymptote. So here, if our graph is above our asymptote, which here it is, we can go ahead and state that our range goes from k, that value of k, to infinity. Now here my value of k is negative 4, so I know that all of my y values are going to start at negative 4 and go to infinity. So here it is from k to infinity negative 4. Now because this one is above, that's what our range is. But if it happened to be below, our range would instead go from negative infinity to k, just depending on what's happening with our graph.

Now that we know how to transform exponential functions, let's get some more practice. Thanks so much for watching.

Transformations of Exponential Graphs Example 1

In this problem, we want to graph our given function g(x) as a transformation of f(x) which is equal to 12x. The function I have g(x) is -12x+3. The first thing that you might notice here is that we have a negative sign. And I know that I've said before that the base of an exponential function cannot be negative, and this does not violate that. So I have this negative sign outside of these parentheses. If this was -12 to the power of x, that's when we would have a problem. So I just wanted to take a second to address that. It's totally okay if your negative is on the outside of your base as long as it's not being raised to that power because here we're taking one half, raising it to the power of x, and then making it negative, and that's what makes this okay. So let's go ahead and get right into graphing, starting with step 0.

Step 0 is to identify and graph our parent function, f(x) = b to the power of x. We know here that our parent function is 12x. So, going ahead and plotting the points that I need, I want to graph negative 1 over b which is just negative one two, 1, and then 1 over b, where b here is one half. So let's go ahead and plot these three points: (-12, 0), (1, 1), and (1, 1/2). Now we can go ahead and connect all of these. And then, of course, we want to plot our horizontal asymptote at y = 0, which is just at our x-axis using a dashed line. So we have our parent function here.

Let's go ahead and get into our new function and transform this parent function. Our new function that we have is -12x+3. The very first thing I want to do is shift my horizontal asymptote to y = k. Here, I have this plus 3 on the end, which is my value for k. So I know that I need to have my horizontal asymptote at y = 3. Let's go ahead and put our horizontal asymptote using a dashed line on my graph up here at y = 3.

Now that we're done with step 1, we can move on to step 2 and decide if there is a reflection happening. Remember, reflection happens if we have a negative outside or inside of our function, and we do have this negative added to the outside of our function. So that tells us that, yes, we do have a reflection, which means that we need to take those test points from our parent function and reflect them here over the x-axis because that negative is outside of our function. So let's go ahead and do that here. Taking my points, so starting with this point, I'm going to reflect that over the x-axis. So my new point will end up here. Same thing for this one here, going to end up right here. And then my last point right here. So I have reflected my new points, but now I need to shift them.

Remember, we shift them by h, k. Here, we don't have a value for h, it's just 0, so I don't have to worry about any horizontal shift. But I do have a vertical shift by k. So we need to take all of these points and move them up by 3. So let's go ahead and do that. Shift up by 3, shifting. And then my other point here by 3, and then my last point up by 3 as well. So now I have my final points that I can go ahead and sketch and connect these points using a curve, of course, approaching my asymptote. So here we're approaching that asymptote and then on this side as well. So we have our new function here. We have completed graphing it, but let's go ahead and identify this additional information, our domain and range.

Remember, our domain will always be the same, no matter what. It's always going to be all real numbers, so I don't have to decide anything or figure anything out there. But for my range, I need to look at whether my function is above or below the asymptote. Now, since my asymptote is right here and my function is down here, that tells me that it is below my asymptote. So my range is going to go from negative infinity until we reach that asymptote at k, which in this case is at 3. So here, my range is from negative infinity to 3. Now that we've identified all of that information, let's get into some practice.

Graph the given function.

$g\left(x\right)=4^{-x}-1$

• A.
• B.
• C.
• D.

The graph for the function $f\left(x\right)=3^{x}$ is given below.

Match the given function, $g\left(x\right)$, to its graph. $g\left(x\right)=-3^{x+2}+1$

• A.
• B.
• C.