Table of contents
- 0. Review of Algebra4h 16m
- 1. Equations & Inequalities3h 18m
- 2. Graphs of Equations43m
- 3. Functions2h 17m
- 4. Polynomial Functions1h 44m
- 5. Rational Functions1h 23m
- 6. Exponential & Logarithmic Functions2h 28m
- 7. Systems of Equations & Matrices4h 6m
- 8. Conic Sections2h 23m
- 9. Sequences, Series, & Induction1h 19m
- 10. Combinatorics & Probability1h 45m
1. Equations & Inequalities
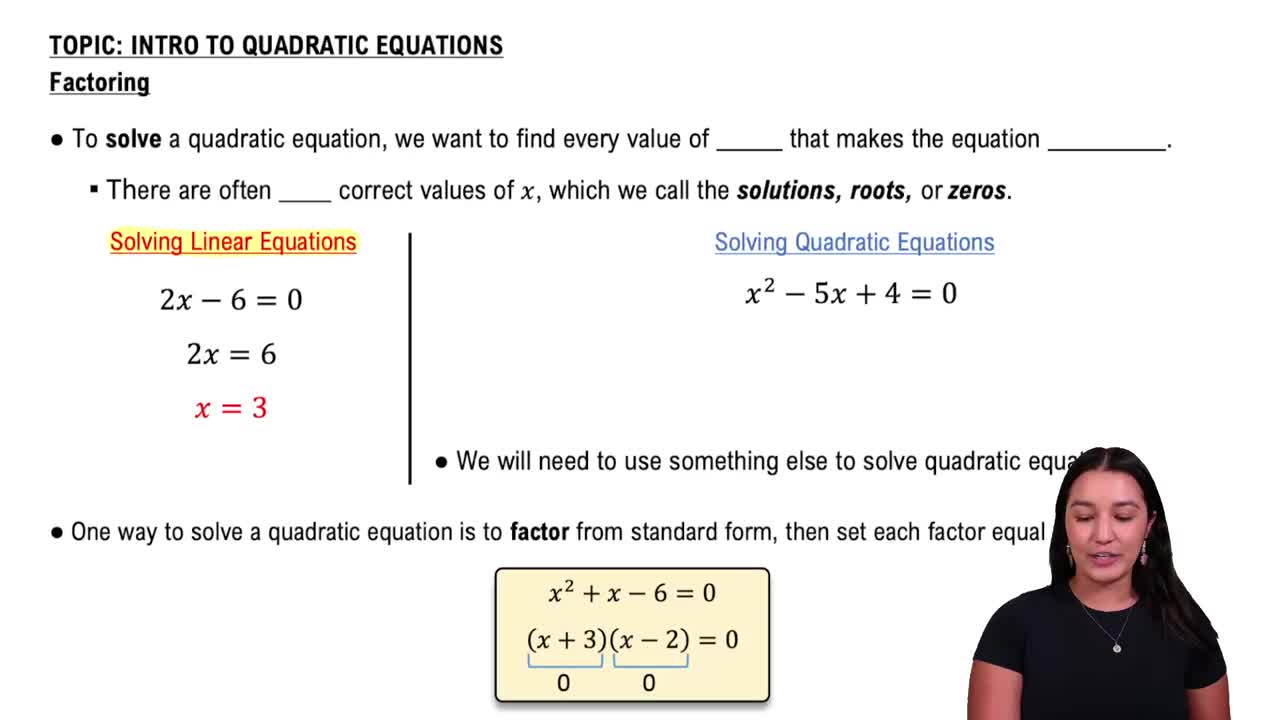
Intro to Quadratic Equations
Problem 16c
Textbook Question
Solve each equation using the zero-factor property. See Example 1. 2x^2 - x = 15
 Verified step by step guidance
Verified step by step guidance1
Rewrite the equation in standard form by moving all terms to one side: \(2x^2 - x - 15 = 0\).
Factor the quadratic equation. Look for two numbers that multiply to \(-30\) (the product of \(2\) and \(-15\)) and add to \(-1\) (the coefficient of \(x\)).
Once you find the numbers, rewrite the middle term \(-x\) using these numbers to split it into two terms.
Factor by grouping. Group the terms into two pairs and factor out the greatest common factor from each pair.
Apply the zero-factor property: Set each factor equal to zero and solve for \(x\).
Recommended similar problem, with video answer:
 Verified Solution
Verified SolutionThis video solution was recommended by our tutors as helpful for the problem above
Video duration:
2mPlay a video:
Was this helpful?
Key Concepts
Here are the essential concepts you must grasp in order to answer the question correctly.
Zero-Factor Property
The Zero-Factor Property states that if the product of two or more factors equals zero, then at least one of the factors must be zero. This principle is essential for solving polynomial equations, as it allows us to set each factor equal to zero to find the solutions. For example, if we have an equation like (x - 3)(x + 2) = 0, we can conclude that x = 3 or x = -2.
Recommended video:
Guided course

Introduction to Factoring Polynomials
Quadratic Equations
A quadratic equation is a polynomial equation of the form ax^2 + bx + c = 0, where a, b, and c are constants, and a ≠ 0. These equations can be solved using various methods, including factoring, completing the square, or using the quadratic formula. In the given problem, we first need to rearrange the equation into standard form before applying the zero-factor property.
Recommended video:

Introduction to Quadratic Equations
Factoring Polynomials
Factoring polynomials involves expressing a polynomial as a product of its factors. This process is crucial for solving equations, as it simplifies the equation to a form where the zero-factor property can be applied. For instance, in the equation 2x^2 - x - 15 = 0, we would factor it into (2x + 5)(x - 3) = 0 to find the values of x that satisfy the equation.
Recommended video:
Guided course

Introduction to Factoring Polynomials

 5:35m
5:35mWatch next
Master Introduction to Quadratic Equations with a bite sized video explanation from Callie
Start learningRelated Videos
Related Practice