Here are the essential concepts you must grasp in order to answer the question correctly.
Perfect Square Trinomial
A perfect square trinomial is a quadratic expression that can be expressed as the square of a binomial. It takes the form (a ± b)² = a² ± 2ab + b². Recognizing this structure is essential for transforming a given binomial into a perfect square trinomial by adding the appropriate constant.
Recommended video:
Solving Quadratic Equations by Completing the Square
Completing the Square
Completing the square is a method used to convert a quadratic expression into a perfect square trinomial. This involves taking half of the coefficient of the linear term, squaring it, and adding it to the expression. This technique is crucial for determining the constant needed to achieve a perfect square trinomial.
Recommended video:
Solving Quadratic Equations by Completing the Square
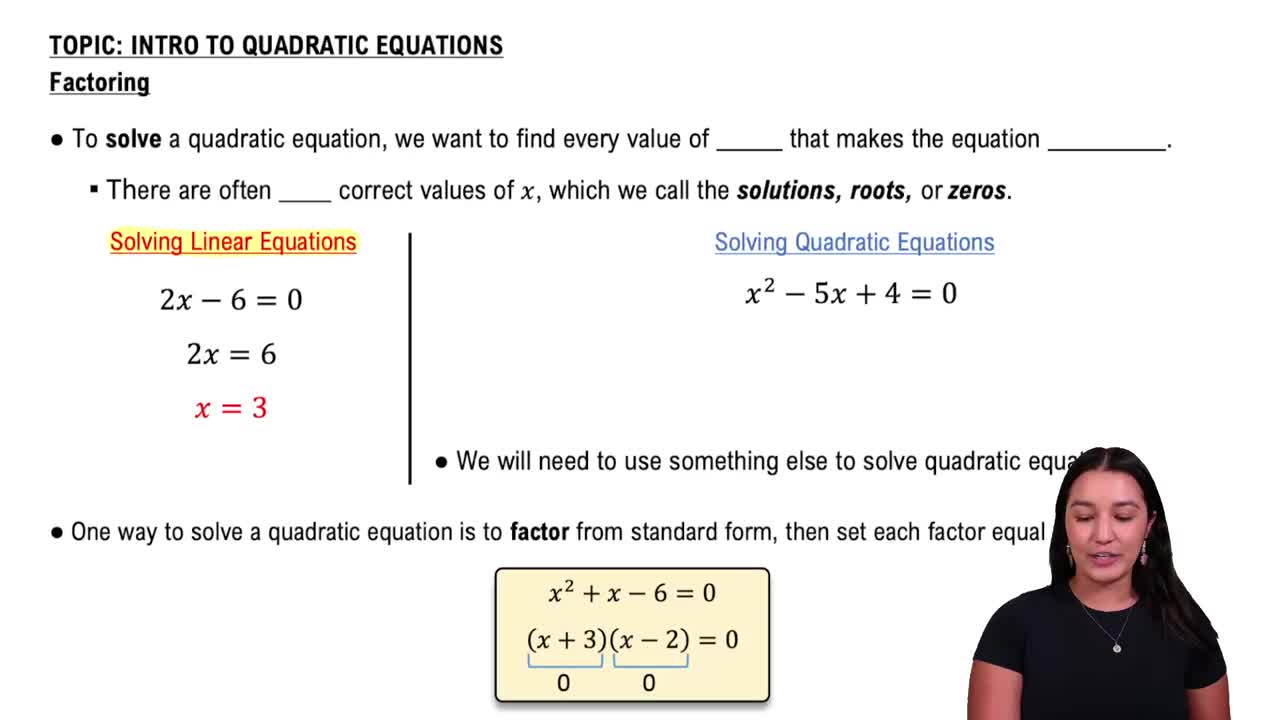
Factoring Quadratics
Factoring quadratics involves rewriting a quadratic expression as a product of its linear factors. For perfect square trinomials, this means expressing the trinomial in the form (a ± b)². Understanding how to factor these expressions is important for simplifying and solving quadratic equations.
Recommended video:
Solving Quadratic Equations by Factoring
 Verified step by step guidance
Verified step by step guidance Verified Solution
Verified Solution


 5:35m
5:35m