Table of contents
- 0. Review of Algebra4h 16m
- 1. Equations & Inequalities3h 18m
- 2. Graphs of Equations43m
- 3. Functions2h 17m
- 4. Polynomial Functions1h 44m
- 5. Rational Functions1h 23m
- 6. Exponential & Logarithmic Functions2h 28m
- 7. Systems of Equations & Matrices4h 6m
- 8. Conic Sections2h 23m
- 9. Sequences, Series, & Induction1h 19m
- 10. Combinatorics & Probability1h 45m
0. Review of Algebra
Exponents
Problem 22
Textbook Question
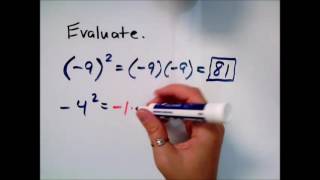
Determine whether each statement is true or false. |-5| * |6| = |-5*6|
 Verified step by step guidance
Verified step by step guidance1
Step 1: Understand the absolute value concept. The absolute value of a number is its distance from zero on the number line, regardless of direction. Therefore, the absolute value of any number is always non-negative.
Step 2: Calculate the absolute value of each number separately. For example, \(|-5|\) is 5 and \(|6|\) is 6.
Step 3: Multiply the absolute values obtained in Step 2. So, calculate \(5 \times 6\).
Step 4: Calculate the absolute value of the product of the numbers. First, find the product \(-5 \times 6\), which is -30, and then find its absolute value, \(|-30|\).
Step 5: Compare the results from Step 3 and Step 4. If they are equal, the statement is true; otherwise, it is false.
Recommended similar problem, with video answer:
 Verified Solution
Verified SolutionThis video solution was recommended by our tutors as helpful for the problem above
Video duration:
2mPlay a video:
Was this helpful?
Key Concepts
Here are the essential concepts you must grasp in order to answer the question correctly.
Absolute Value
The absolute value of a number is its distance from zero on the number line, regardless of direction. It is denoted by vertical bars, such as |x|, and is always non-negative. For example, |-5| equals 5, as it measures the distance of -5 from 0.
Recommended video:

Parabolas as Conic Sections Example 1
Properties of Absolute Values
One important property of absolute values is that the absolute value of a product is equal to the product of the absolute values. This means |a * b| = |a| * |b| for any real numbers a and b. This property is crucial for simplifying expressions involving absolute values.
Recommended video:

Change of Base Property
Multiplication of Real Numbers
Multiplication of real numbers follows specific rules, including the commutative property (a * b = b * a) and the associative property ((a * b) * c = a * (b * c)). Understanding these properties helps in manipulating and simplifying expressions involving multiplication, especially when combined with absolute values.
Recommended video:

Introduction to Complex Numbers

 7:39m
7:39mWatch next
Master Introduction to Exponent Rules with a bite sized video explanation from Patrick Ford
Start learningRelated Videos
Related Practice