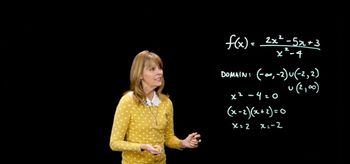Hi, my name is Rebecca Muller. Today, we're going to look at the topic of rational functions. Now, if you look at the root word of rational, you think of the word ratio, and you know a ratio can be thought of as a fraction if you're thinking about numbers. Well, you might say, OK, great, so rational functions, then, would be functions that are written as fractions. And you'd technically be correct, but it's a little bit more detailed than that. So let's look at a technical definition Now of what a rational function is. A rational function in terms of x can be written in the form r of x equals f of x divided by g of x, where f of x and g of x are both polynomials and g of x does not equal 0. So notice that what you have here is a rational function, which is really just a quotient of two polynomial functions. Now technically, every polynomial function, then, is a rational function, because you can always write a function f of x as f of x divided by 1. So you can think of that as being a rational function, and that's going to make sense when we start looking at similarities between the set of rational functions, that is, and the set of polynomial functions. But there are also some differences that we'll be bringing to the forefront. And therefore, I'm going to concentrate on those rational functions during this session where the denominator is going to end up being something at least linear so that we don't end up just having a constant in the denominator. Well, of course, constant could be linear also. But I mean, we have to have a degree 1 in the denominator in order to be talking about it. So let's look at the specific topics we're going to look at. We're going to start by looking at finding the domain of rational functions. Then, we're going to move on to looking at graphs of the format y equals 1 divided by x to the nth power, where our value could be 1, 2, 3, and so on and so forth. Then, we'll look at identifying asymptotic behavior in rational graphs. And finally, we'll look at sketching the graph of a rational function. So we're going to start with the one dealing with finding the domain. So let's look at a few examples now. If we look at a function-- and I'm going to have one. We'll call it f of x. And that's going to be 2x squared minus 5x plus 3 divided by a denominator of x squared minus 4. We can see that this is a rational function. Because by definition, the numerator is a polynomial, and the denominator is also a polynomial. If all I'm interested in is determining the domain, then we have to think about the fact that when we're working with fractions, we can't allow division by 0. That means that in this expression, I have to make sure that the denominator is not equal to 0. Now, in order to figure out that it's not equal to 0, we're going to approach it in a counterintuitive way in that we're going to say, well, when is it equal to 0? So to figure out the domain, I'm going to take the denominator x squared minus and set it equal to 0. And this can be solved by factoring. We can say that's x minus 2 times x plus since x squared minus 4 is a difference of two squares. And I come up with two results, x equals and x equals negative 2. That means that the domain has to be all values other than these two values. If I substituted in either of these, you can see that substitution into the denominator will make that denominator equal to 0. To write that in interval notation, we can say that we're going to begin our domain at negative infinity. We're going to keep going until we end up at the first of those two. Think about it on a number line. It's going to be a negative 2. Then, we're going to eliminate negative 2, and we can do that just like that, just by writing parentheses around the negative on both sides of the interval notation so that the only thing that's left out is the single value of negative 2. That continues until we end up at positive 2. And then, we're going to pick up from there at positive and go all the way to infinity. So our domain is from negative infinity to negative non-inclusive, union negative 2 to 2 non-inclusive, union 2 to infinity non-inclusive. It's time for a quick quiz. Since a rational function is the quotient of two polynomial functions, the domain of a rational function can be found by determining where the polynomial in the blank is equal to blank. How should we fill in the blanks. Should it be A? The polynomial in the numerator is equal to 0. Should it be B? The polynomial in the denominator is equal to 0. or should it be C? The polynomial in the numerator is equal to the denominator. Choose from A, B, and C now. you're correct, the answer is B. To find out the domain of a rational function you want to determine where the polynomial in the denominator is equal to 0. Sorry the answer is B. To determine the domain of a rational function you want to determine where the polynomial in the denominator is equal to 0. We know that division by 0 is undefined therefore if we set the denominator of a rational function equal and we come up with certain values that make the denominators then we need to eliminate those values from our domain. Let's look at a second example now. And I'm going to start with a g of x. This g of x is going to equal 4x divided by 4x plus x squared. Again, what we're really interested in for domain is values for which this function will be defined. And again, we'll figure those values out by figuring out where it's undefined. This will be undefined wherever the denominator is equal to 0. So we'll take 4x plus x squared set it equal to 0. Notice I can factor out an x, leaving me with 4 plus x equals 0. And then setting each factor equal to gives us x equals 0 and x equals negative 4. The domain will be all values other than these two. So we'll start at negative infinity. We'll go all the way to negative 4. Then, we'll pick up at negative 4 and go till we get to 0. And then, we'll pick up at 0 and go to positive infinity. One more example. Let's look at an h of x. Our h of x is going to equal x cubed minus 4x squared divided by x squared plus 4. Once more, all we're really interested in is where this fraction could be undefined, which would be where the denominator is 0. Well, if I set x squared plus 4 equal to 0, that would mean that x squared would have to equal negative 4. And we know that any positive number squared gives us a positive result. 0 squared gives us 0. Negative numbers squared give us a positive result. There is no real number for which x squared could equal negative 4. And therefore, the domain for this rational function would be the set of all real numbers from negative infinity to positive infinity. Now, a good place to begin with we're starting to look at graphs of rational functions would be with the functions of the form over x to the nth power, where the n value is going to end up being 1, 2, 3, and so on and so forth. So let's look at f of x equals 1 divided by x to the nth power for n equal to 1 to start with. And then, I'm going to end up also looking at what happens when I look at n equal to 2. And then, we'll generalize from there. So when we look at 1 over x, which is how I'm going to begin-- so f of x equals 1 divided by x-- because we really don't have anything to go on here, we don't know anything about this functional graph at this point, we're going to use the technique of plotting points. So just a quick sketch-- let's give ourselves axes to work with. And let's just substitute in values. Now, obviously, we cannot let x equal 0, because that would give us a division by 0, which is undefined. So just starting with the value of 1, if I let x equal 1, then notice that my y value is going to be 1 divided by 1, which is also 1. If my x-value is 2, we end up with 1/2. If the x-value is 3, we end up with the value 1/3. If x is equal to 4, the value is 1/4. And so you should be seeing a trend here. Notice that as x becomes larger and larger, the value of 1 over x becomes smaller and smaller and closer and closer to 0. So I'm going to connect these dots. But it never ends up hitting, because it cannot equal 0, since the numerator is a 1. A fraction can't be equal to 0 unless the numerator is 0. Notice there are some other numbers in here between 0 and 1. So in particular, what happens if we substitute in the value of 1/2? Well, we're taking a reciprocal here, so the reciprocal of 1/ is 2, so I end up with the point 1/2, 2. What about 1/3? We end up with the reciprocal of 1/3, which is going to equal 3. The reciprocal of 1/4 is equal to 4. And as we get closer and closer to 0 for our x-values, the reciprocal of those values ends up increasing, increasing without bound. Now, one other thing that we can notice is that if I check for symmetry here, f of negative x is going to equal 1 divided by negative x, which is, written another way, negative 1 over x. Remember that when we end up getting f of negative x equals the negative of what we got when we had f of x, that means we have odd symmetry. So this graph is going to be symmetric to the origin. And what that means is whatever happened in quadrant I is also going to happen in quadrant III. And I'm going to give myself a couple of points here just as reference, and I'm going to connect the dots heading off in this direction, heading off down in this direction. Now, this is the first graph that we're going to see with this asymptotic behavior. What we end up having is the horizontal line, which is our x-axis, whose equation is y equals 0, is going to be what's called a horizontal asymptote period to the graph, indicating that as my values of x get larger and larger without bound in the positive direction and in the negative direction, we note that the graph gets closer and closer to resembling this horizontal line. Now, it never ends up hitting that horizontal line, but the graph gets closer and closer and closer. Likewise, we notice that we have the graph getting closer and closer to the y-axis. That equation is x equals 0. As x becomes closer and closer to 0, my y-values go off in the positive direction for positive values of x and off in the negative direction for negative values of x. And so we end up having what's called a vertical asymptote at the value x equals 0. Now, one thing that's important here is to note that asymptotes in these cases are going to be equations of lines. So we have-- again, you don't say that you have an asymptote at 0. You say that the horizontal asymptote is equals 0, and the vertical asymptote is x equals 0. So now, I want to look at the second version, and that is where I substitute in the value of for n in this format. So we're going have f of x equals divided by x to the 2nd power. Now, we're going to, again, start off by plotting points. So let's make a quick sketch. Here's my y-axis. Let's make this our x. And we're going to substitute some values in. Once more, notice that the domain would be the set of all real numbers except for 0. When we substitute in x equal to 1, we end up with 1 divided by 1 squared, which is 1. So for x equal to 1, y equals 1. If x is equal to 2, we end up with 1 over 2 squared, which is going to be 1/4. So notice that this is going to start approaching that axis a lot faster. If x is equal to 3, we end up with 1/3 squared, which is 1/9. And I'm almost to the point where I just have to do big dots, so you can see what's going on. But notice here it's going to continue to get closer and closer for larger and larger values of x. And what we kind of do is just draw it so it kind of looks like it's going to be approaching. And I notice I even put like only half an arrow in. For values between 0 and 1, let's just try a few. If I start off with 1/2, notice we're going to end up with 1 over 1/2 squared. So we think of it as reciprocal. But now, we're going to have 2 squared, which is going to be 4 to give us our result. And I'm not even going to have space to put what happens when I plug it in 1/3. 1/3 squared is 1/9, the reciprocal of which is 9. So you can see that again, it's going to have the same type of shape. But of course, we're not going to the same values, and this is going to be rising a lot quicker than the one over here was. Let's more explore, look at what's going to be going on as far as symmetry is concerned. So we're going to look at f of negative x. That's going to be 1 over negative x squared. That's going to equal 1 over x squared. So we end up with the same result, the same y-value for negative x values. And that means that we're going have a symmetry to the y-axis, so symmetric to the y-axis. Now, since we know what quadrant I looks like, symmetric to the y-axis-- we think of flipping that-- we're going to end up in quadrant II. And I'm going to try to sketch this in-- give myself a little bit of reference here-- coming out this way and then, again, going off in this direction. And one more time what you can notice is that we're going to end up having a horizontal asymptote in that the graph is getting closer and closer to the x-axis. So we have a horizontal asymptote at y equals 0. And we can notice that the y-axis, whose equation is x equals 0, is going to be a vertical asymptote. So I'm going to start using some abbreviations now. Horizontal asymptote, I'm going to write HA. And vertical asymptote, I'll write VA. And so we had that in both cases. We had the horizontal asymptote and the vertical asymptote. And so when we're looking at graphs of the format f of x equals 1 over x to the n, we can see that we're going to have-- for power equal to 1, we have something that looks like this. For power equal to 2, we have something that looks like this. What about 1 over x cubed? Well, even though the numbers aren't going to be the same because of the odd exponent, we're going to have this odd nature to it where it's symmetric to the origin. For powers that are even, we're going have the even nature to it that is symmetric to the y-axis. Well, now that we're familiar with functions of the format over x to the nth power, we're going to now be able to use your prior knowledge about transformations in order graph functions of the form f of x equals a divided by x minus h quantity to the nth power plus k for values of equal to 1, 2, 3, and so on and so forth. Let's look at a couple of examples of that now. So the first one I'm going to consider is f of x equals negative 1 over x minus 2 quantity squared plus 3. Now, this is going to end up being a transformation of the function 1 over x squared. So let's identify what will happen to that graph. Remember that this minus 2, which is going to be an impact on the x-value, is going to end up giving us a horizontal shift. And because we end up in our format as x minus 2, recall that this is a shift to the right of 2 units. So we're going to end up going right 2 units. The minus 1, which is in the numerator here, well, that's going to be a multiplication by negative 1. So that's going to end up being a reflection. And we're going to reflect across the x-axis. And then finally, what happens when you have the plus 3 there? Well, the plus 3, that's added after the functional format takes place. So this is going to be a shift up 3 units. So let's put all of that together, and again, we're just trying to get a quick sketch of what it's going to look like. So remember, the original format was 1 over x squared. So in your mind, you should kind of be thinking, OK, 1 over x squared, that's this one. It's the one that used to be in the first and second quadrants. I'm going to take that graph-- and let's just give myself a little bit larger scale here. Now, I'm going to worry about the reflection in a moment. But let's just note that if we move up three units, that's going to affect the horizontal asymptote. So that horizontal asymptote is going to move up three units. So I'm going to end up at y equals 3, and that's going to be a horizontal asymptote. And then, this shift to the right of 2 units, that's going to affect the vertical asymptote that used to be on the y-axis. So we're going to end up shifting it to the right 2 units. And again, let's try to get this semi-straight. And now, the graph is going to be reflected across, to begin with, the x-axis. So notice instead of being above the horizontal asymptote, it's going to end up being below the horizontal asymptote. Remember, the reflection would have been done prior to moving it upward. So that means that I'm going to end up having it just below the horizontal asymptote in these two areas. I mean, one thing you can do very quickly is to find the y-intercept, if you want to, in order to figure out where that's going to be. So I can evaluate f of 0. By substituting in 0, we're going to end up with negative 1 divided by negative squared, which is 4. So then I get negative 1/3 plus 3, or 3 minus 1/4, so very, very close here. You could then just quickly sketch in this graph, only do it right down here. So we're going to end up having it approaching horizontal asymptote and then approaching the vertical one. And same thing happening over here-- approaching the horizontal asymptote, approaching the vertical one. And I end up with a quick sketch for this graph, which just has transformations going on. Let's try one more example of that. This time, I'm going to call it g of x, and this is going to equal 3 divided by x minus 1. Now in this format, notice that the minus 1, which is applied after we take reciprocals, is going to end up being a downward shift. And the 3 that is going on here is like multiplication by 3. It's 3 times 1 over x. So therefore, this is going to be a stretch of the function. It's going to end up getting closer to the y-axis faster. So we'll end up doing a quick-- again, a quick sketch of this. And really what's important here is to get the asymptotes in the right place. Because we've shifted down one unit, that horizontal asymptote that used to be on the y-axis also shifts down one unit. So if I go down to negative 1, I end up with a horizontal asymptote that y equals negative 1. I do not have a shift right or left, so therefore, I still have my vertical asymptote at x equals 0. And the stretching is kind of hard to do without plotting a few points. I mean, one thing you could do is substitute some values in to see what occurs. So for instance, if I substitute in a value of 1, I end up with 3 divided by 1, which is 3. 3 minus 1 is 2. I could substitute in a value of 2. That's going to give me 3/2, which is 1 and 1/ minus 1, which is 1/2. And we're just trying to get a sketch of this, so what occurs is we know that the normal picture, y equals 1 over x, quickly looks like this in relation to the first and third quadrants. And so here, we're going to end up connecting the dots. Let's just kind of go off toward the horizontal asymptote and then upward here. And now, we still want the same orientation. So what was in the first quadrant was flipped over and then flipped down to get into the third quadrant. This time, it's just going to be in relation to the asymptotes. So we're going to flip over and then flip down, and we end up with a sketch of the other portion of it that looks something like that. And so we can identify the graphs of these two by using the transformations of the ones that we're most familiar with, the 1 over x and the 1 over x squared. Now, we're going to look at some rational functions that have a slightly different format. For our first example, let's look at the function f of x equals-- we'll have a numerator of x squared minus 4. In our denominator, we'll have x squared minus 5x minus 6. Now, one of the first things that we want to consider would be the domain of this function. So to find the domain of the function, recall that we're going to look at where the denominator would be equal to 0 and then eliminate those values. So if we start with x squared minus 5x minus 6 equals 0, we can factor this trinomial into a product of two binomials. Notice that we can take the constant term of 6, and we can look at its factors, 1 and 6 or 2 and 3. Well, we need those to have different signs, so we're going to use the 1 and the and have the negative on the 6 and the plus sign on the 1. So if we set each factor equal to 0-- we'll do that in one step-- we end up with x equal and x equal to 6. We now need to eliminate those values from our domain. So our domain will end up being the set that begins where we got negative infinity, goes all the way to negative 1, and we'll eliminate that value and pick up right there that negative 1, go all the way to 6, and then eliminate that value, and then continue on to infinity. So again, in interval notation, it's negative infinity to negative 1 exclusive union, that is; negative 1 to 6, union 6 to infinity, not including any of the endpoints. Now, one more thing we'd like to do before we start looking at the graph of this is to consider the functional value if it were factored completely. So we'll go ahead and look at f of x equals-- and now, I'm going to factor the numerator. That's a difference of two squares. So we're going to have x minus 2 times x plus 2. And our denominator we just factored a few moments ago as x plus 1 times x minus 6. It turns out that sometimes, it's going to be easier to use this factored format than it is the one that's multiplied out. And the other thing that we'd be considering is whether we had any common factors. We would want to make sure that we take care of those before we continue on. In this case, we can see there were no common factors that we need to work with. Now with rational functions, we know we need to worry about asymptotic behavior. We're going to end up with a vertical asymptote whenever our denominator is getting closer and closer to 0, because what happens then is that our entire fraction is getting larger and larger without bound either in the positive or the negative direction. So we've made sure that this is in reduced format, which is going to be important. And I'll do an example in a few minutes where you will see what happens if it's not. But right now, we're going to end up having a vertical asymptote for each 0 of the denominator. That means that if I set each of these values equal to 0, we're going to have a vertical asymptote each time. So I'm going to end up with a vertical asymptote. One will be at x equals negative 1, and the other will be at x equal 6. Now, we have those as vertical asymptotes. What about horizontal asymptotes? Well, it turns out that horizontal asymptotes will occur when our x values are getting larger and larger without bound either in the positive or the negative direction. And we're going to look at two methods for figuring this out. One is going to be noticing in the format where it's not factored yet, we can see that as our x value gets larger and larger without bound, there's a couple of ways of considering what this fraction does. Method one will be to do a little bit of manipulation on that expression. And that is if I take the value that is going to be the greatest power of x that I see-- that's going to be x squared-- and I divide both the numerator and denominator by x squared, which in this case ends up being dividing each term in the numerator and denominator by x squared, we'll see how that affects things. So in other words, I'm going to have x squared divided by x squared minus 4 divided by x squared. My denominator will be x squared divided by x squared minus 5x divided by x squared minus 6 divided by x squared. And that ends up giving me a value of 1 minus over x squared in the numerator. And in the denominator, we have 1 minus 5 over x minus over x squared in the denominator. Now remember, what we're looking at is what happens as our x value gets bigger and bigger without bound. And it really doesn't matter for rational functions whether that's happening in the positive or the negative direction. We'd have the same result. Well, notice that the terms that end up being fractional in this format have a denominator that's getting very large while the numerator is staying constant. When that occurs, we have a format c over x to the nth power. And as x goes to infinity, what we have is that this expression, c over x to the nth power, gets closer and closer to 0. So of course, the value of 1 remains at the value 1. It's not affected by what happens to x So actually instead of saying equals, I'm going to say it goes toward-- and that's going to be 1 minus 0 over minus 0 minus 0, which is going to equal 1. And if I read from the beginning to the end, what it's basically saying is my f of x is getting closer and closer to 1 as my x value gets close-- larger and larger without bound. And that's exactly what a horizontal asymptote does. So we end up with a horizontal asymptote whose equation is y equals 1. Now, the second method is to note that in this format, what we notice is the term that's going to take over-- we want to think about it like that; it's going to take over the numerator-- is going to be the term that has the greatest power of-- in for x. So x squared term is really going to take over everything. Think about it like this. If I have a small amount of money and I subtract $4 from it, it's impacted. But if I have $3 million and I subtract $4 from it, it's got very insignificant effect. So the term that makes the most sense is to look at what's happening to the x that's to the larger power. Same thing with the denominator. The x to the larger power is going to take over once we start getting values of x that are larger and larger without bail. So that leads me to method two. And method two is basically saying that I can look at my f of x in terms of what happens in the numerator and in the denominator with the powers that matter the most. So the numerator is x squared plus some stuff, and the denominator is x squared plus some stuff. And the stuff is really insignificant. So this ends up tending to be closer to x squared over x squared, which equals 1, same result as we had previously. As my x gets larger and larger without bound, my function ends up getting closer and closer to x squared over x squared, which is equal to 1. And our horizontal asymptote is y equals 1. So now, I've transferred over the information that we've already found. That is that we have vertical asymptotes at x equal negative 1 and x equal to 6, and we have a horizontal asymptote at y equals 1. Let's go ahead and put those onto the graph that I've drawn. So we have x equals negative 1. And I'm going to use dash lines to indicate my vertical asymptotes. So play like this is relatively straight. And at x equal to 6-- 1, 2, 3, 4, 5, 6-- we have another one. Stand in front of it so I can try to get it straight. Ehh, curvy. That's all right. You get a not good idea. And then at y equals 1, we have the horizontal asymptote. Now what else can we do? Well, we can certainly find intercepts. So the next thing we're going to look at are the x and y-intercepts for this function. So to find the y-intercept, we know that we're going to find f of 0. So substituting in 0 in the numerator gives us 0 squared minus 4, which is going to be negative 4. In the denominator, 0 squared, 0, minus 5 times is 0, and then minus 6. And so we end up with negative 6, which is going to give us a y-intercept of 2/3. Let's go ahead and put that on our graph also. So here at y equal to 2/3, which is approximately right here, we end up with y-intercept. How about our x-intercepts? To find x-intercepts, we know that we need to take f of x and set it equal to 0. So we have x squared minus 4 divided by x squared minus 5x minus 6 equals 0. Now, for a fraction to equal 0, the numerator has to be 0. And you can only think about this as multiplying both sides of this equation by the denominator. But once you kind of get the feel for it, you know fractions are undefined when their denominator is a 0, and they're equal to 0 when their numerators are and, of course, their denominators are not. So we've already determined that this is not going to be reducible, so I can multiply both sides by that denominator and end up with x squared minus 4 equals 0. We can either factor it, or we can simply say x squared equals 4. And now, take square root of both sides of the equation. x equals plus or minus the square root of 4, and that means plus or minus 2. So I'm going to now place those values onto my graph. So we have x equal to negative 2 as one of my x-intercepts and x equal to positive 2 as another one of the x-intercepts. Now, that's not very many points to go with. So do I have to plot a whole lot more points in order to figure out what's going on? Well, there's one other thing I do need to consider, and then, believe it or not, we're going to just connect the dots and have a go at it. The other thing I need to consider is whether or not my graph could ever cross through that horizontal asymptote. Now here's the thing. We know that the graph as x gets larger and larger without bound is going to approach that horizontal line. But for values that are small for x, like close to 0-ish small, it's possible that it could cross a horizontal asymptote. It cannot cross vertical ones, because recall that the vertical ones occur when the denominator is equal to 0. And that means it's undefined. It's not part of the domain. But it is possible for a graph to go through the horizontal asymptote. How do you determine that? Well, if it goes through the horizontal asymptote, remember that that horizontal asymptote has the equation y equals 1. So that would mean that f of x would have to equal 1. So the question is, does f of x cross y equals 1? We can figure that out by solving 1 equals x squared minus 4 divided by x squared minus 5x minus 6. We'll multiply both sides of that equation by the denominator. That gives us x squared minus 5x minus 6 equals the numerator, x squared minus 4. Well, notice that the x squared is going to subtract out, so we end up with negative 5x minus equals negative 4. We'll add 6 to both sides of the equation. Negative 5x equals negative 4 plus 6 is 2. And then, divide both sides of the equation by negative to give us x equals negative 2/5. Now, let's recall what that means. It means that when x is equal to negative 2/5-- that is a point-- our y value is equal to 1. This is a point on the graph, Negative 2/5, 1. So negative 2/5, 1 gives us another point right here, which ends up being right on that horizontal asymptote. At this point, we're kind of going to use a process of elimination. There are a couple of things that you got to recall. Remember that rational functions are going to be similar to polynomial functions. And they're similar to polynomial functions in the following manner. They're going to be smooth and continuous at every value in their domain. Now, what are the values that are not in the domain? Well, we have the vertical asymptote, which is occurring at x equals negative 1. We have another vertical asymptote occurring at x equal to 6. And those were the same values that we took out of the domain of this function. So we have three distinct regions. We have this region over here to the left of the vertical asymptote at x equal to negative 1. We have the region between the two vertical asymptotes. And we have the region over here to the right. Again, we're going to kind of use a process of elimination and logic in order to complete this graph. We know that the graph needs to get close to the vertical asymptote and close to the horizontal asymptote. So if I just consider this portion right here, it has to go through this point. From this point, it has to get close to the vertical asymptote. It could either do it by moving upward or by moving downward. But you know if it went upward, I would have found another value where the graph would intersect the horizontal asymptote. It did not do that. So in order to get close to this vertical asymptote, we have to go downward. It's the only way it can happen. It's got to get close to the horizontal asymptote. It's got to be doing it as I'm heading off toward negative infinity, and it does not cross through the horizontal asymptote to do so, so it has to come up like this. So here's a nice, smooth curve, smooth and continuous on that piece of the domain. Let's now move to the middle section. We have three points that we've already found. I'm going to go ahead and connect the dots right here, so you can kind of see the beginning of it. At this point, the graph either has to approach the vertical asymptote going up or by going back down. But if it went back down, I'd have to have another x-intercept, which I don't have. So again, process of elimination-- it cannot be going down. It has to be going up, so approaching that vertical asymptote by moving upward. Here, I've got to get close to this other vertical asymptote. I could either go up to get close to it, or I could go down. But if I went up, I'd have to go through the horizontal asymptote, which did not occur at any other value besides that negative 2/5. So it has to do it by going down. And finally, let's look at the last little section here. We have no x-intercept. We have no other values where it ends up hitting the horizontal asymptote. If it were down here in this portion, it would have to get-- come from here, go up, and go through the x-axis. It didn't happen. It's got to be up here, and it's got to go toward the asymptote without crossing it. And so here's our picture. And even without calculus, that is a pretty good demonstration of what we can do using just algebra and logic in order to come up with a graph. We're going to look at a couple more examples, and we're going to concentrate on what happens as x heads off toward infinity. Now, we've seen in the previous example that we end up with horizontal asymptote when that occurs. But let's look at these two examples and notice the difference between them. In the first one, we have a function, which is given as f of x equals 6x cubed plus 5x divided by 4x cubed minus 2x squared plus 17x minus 100. Now, this is similar to the last example we just finished with. And in it, we noted that the term that has the greatest exponent on it is going to take over. It's going to have the most impact on that fraction. And so what we can say here is as x goes to infinity, my f of x value is going to approach the fraction that has in its numerator 6x cubed and in its denominator 4x cubed. So I'm taking the term to the greatest exponent power in the numerator and a term to the greatest exponent power in the denominator. And notice that the x cubed over x cubed is going to divide out, and so what I end up with is 6/4. And what that says is that as x goes to infinity, my y value is heading towards 6/4. So y equals 6/4, which can be reduced to 3/2, ends up being a horizontal asymptote. And I'll go ahead and write it out in all of its glory. In the second example, we notice that we have g of x equals 5x minus 4 divided by x squared plus 2x minus 3. Now, the greatest power that we see in the denominator is x to the 2nd power. But notice we don't have an x to the 2nd power in the numerator. Let's see what happens there by using that other technique that we saw earlier. That is, let's divide everything here by the greatest power of x that we see, and then we'll worry about what happens as x goes to infinity. So I'm going to rewrite my g of x as 5x divided by x to the second power minus 4 divided by x to the second power. In the denominator, we'll have x squared divided by x squared plus 2x divided by x squared minus 3 divided by x squared. Now of course, we're able to do this because we're doing the same technique to the numerator as we are to the denominator. It's as though we're multiplying by 1 over x squared divided by 1 over x squared. And it's like multiplying by 1. Let's simplify that. So my g of x then equals-- in my numerator, we end up with 5 over x minus over x squared. In our denominator, we end up with 1 plus-- middle term becomes 2 over x. Last term cannot be simplified, so that's minus 3 over x squared. Remember, what we're interested in is what happens to g of x as x gets larger and larger without bound heading toward infinity. So our g of x is going to get closer to-- well, in the numerator, 5 divided by x gets closer and closer to 0 as x gets larger and larger. So we have 0 minus-- 4 over x squared also goes to 0. Denominator-- 1 is not affected by what x is doing, because it is constant. So we end up with a 1. The other two terms that are, again, both fractional with a denominator that is getting larger and larger without bound go toward 0. So what we see is that g of x goes toward the value of 0. Now, if we back up a little bit, let's just generalize some of this. In my first example, notice that we had the same degree in the numerator as we had in the denominator. They were both degree 3. We could then think of this is heading toward those terms that are of the largest degree. And notice, therefore, we end up with a value, which is going to be the coefficient of the largest term in the numerator divided by the coefficient of the power to the greatest power, which in the denominator is 4x cubed. So we end up with y equals 3/2. In our second example, we have the degree of the numerator, which is a degree smaller than the degree of the denominator. And notice that that numerator heads off towards 0, whereas the denominator heads off toward whatever the coefficient would have been on the largest degree power. So when my degree of my numerator is smaller than the degree of the denominator, it is always going to be the case that we end up with a horizontal asymptote, and that asymptote is going to end up at y equals 0. When those degrees are the same, it turns out that this is going to end up being the leading coefficient divided by the leading coefficient, which in this case I'll reduce to give us 3/2. Now, there's one other case that I want to consider, and that's going to be when we don't end up having the degrees the same or the degrees where the numerator is smaller. We'll look at that next. So now, we're going to look at the function h of x equals x squared minus 3x minus 4 divided by x plus 2. We can see that the degree of the numerator is 2. The degree of the denominator is 1. Now, the first thing I'm going to do before I go any further as far as trying to determine what happens as x gets larger and larger without bound is going to be to make sure that this particular fraction does not reduce any further. That is, let's make sure we don't get in common factors. So in the numerator, I notice that I can factor this into a product of two binomials. We have x and x and then factors of that are going to subtract to give me 3 are going to be 4 and 1. And if I put a minus sign in front of the 4 and a plus in front of the 1, we end up with the correct factorization of the numerator. The denominator is simply x plus 2. So we can see right off the bat that we do not have any common factors. Now remember, what we're interested in is what happens to h of x as x heads off into infinity. What we've done previously is we divided everything by the greatest power of x that we see. I don't know if you can see this right off the bat, but you can see that the numerator is going to head off toward 1, but the denominator, we're not going to end up having anything that's not fractional when I divide by x squared. So I'm going to end up with 1 over something like 0, and that's a value that we don't understand. It's undefined for us. So we're going to have to do a little bit of manipulation to start with. This is really called an improper fraction, because the numerator has a higher degree than the denominator does. And so one thing I can do in this case is I can divide, and I'm going to use long division and show you how that works in a moment. So we're going to take our numerator, which is x squared minus 3x minus 4. And we're going to think about long division where we're going to divide by x plus 2. Now in division of polynomials, let's review that in case you haven't seen it before. We're going to take the leading term in the divisor, which is x, and divide it into the leading term of the dividend, which is x squared. So x divides into x squared x times. And so I can write the value x in my quotient, which is going to be up here. And different textbooks do in different places. I like to put it in the same column with the x's. So x now has to be multiplied times our divisor. So x times x is going to give us x squared. x times 2 is going to give us 2x. Now, thinking about what we do with the numbers, once we get this expression, we then subtract. In this case, it's change the signs and add. So the x squared minus x squared is 0. Minus 3x minus 2x is going to give us minus 5x. And I'm going to bring down the minus 4. And now we repeat the process. How many times does x divide into the first term here, negative 5x? That's going to be a negative 5 times. Multiplying through, we get negative 5 times x to give us negative 5x. We have negative 5 times 2, which is negative 10. We changed the signs and add to give us a value of 6. What that means is I can rewrite my function h of x as follows. h of x is going to equal our quotient, which is x minus 5, plus our remainder 6 over the divisor x plus 2. Now, I don't know when the last time was that you did long division of numbers, but if you mimic what we just did with these two polynomials in division, you'll see that it's the same process as what you do with numbers. What it tells us is that as x is going to infinity, my h of x is getting closer to the expression x minus plus this expression. Now, this expression has an x in the denominator. So in the denominator, if x is going toward infinity, that means the entire fraction is going to 0. So anything that remains like this ends up heading towards 0, which means that we end up with the equation y equals this quotient, x minus 5, as an asymptote. Our value of h of x is getting closer and closer to this line as x gets bigger and bigger without bound in positive and negative direction. Now, this is not going to end up being a vertical line. It's not going to be a horizontal line. Instead, it's a slanted line. And sometimes, it's called a slant asymptote, and other times, you'll hear the term an oblique asymptote. So in either case, you're looking at an equation for a line that is going to be slanted, and this graph is going to get closer and closer to that slanted asymptote as x gets bigger and bigger without bound. We're going to look at one more example before I let you try one on your own. There's one other technique that I need to point out that we haven't come across yet. And that is that we're going to look at this expression, which is y equals-- it's a function-- 2x squared minus 2 over x squared minus 3x plus 2. Remember that we start off by trying to look at its factored form, and then we'll look at what we can determine from there. So in my numerator, I notice that I can factor out a 2. That's going to give me 2 times x squared minus 1. I'm going to go ahead and take this one more step. Notice that this is 2 times x minus 1 times x plus 1, because that's the difference of two squares. My denominator, we can simply factor. We're going to have x and an x, and we need a 2 and a 1. And both are negative. So we end up with x minus 2 times x minus 1. Now, in order to find the domain, it's important that you don't do any kind of reducing yet. So I'm going to come back to this in a moment. But to find the domain of the function, recall that we look at our denominator, and we make sure that our denominator does not equal 0. And then, we take any other values that we end up with. Well, our denominator will equal when we end up having x equal to 2 or x equal to 1. So I'm going to eliminate those two values from domain. That is, we're going to go from negative infinity to 1, pick up at 1, go all the way to 2, pick up at 2, and go to infinity. So again, we have our domain. But now, this is where this problem is a little different than what we'd seen earlier. We notice that x minus 1 is a common factor to both the numerator and the denominator. You may say, oh, good. Let's just cancel it out. Well, yes, I am going to do that. But when you're canceling it out, you're really using division. You're dividing both numerator and denominator by the same value. And when you divide, you have to make sure you're not dividing by 0. So when I do this little slashing right here and I rewrite this as-- I'm going to go ahead and multiply out 2x plus over x minus 2-- what we're really saying is in-between this step, this is true as long as x minus 1 doesn't equal or, in other words, as long as x does not equal 1. Let's take it from the beginning to the end. We start off with a function which is equal to this. We come up with it equal to this, and that's going to be true as long as x doesn't equal 1. Or in other words, the graphs of this function and this function will coincide at all values except for x equal to 1. What that means is we can now concentrate on graphing this function, and then we'll come back and take care of that point that has to be taken out. It's going to end up being called a hole in the graph. So I'm going to concentrate now on y equals 2x plus 2 divided by x minus 2. And using the techniques that we just came up with, we know that now it's reduced. We're going to have a vertical asymptote at all values that make the denominator 0. So we'll have a vertical asymptote at x equals 2. Notice the degree of the numerator is 1. The degree of the denominator is 1. So therefore, we're going to end up having a horizontal asymptote where my y value is getting closer and closer to 2x divided by x, which means our y value equal to 2 gives us a horizontal asymptote. We could find our intercepts pretty easily. To find our y-intercept, we're going to be evaluating f of 0. But I didn't call it f of x, but it's the same thing. So f of 0 means substitutes in 0 for x. So I am going to end up with 2 over negative 2, which equals negative 1. To find my x-intercepts, we can look at where this fraction equals 0, which would be where the numerator is 0. So 2x plus 2 equals 0 when x is equal to negative 1. And so we end up having these values that we can now place on a graph. And again, I'm going to just put them all together. So quickly sketching in an xy plane. What do we have far? For we have a vertical asymptote at x equals 2. We have a horizontal asymptote at y equals 2. We have a y-intercept at negative 1. We have an x-intercept at negative 1. By process of elimination, we see that the graph is going to be below here and then probably above here. That's actually one other thing we should consider is, does this function ever end up crossing that horizontal asymptote? That is-- and remember, I'm dealing with this one-- does 2x plus 2 divided by x minus ever equal the value of the horizontal asymptote, which is 2? So does 2x plus 2 ever equal-- and I'm going to now multiply both sides of this by x minus 2-- does 2x plus 2 ever equal 2x minus 4? Well, that would require 2 to equal negative 4, which, of course, does not happen. So what that means is that our graph will not cross the horizontal asymptote. So when I connect the dots down here, these two points have to be connected. It has to get up closer and closer to the vertical asymptote. If it went upward, it would have to cross the horizontal asymptote, which it does not. It has to get closer and closer over here. We don't have any x-intercept over here, and so we know that we can't have that occurring. And it has to basically approach both of these asymptotes. There's one other thing to worry about. What's this part? What happens when x is equal to the value of 1? And that means down here-- well, let's go ahead and put this part in, and then we'll worry about it. It has to go like this, approach those asymptotes. When x equals 1, we have a point on that graph about right here. Now, we can determine what the value is by substituting right there. So we're going to plug in a value of 1. 2 time 1 plus 2 over 1 minus 2. That's going to give us 2 plus 2, which is going to equal 4 divided by negative 1, which equals negative 4. So down here, about right there, we have a point right here which I'm actually going to take out of my graph. So it's as though I'm erasing it, and there's a gap right there. That's hard to see, and plus, the gap is extremely small. So what we do to indicate that that point is missing is we draw an open circle right there. So what we end up with is a graph that resembles the graph once it's in reduced format, except that factor that ended up being canceled out has to now give us a point' which is missing in our original function, because it's not part of our domain. So whenever we cancel out a common factor, we're going to end up having a hole in our graph wherever that factor would be equal to 0. And this is now the result of our problem. That's our graph. So now, let's look at some generalizations we can make when we're looking at graphing rational functions. For rational function of the form y equals f of x divided by g of x, the domain is going to be the set of all x values such that g of x does not equal 0. That is, our denominator can but not be 0. The graph of a rational function is smooth and continuous on its domain. We'll begin by reducing the fraction as much as possible. If any common factors are present, there will be a hole in the graph for the x-value where that factor equals 0. We'll next look at finding all intercepts. There will be a vertical asymptote for every value that makes the denominator equal to in the reduced format. We're going to check for horizontal or oblique, also called slant, asymptotes by considering what happens to the functional value as x approaches infinity. If the degree of the numerator is less than the degree of the denominator, the graph will have a horizontal asymptote at y equals 0. If the degree of the numerator is equal to the degree of the denominator, the graph will have a horizontal asymptote y equals a sub n divided by b sub n, where a sub n is the leading coefficient of the numerator and b sub n is the leading coefficient of the denominator. If the degree of the numerator is greater than the degree of the denominator, the graph will have an oblique asymptote to be determined by the quotient upon division of the numerator by the denominator. Its time for a quick quiz. Name the vertical and horizontal asymptotes for the graph of the function given by f of x equals in the numerator we have x multiply times second factor which is ax minus b, in the denominator we have two factors, the first is x minus c and the other one is x minus d. Is the answer A? x equals c comma, x equals d comma y equals a. or is the answer B? x equals b divided by a, x equals c, x equals d and y equals 0. or is the answer C? y equals 0, y equals a, x equals c and x equals d. Use some time in order to figure out what you think the asymptotes are and then choose from A, B, or C. You're correct, the answer is A. We have 3 asymptotes for this function they are x equals c, x equals d. and y equals a. Sorry the correct answer is A, there are 3 asymptotes for this function. The equations are x equals c, x equals d, and y equals a. In trying to find the asymptotes for the function we first note that the function is in reduced form, there were no common factors between the numerator and the denominator. When that is the case we can locate our vertical asymptotes by taking the denominator and setting it equal to 0. So I'm going to have x minus c times x minus d equals 0. So already in factored form so I can set each factor equal to to give us results of x equals c and x equals d. So I have 2 vertical asymptotes x equal c and x equals d. Well, noticed that those were part of all of the different answers. So one thing you will note is that in b, we had an extra vertical asymptotes which was x equals b over a and so this is not going to be correct. We're not going to take something from the numerator and said it equals 0. Let's now consider where we find horizontal asymptotes to consider that I'm going to do a process here where I'm going to multiply out. So I want to consider what's going to happen in my numerator if I multiply those terms together I'm going to have ax squared minus be times x. In the denominator when I multiply out I'm going to end up with x squared minus dx minus cx plus cd. Now what's important here is to consider what's going on with the leading terms in our numerator and our denominator. Ask why x value gets larger and larger and larger without bound which is what we're considering we were talking a horizontal asymptotes. The terms that are going to take over if you'd like or going to be the terms that have the greatest power on my variable. So another words when I'm looking for horizontal asymptotes I want to consider what happens as x is heading off toward infinity. And its x heads off toward infinity my value of f of x is getting closer and closer to the value of ax squared divided by x squared in other words the terms that don't have the greatest power on x such as the minus bx and the minus dx minus cx plus cd they become less influential. Well if I look at this and I take ax squared divide by x squared that's equal to the value of a, so as x is heading toward infinity the y value is getting closer and closer to the value of a, which means that y equals a is going to end up as a horizontal asymptotes. Notice that, that's going to occur in the answer that was given in A. Note also occurred in C, but notice we had an extra horizontal asymptote of y equals 0 in both B and C. So both of those answers are incorrect and the correct answer was A, they're only 3 asymptotes for this function. So finally, we're at the point where you can try one of these problems on your own. So here's the function we want to look at. f of x equals 4x squared minus 4x plus 1 in the numerator divided by 2x squared minus x minus in the denominator. We want to find the domain, identify all asymptotes, find intercepts, and then finally, sketch the graph. Pause the recording right now, and try this on your own. And then, pick back up in a few minutes, and we'll see how you did. So now, let's look at the problem together. We start with the domain. Recall that means that we need to take the denominator of this fraction and set it equal to and then eliminate any of those values from our domain. So therefore, we have 2x squared minus x minus 3. Let's set that equal to 0 and factor. And check your factorization to see if you did it as I'm doing it. We end up with x equals 3/2 and x equals negative 1. Those will be our values that we need to eliminate from the domain. So we'll have our domain from negative infinity to negative 1, picking up at negative 1 and going to 3/2, and then picking up at 3/2 and going to infinity. Do we have any asymptotes? Well, we need to first look at the factorization of this numerator and denominator to see if we have any common factors. So in the numerator, we can look at factoring that. Let's see-- we need factors of 4x squared minus 4x plus 1. So we start off with factors of 4x squared. And probably, we're going to need to use 2x and 2x and then factors of 1, which would be 1 and 1. And in order to end up with a negative middle term, we need to have both of these negative. So we can see that the numerator has the double factor of 2x minus 1. Our denominator we'd already factored a few minutes ago. It was 2x minus 3 times x plus 1. So if we want to rewrite this, we can rewrite it as 2x minus 1 quantity squared over 2x minus 3 times x plus 1. Now, notice, therefore, we do not have any common factors. So we will not end up with a hole in the graph. If we're looking at now finding asymptotes, we can take each of the values from the denominator, each factor, set it equal to 0, and we'll have a vertical asymptote for each of those values. So those are the same values we came up with that we had to eliminate from our domain. So our asymptotes are going to have equation x equals negative 1, x equals 3/2. To find our oblique, or slant, asymptote, we look at the degree of the numerator and compare it to the degree of the denominator. Remember, we're interested in what happens as x goes to infinity. The degree of the numerator is 2. The degree of the denominator is 2, which means that as x goes to infinity, our y-value, our f of x value, is getting closer to this leading term, 4x squared over 2x squared. Or in other words, we're going to have an asymptote which equals the reduction of that 2, and our y-value would equal 2. So we're going have a horizontal asymptote that y equals 2. To find our intercepts, we can find our y-intercept by finding the value of f of 0. Now, the easiest place to substitute that in would be in its nonfactor form. Plugging in 0 into the numerator, we end up with a value of 1. In the denominator, substituting in gives us a value of negative 3. So we end up with our y-intercept at 0, negative 1/3. For x-intercepts, we can take our numerator and set it equal to 0 because of the fact that we know that the fraction is equal to when its numerator is equal to 0. And we have this already in its factor format, and it was not reducible. So that means our numerator is equal to when 2x minus 1 squared equals 0 or, in other words, when x is equal to 1/2. So we're going to have an intercept at 1/2, 0. Now, we're ready to put all of this together in order to come up with a graph. So we're going to take what we have so far and put it onto a graph and then see what else we need to do. So to begin with, let's just quickly sketch out an xy plane, and let's see what we can put on there. We have an asymptote at x equals negative 1. That's a vertical asymptote. We have another vertical asymptote at x equals 3/2. So it's this 1 and that's 2, then 3/2 is about right there. We have a horizontal asymptote at y equals 2. We have an intercept at 0, negative 1/3-- big ol' dot. We have another x-intercept at 1/2, 0, right here. Now, there's something we haven't determined yet, and that's whether or not our graph can actually cross the horizontal asymptote. Recall that to do that, we have to take our function and set it equal to that value of y. So I'm going to go with this top format, and we're going to have the 4x squared minus 4x plus 1 divided by 2x squared minus x minus 3. Let's set that equal to 2. We'll multiply through by the denominator, 4x squared minus 4x plus 1 would have to equal 2 times this denominator, which is going to give us 4x squared minus 2x minus 6. Notice that the 4x squared is going to subtract out. I'm then going to end up with negative 4x plus 1 equals negative 2x minus 6. Let's go ahead and add 2x to both sides of the equation. So we'll have negative 2x on the left. We'll go ahead and subtract 1 from both sides of the equation, so we'll end up with negative 7. And finally, we can divide both sides of the equation by negative 2 to end up with x equals 7/2. What that tells us is that if I go over to 3.5, we're going to end up with a value that is going to be right here at 3.5, 2. Now, we've got to figure out what's going on with this graph from just this information, so again, kind of a process of elimination. We're going to look first at the section that is over here to the left of our vertical asymptote at x equal to negative 1. Notice that we do not have an x-intercept. The graph cannot cross through the horizontal asymptote. It's got to be above that horizontal asymptote heading up toward the vertical asymptote. So we're going to have some sort of a picture. And again, I'm not really worried about exact values. And then, I'm not going to plot any more points. I just want a general shape. There we go. Got that one section done. In the middle section, we notice that we have these two points that certainly can be connected. It does not end up crossing the horizontal asymptote at all. And I know that I'm going to have to head down to this vertical asymptote. Well, to get close to this other vertical asymptote, I also have to head down, and I'm going to go ahead and do that like that. Now, let's just make a connection between polynomial functions and rational functions. When we look at this x-intercept, notice that it came from the factor 2x minus 1 squared. We can think of that as a factor of multiplicity 2. So therefore, notice it's bouncing off the graph right there. So it's in keeping with what we learned earlier. And finally, we have this section over here to the right. Now, we only have a single point. We have that it's no x-intercept. So if it were coming down toward that asymptote, it would have to have an x-intercept. It must be going up toward that vertical asymptote. And then, what in the world is happening here? Well, it's got to get close to this horizontal asymptote. But notice it's got this point where it crosses through. So this is what looks like it goes down, and then it comes right back up toward it. It's kind of a cool shape right there. And that is going to be the picture of your graph. Now, don't worry about it if you didn't get everything correct here, because we added in a couple of little things that were a little bit different than what you'd seen earlier. But if you had the major ideas, then you're on the right track. It's time now for you to try some problems more than this one on your own and see how you do. Good luck.
Table of contents
- 0. Review of Algebra4h 16m
- 1. Equations & Inequalities3h 18m
- 2. Graphs of Equations43m
- 3. Functions2h 17m
- 4. Polynomial Functions1h 44m
- 5. Rational Functions1h 23m
- 6. Exponential & Logarithmic Functions2h 28m
- 7. Systems of Equations & Matrices4h 6m
- 8. Conic Sections2h 23m
- 9. Sequences, Series, & Induction1h 19m
- 10. Combinatorics & Probability1h 45m
5. Rational Functions
Introduction to Rational Functions
Video duration:
53sPlay a video: