Table of contents
- 0. Review of Algebra4h 16m
- 1. Equations & Inequalities3h 18m
- 2. Graphs of Equations43m
- 3. Functions2h 17m
- 4. Polynomial Functions1h 44m
- 5. Rational Functions1h 23m
- 6. Exponential & Logarithmic Functions2h 28m
- 7. Systems of Equations & Matrices4h 6m
- 8. Conic Sections2h 23m
- 9. Sequences, Series, & Induction1h 19m
- 10. Combinatorics & Probability1h 45m
7. Systems of Equations & Matrices
Determinants and Cramer's Rule
Problem 41a
Textbook Question
In Exercises 37–44, use Cramer's Rule to solve each system.
x + y + z = 4
x - 2y + z = 7
x + 3y + 2z = 4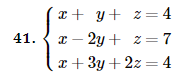
 Verified step by step guidance
Verified step by step guidance1
Identify the coefficient matrix A from the system of equations: A = \begin{bmatrix} 1 & 1 & 1 \\ 1 & -2 & 1 \\ 1 & 3 & 2 \end{bmatrix}.
Calculate the determinant of matrix A, denoted as det(A).
Form the matrix A_x by replacing the first column of A with the constants from the right side of the equations: A_x = \begin{bmatrix} 4 & 1 & 1 \\ 7 & -2 & 1 \\ 4 & 3 & 2 \end{bmatrix}.
Calculate the determinant of matrix A_x, denoted as det(A_x).
Use Cramer's Rule to find x: x = \frac{\text{det}(A_x)}{\text{det}(A)}.
Recommended similar problem, with video answer:
 Verified Solution
Verified SolutionThis video solution was recommended by our tutors as helpful for the problem above
Video duration:
8mPlay a video:
Was this helpful?

 4:36m
4:36mWatch next
Master Determinants of 2×2 Matrices with a bite sized video explanation from Patrick Ford
Start learningRelated Videos
Related Practice