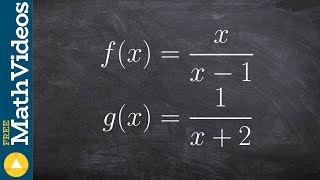The functions in Exercises 11-28 are all one-to-one. For each function, a. Find an equation for f^-1(x), the inverse function. b. Verify that your equation is correct by showing that f(ƒ^-1 (x)) = = x and ƒ^-1 (f(x)) = x. f(x) = (2x +1)/(x-3)

Verified step by step guidance

1. <Step 1: To find the inverse of the function \( f(x) = \frac{2x + 1}{x - 3} \), start by replacing \( f(x) \) with \( y \). So, \( y = \frac{2x + 1}{x - 3} \).>
2. <Step 2: Swap \( x \) and \( y \) to find the inverse. This gives \( x = \frac{2y + 1}{y - 3} \).>
3. <Step 3: Solve for \( y \) in terms of \( x \). Multiply both sides by \( y - 3 \) to get \( x(y - 3) = 2y + 1 \).>
4. <Step 4: Distribute \( x \) on the left side: \( xy - 3x = 2y + 1 \). Rearrange the equation to get all terms involving \( y \) on one side: \( xy - 2y = 3x + 1 \).>
5. <Step 5: Factor out \( y \) from the left side: \( y(x - 2) = 3x + 1 \). Finally, solve for \( y \) by dividing both sides by \( x - 2 \): \( y = \frac{3x + 1}{x - 2} \). This is \( f^{-1}(x) \).>

Key Concepts

One-to-One Functions

A one-to-one function is a type of function where each output is produced by exactly one input. This means that if f(a) = f(b), then a must equal b. One-to-one functions have unique inverses, which is crucial for finding the inverse function f^-1(x). Understanding this property ensures that we can correctly derive and verify the inverse.

Inverse Functions

An inverse function, denoted as f^-1(x), reverses the effect of the original function f(x). To find the inverse, we typically swap the roles of x and y in the equation and solve for y. The existence of an inverse is contingent upon the function being one-to-one, allowing us to express the relationship in a way that undoes the original function's operations.

Verification of Inverse Functions

To verify that two functions are inverses, we must show that f(f^-1(x)) = x and f^-1(f(x)) = x. This means that applying the original function to its inverse returns the input value, confirming their relationship. This verification process is essential to ensure that the derived inverse function is correct and accurately represents the original function's behavior.