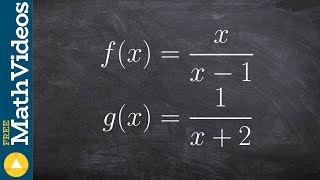Table of contents
- 0. Review of Algebra4h 16m
- 1. Equations & Inequalities3h 18m
- 2. Graphs of Equations43m
- 3. Functions2h 17m
- 4. Polynomial Functions1h 44m
- 5. Rational Functions1h 23m
- 6. Exponential & Logarithmic Functions2h 28m
- 7. Systems of Equations & Matrices4h 6m
- 8. Conic Sections2h 23m
- 9. Sequences, Series, & Induction1h 19m
- 10. Combinatorics & Probability1h 45m
3. Functions
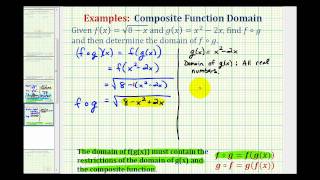
Function Composition
Problem 20
Textbook Question
Determine whether each function graphed or defined is one-to-one. y = -√100 - x^2
 Verified step by step guidance
Verified step by step guidance1
Understand that a function is one-to-one if each output value is paired with exactly one input value, meaning it passes the horizontal line test.
Consider the given function: \( y = -\sqrt{100 - x^2} \). This is a transformation of the equation of a circle \( x^2 + y^2 = 100 \).
Recognize that the function \( y = -\sqrt{100 - x^2} \) represents the lower half of a circle centered at the origin with a radius of 10.
Visualize or sketch the graph of the function. The graph is a semicircle below the x-axis, spanning from \( x = -10 \) to \( x = 10 \).
Apply the horizontal line test: If any horizontal line intersects the graph more than once, the function is not one-to-one. In this case, any horizontal line below the x-axis will intersect the semicircle at two points, indicating the function is not one-to-one.
Recommended similar problem, with video answer:
 Verified Solution
Verified SolutionThis video solution was recommended by our tutors as helpful for the problem above
Video duration:
2mPlay a video:
Was this helpful?
Key Concepts
Here are the essential concepts you must grasp in order to answer the question correctly.
One-to-One Functions
A function is considered one-to-one if it assigns a unique output for every unique input, meaning no two different inputs produce the same output. This can be verified using the horizontal line test: if any horizontal line intersects the graph of the function more than once, the function is not one-to-one.
Recommended video:

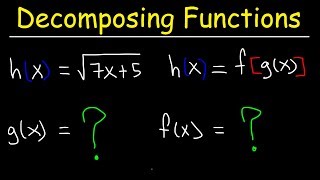
Decomposition of Functions
Graphing Functions
Graphing a function involves plotting its output values against its input values on a coordinate plane. Understanding how to interpret the shape and behavior of the graph is crucial for determining properties like whether the function is one-to-one. The graph of a function can reveal important characteristics such as symmetry and intervals of increase or decrease.
Recommended video:

Graphs of Logarithmic Functions
Square Root and Quadratic Functions
The function given, y = -√(100 - x^2), combines a square root and a quadratic expression. The square root function typically produces non-negative outputs, while the negative sign inverts these values. This affects the overall behavior of the function, particularly its range and whether it can be one-to-one, as quadratic functions are generally not one-to-one due to their parabolic shape.
Recommended video:

Solving Quadratic Equations by the Square Root Property

 4:56m
4:56mWatch next
Master Function Composition with a bite sized video explanation from Nick Kaneko
Start learningRelated Videos
Related Practice