Table of contents
- 0. Review of Algebra4h 16m
- 1. Equations & Inequalities3h 18m
- 2. Graphs of Equations43m
- 3. Functions2h 17m
- 4. Polynomial Functions1h 44m
- 5. Rational Functions1h 23m
- 6. Exponential & Logarithmic Functions2h 28m
- 7. Systems of Equations & Matrices4h 6m
- 8. Conic Sections2h 23m
- 9. Sequences, Series, & Induction1h 19m
- 10. Combinatorics & Probability1h 45m
1. Equations & Inequalities
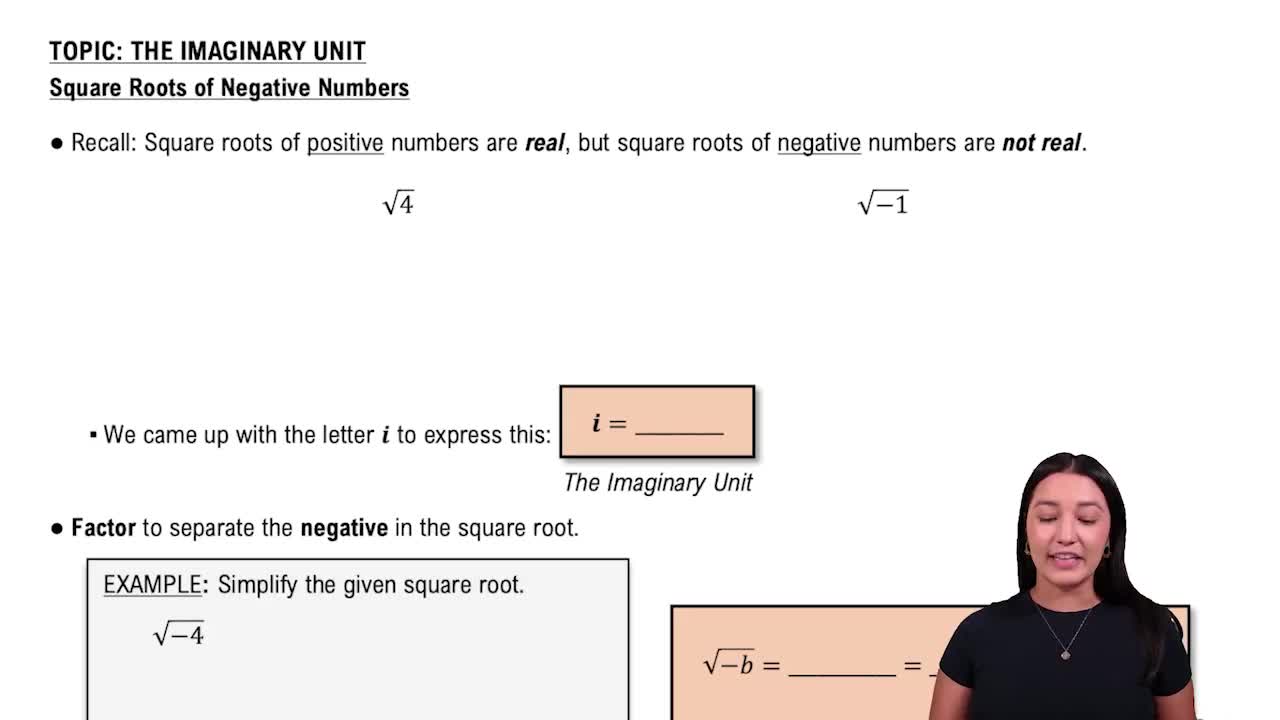
The Imaginary Unit
Problem 53
Textbook Question
In Exercises 53–60, write each power of i as as i, - 1, - i, or 1. i^31
 Verified step by step guidance
Verified step by step guidance1
Recognize that the powers of \(i\) cycle every four: \(i^1 = i\), \(i^2 = -1\), \(i^3 = -i\), \(i^4 = 1\).
To find \(i^{31}\), determine the remainder when 31 is divided by 4, since the powers of \(i\) repeat every 4.
Calculate \(31 \div 4\) to find the quotient and remainder.
The remainder of \(31 \div 4\) is 3, which corresponds to \(i^3\) in the cycle.
Therefore, \(i^{31} = i^3 = -i\).
Recommended similar problem, with video answer:
 Verified Solution
Verified SolutionThis video solution was recommended by our tutors as helpful for the problem above
Video duration:
3mPlay a video:
Was this helpful?
Key Concepts
Here are the essential concepts you must grasp in order to answer the question correctly.
Powers of i
The imaginary unit i is defined as the square root of -1. The powers of i cycle through four distinct values: i^1 = i, i^2 = -1, i^3 = -i, and i^4 = 1. This cyclical pattern repeats every four powers, which is essential for simplifying higher powers of i.
Recommended video:

Powers of i
Modulus and Division
To simplify powers of i, we can use the modulus of the exponent with respect to 4. For example, to find i^31, we calculate 31 mod 4, which gives us a remainder of 3. This means i^31 is equivalent to i^3, allowing us to simplify the expression effectively.
Recommended video:

Higher Powers of i
Complex Numbers
Complex numbers are numbers that have a real part and an imaginary part, expressed in the form a + bi, where a and b are real numbers. Understanding complex numbers is crucial when working with powers of i, as it provides context for their applications in mathematics and engineering.
Recommended video:

Dividing Complex Numbers

 5:02m
5:02mWatch next
Master Square Roots of Negative Numbers with a bite sized video explanation from Callie
Start learning