Here are the essential concepts you must grasp in order to answer the question correctly.
Quadratic Equations
A quadratic equation is a polynomial equation of the form ax^2 + bx + c = 0, where a, b, and c are constants, and a ≠ 0. These equations can represent various real-world scenarios and are characterized by their parabolic graphs. Understanding how to manipulate and solve these equations is essential for applying the quadratic formula.
Recommended video:
Introduction to Quadratic Equations
Quadratic Formula
The quadratic formula is a method for solving quadratic equations, given by x = (-b ± √(b² - 4ac)) / (2a). This formula provides the solutions for x by substituting the coefficients a, b, and c from the quadratic equation. It is particularly useful when factoring is difficult or impossible.
Recommended video:
Solving Quadratic Equations Using The Quadratic Formula
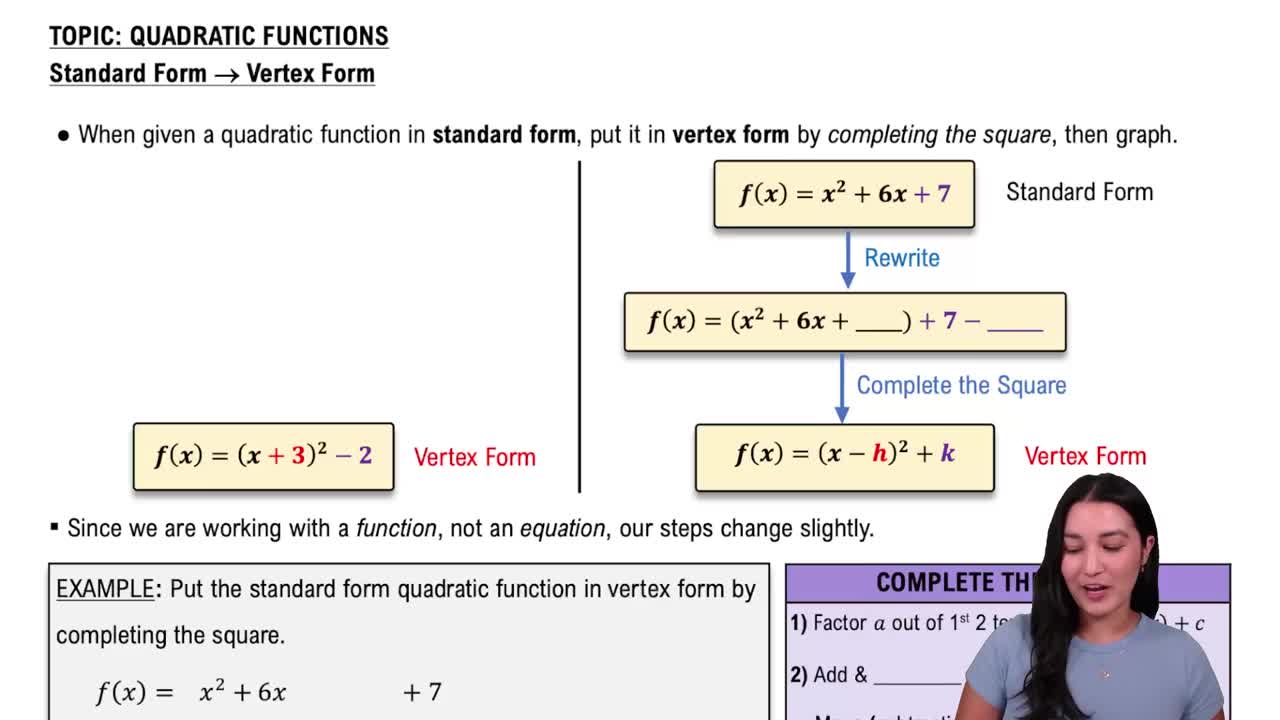
Standard Form of a Quadratic Equation
The standard form of a quadratic equation is expressed as ax^2 + bx + c = 0. To use the quadratic formula effectively, the equation must first be rearranged into this form. This often involves moving all terms to one side of the equation, ensuring that the equation is set equal to zero, which is a crucial step in the solving process.
Recommended video:
Converting Standard Form to Vertex Form
 Verified Solution
Verified Solution


 6:36m
6:36m
