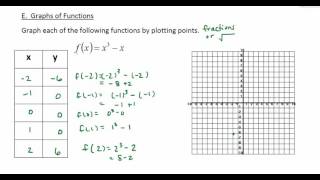
Graph each equation in Exercises 13 - 28. Let x = - 3, - 2, - 1, 0, 1, 2, 3 y = x - 2

Verified step by step guidance

1. Identify the equation given: \( y = x - 2 \). This is a linear equation in the form \( y = mx + b \), where \( m \) is the slope and \( b \) is the y-intercept.
2. Create a table of values for \( x \) using the given values: \(-3, -2, -1, 0, 1, 2, 3\). For each \( x \), calculate the corresponding \( y \) value using the equation \( y = x - 2 \).
3. For each \( x \) value, substitute it into the equation to find \( y \). For example, if \( x = -3 \), then \( y = -3 - 2 \). Repeat this for each \( x \) value.
4. Plot the points \((x, y)\) on a coordinate plane. Each point corresponds to a pair from your table of values.
5. Draw a straight line through the plotted points. This line represents the graph of the equation \( y = x - 2 \).

Key Concepts

Linear Equations

A linear equation is an algebraic expression that represents a straight line when graphed on a coordinate plane. It typically takes the form y = mx + b, where m is the slope and b is the y-intercept. In the given equation y = x - 2, the slope is 1 and the y-intercept is -2, indicating that the line rises one unit for every unit it moves to the right.

Graphing Points

Graphing points involves plotting specific coordinates on a Cartesian plane, where each point is defined by an x-value and a corresponding y-value. For the equation y = x - 2, you can calculate y for each given x value (-3, -2, -1, 0, 1, 2, 3) to find the corresponding points, which can then be plotted to visualize the linear relationship.

Slope-Intercept Form

The slope-intercept form of a linear equation is a way to express the equation of a line as y = mx + b, where m represents the slope and b represents the y-intercept. This form is particularly useful for quickly identifying the slope and y-intercept, allowing for easy graphing. In the equation y = x - 2, the slope is 1, indicating a 45-degree angle, and the line crosses the y-axis at -2.