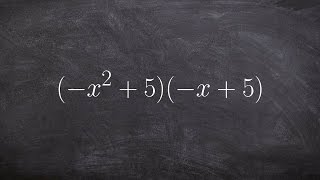Table of contents
- 0. Review of Algebra4h 16m
- 1. Equations & Inequalities3h 18m
- 2. Graphs of Equations43m
- 3. Functions2h 17m
- 4. Polynomial Functions1h 44m
- 5. Rational Functions1h 23m
- 6. Exponential & Logarithmic Functions2h 28m
- 7. Systems of Equations & Matrices4h 6m
- 8. Conic Sections2h 23m
- 9. Sequences, Series, & Induction1h 19m
- 10. Combinatorics & Probability1h 45m
0. Review of Algebra
Multiplying Polynomials
Problem 44c
Textbook Question
In Exercises 35–54, use the FOIL method to multiply the binomials. (2x−3)(4x−5)
 Verified step by step guidance
Verified step by step guidance1
Identify the binomials to be multiplied: \((2x - 3)\) and \((4x - 5)\).
Apply the FOIL method, which stands for First, Outer, Inner, Last, to multiply the terms in the binomials.
First: Multiply the first terms of each binomial: \(2x \times 4x\).
Outer: Multiply the outer terms of the binomials: \(2x \times -5\).
Inner: Multiply the inner terms of the binomials: \(-3 \times 4x\).
Recommended similar problem, with video answer:
 Verified Solution
Verified SolutionThis video solution was recommended by our tutors as helpful for the problem above
Video duration:
6mPlay a video:
Was this helpful?
Key Concepts
Here are the essential concepts you must grasp in order to answer the question correctly.
FOIL Method
The FOIL method is a technique used to multiply two binomials. FOIL stands for First, Outside, Inside, Last, which refers to the order in which you multiply the terms of the binomials. This method helps ensure that all combinations of terms are accounted for, leading to the correct product.
Recommended video:
Binomials
A binomial is a polynomial that consists of exactly two terms, typically separated by a plus or minus sign. In the expression (2x−3)(4x−5), both (2x−3) and (4x−5) are binomials. Understanding the structure of binomials is essential for applying the FOIL method effectively.
Recommended video:
Guided course

Special Products - Cube Formulas
Distributive Property
The distributive property states that a(b + c) = ab + ac, allowing you to distribute a single term across terms within parentheses. This property is fundamental to the FOIL method, as it involves distributing each term in the first binomial to each term in the second binomial, ensuring all products are calculated.
Recommended video:
Guided course
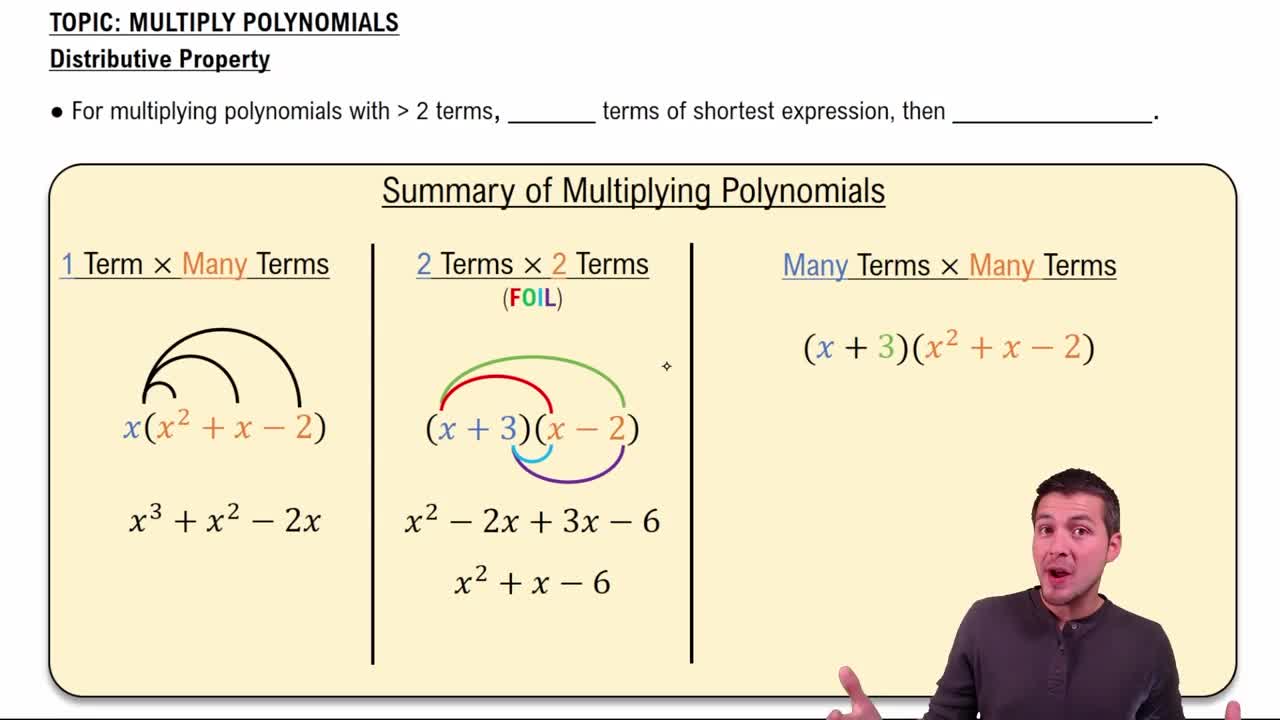
Multiply Polynomials Using the Distributive Property
Related Videos
Related Practice